A toy gun a spring of force constant k. When changed before being triggered in the upward direction, the spring is compressed by a distance x. If the mass of the shot is m, on the being triggered it will go up to a height of
(a) Kx2mg
(b) x2Kmg
(c) Kx22mg
(d) K2x2mg
The correct Answer is:C
To solve the problem, we need to analyze the energy transformations involved when the spring is released and the mass is shot upwards. We will use the principle of conservation of energy.
Step 1: Understand the initial conditions
- The spring is compressed by a distance x.
- The force constant of the spring is k.
- The mass of the shot is m.
- Initially, the kinetic energy of the mass is zero as it is at rest.
Hint: Identify the initial potential energy stored in the spring when it is compressed.
Step 2: Calculate the potential energy stored in the spring
The potential energy (PE) stored in the spring when it is compressed by a distance x is given by the formula:
PE=12kx2
Hint: Remember that the potential energy in a spring is calculated using the spring constant and the compression distance.
Step 3: Analyze the final conditions
When the spring is released, the potential energy converts into gravitational potential energy (GPE) as the mass rises to a height h. At the maximum height, the kinetic energy will be zero again.
The gravitational potential energy at height h is given by:
GPE=mgh
Hint: Think about how energy conservation applies when the spring releases its energy to lift the mass.
Step 4: Apply the conservation of energy principle
According to the conservation of energy, the initial potential energy in the spring will equal the gravitational potential energy at the maximum height:
12kx2=mgh
Hint: Set the potential energy from the spring equal to the gravitational potential energy to find the height.
Step 5: Solve for height h
Rearranging the equation to solve for h:
mgh=12kx2
h=kx22mg
Hint: Isolate h on one side of the equation to express it in terms of the other variables.
Final Answer
The height h to which the mass will rise is given by:
h=kx22mg
Summary of Steps
1. Identify initial conditions (spring compressed, mass at rest).
2. Calculate the potential energy stored in the spring.
3. Analyze final conditions (mass rises to height h).
4. Apply conservation of energy to relate spring potential energy to gravitational potential energy.
5. Solve for height h.
- The spring is compressed by a distance x.
- The force constant of the spring is k.
- The mass of the shot is m.
- Initially, the kinetic energy of the mass is zero as it is at rest.
The potential energy (PE) stored in the spring when it is compressed by a distance x is given by the formula:
PE=12kx2
When the spring is released, the potential energy converts into gravitational potential energy (GPE) as the mass rises to a height h. At the maximum height, the kinetic energy will be zero again.
GPE=mgh
According to the conservation of energy, the initial potential energy in the spring will equal the gravitational potential energy at the maximum height:
12kx2=mgh
Rearranging the equation to solve for h:
mgh=12kx2
h=kx22mg
The height h to which the mass will rise is given by:
h=kx22mg
1. Identify initial conditions (spring compressed, mass at rest).
2. Calculate the potential energy stored in the spring.
3. Analyze final conditions (mass rises to height h).
4. Apply conservation of energy to relate spring potential energy to gravitational potential energy.
5. Solve for height h.
Topper's Solved these Questions
- WORK, POWER & ENERGYBook:CENGAGE PHYSICS ENGLISHChapter:WORK, POWER & ENERGYExercise:Multiple Correct
Explore 25 Videos
- WORK, POWER & ENERGYBook:CENGAGE PHYSICS ENGLISHChapter:WORK, POWER & ENERGYExercise:Linked Comprehension
Explore 55 Videos
- WORK, POWER & ENERGYBook:CENGAGE PHYSICS ENGLISHChapter:WORK, POWER & ENERGYExercise:Subjective
Explore 23 Videos
- VECTORSBook:CENGAGE PHYSICS ENGLISHChapter:VECTORSExercise:Exercise Multiple Correct
Explore 5 Videos
Similar Questions
Two masses m1andm2 re connected by a spring of spring constant k and are placed on as frictionless horizontal surface. Initially the spring is stretched through a distance x0 when the system is released from rest. Find the distance moved by the two masses before they again come to rest.
View SolutionFigure shows a smooth track, a part of which is a circle of radius r. A block of mass m is pushed against a spring of spring constant k fixed at the left end and is then released. Find the initial compression of the spring so that the block presses the track with a force mg when it reaches the point P, where the radius of the track is horizontal.
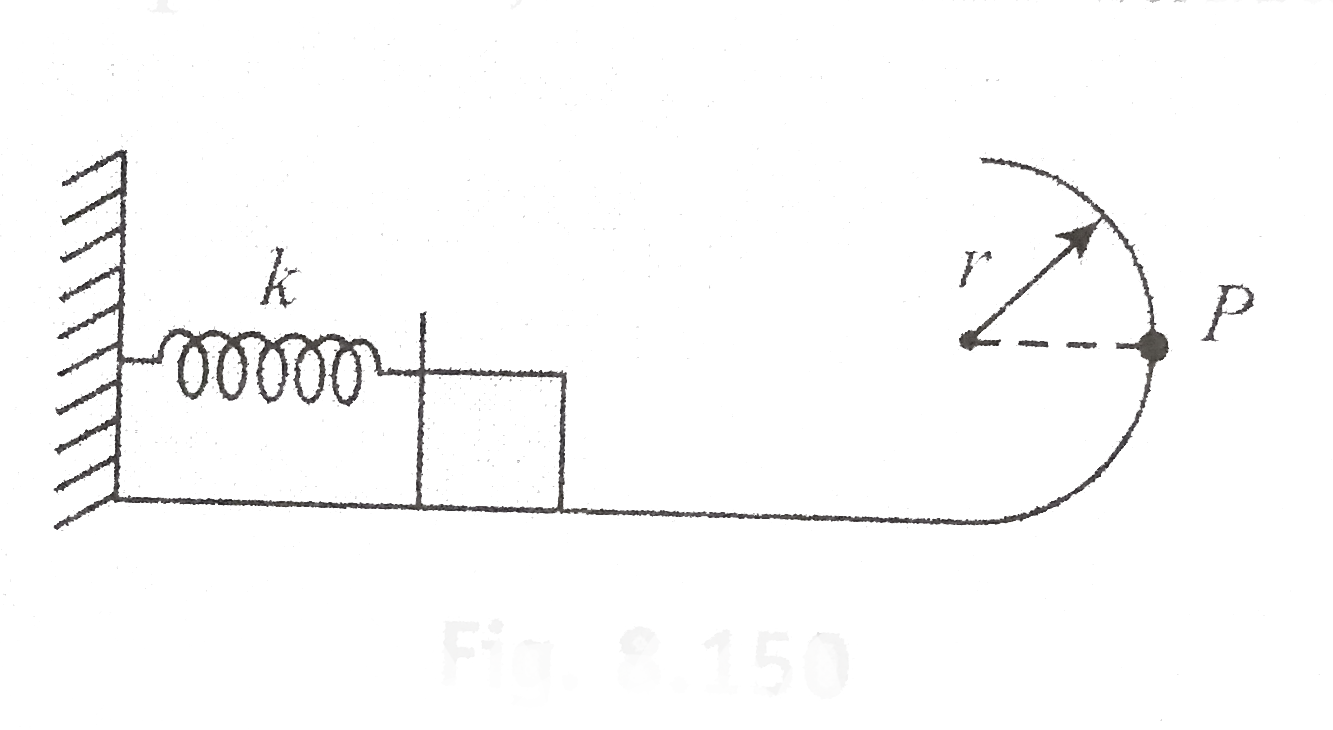
View SolutionA vertical spring with force constant k is fixed on a table. A ball of mass m at a height h above the free upper end of the spring falls vertically on the spring , so that the spring is compressed by a distance d. The net work done in the process is
View SolutionTwo blocks A and B of masses m and 2m, respectively are connected by a spring of force constant k. The masses are moving to the right with uniform velocity v each, the heavier mass leading the lighter one. The spring is in the natural length during this motion. Block B collides head on with a third block C of mass m, at rest, the collision being completely inelastic. Calculate the maximum compression of the spring.
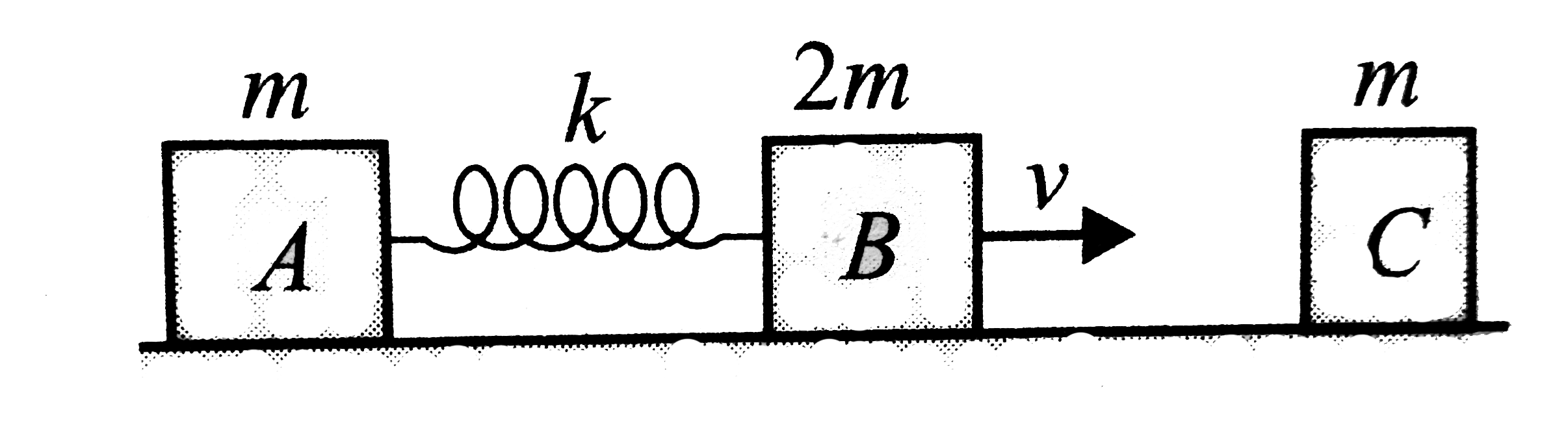
View SolutionAccording to the principle of conservation of linear momentum, if the external force acting on the system is zero, the linear momentum of the system will remain conserved. It means if the centre of mass of a system is initially at rest, it will remain, at rest in the absence of external force, that is, the displacement of centre of mass will be zero.
Two blocks of masses 'm'and '2m' are placed as shown in Fig. There is no friction anywhere. A spring of force constant k is attached to the bigger block. Mass 'm' is kept in touch with the spring but not attached to it. 'A' and 'B' are two supports attached to '2m'. Now m is moved towards left so that spring is compressed by distance 'x' and then the system is released from rest.

Find the relative velocity of the blocks after 'm' leaves contact with the spring.
View SolutionA spring is stretched by distance x and then compressed to same distance from its original length. The work done by the spring force is (k=spring constant)
View SolutionA ball of mass m is droppped from a height h on a platform fixed at the top of a vertical spring. The platform is depressed by a distance x. What is the spring constant K?
View SolutionA block of mass m is connect to another block of mass M by a massless spring of spring constant k. The blocks are kept on a smooth horizontal plane. Initially the blocks are at rest and the spring is upstretched when a constant force F starts acting on the block of mass M to pull it. Find the maximum extension of the spring.

View SolutionA block of mass m starts at rest at height on a frictionless inclined plane. The block slides down the plane, travels across a rough horizontal surface with coefficient of kinetic friction μ and compresses a spring with force constant k a distance x before momentarilly coming to rest. Then the spring extends and the block travels back across the rough surface, sliding up the plane. The block travels a total distance d on rough horizontal surface. The correct experssion for the maximum height h' that the block reaches on its return is :

View SolutionA block of mass m starts at rest at height on a frictionless inclined plane. The block slides down the plane, travels across a rough horizontal surface with coefficient of kinetic friction μ and compresses a spring with force constant k a distance x before momentarilly coming to rest. Then the spring extends and the block travels back across the rough surface, sliding up the plane. The block travels a total distance d on rough horizontal surface. The correct experssion for the maximum height h' that the block reaches on its return is :

View Solution