A man crosses a river in a boat. If he cross the river in minimum time he takes 10min with a drift 120m. If he crosses the river taking shortest path, he takes 12.5min, find
(a) width of the river
(b) velocity of the boat with respect to water
(c) speed of the current
To solve the problem step by step, we will use the information provided about the time taken to cross the river and the drift caused by the current.
Given:
1. Time taken to cross the river in minimum time (perpendicular to the current) = 10 minutes
2. Drift caused during this time = 120 m
3. Time taken to cross the river taking the shortest path = 12.5 minutes
Step 1: Convert time from minutes to seconds
Since we need to work in standard units (meters and seconds), we convert the time from minutes to seconds.
- Time in seconds for minimum time:
t1=10 min=10×60=600 s
- Time in seconds for shortest path:
t2=12.5 min=12.5×60=750 s
Step 2: Calculate the speed of the current (velocity of water)
The drift caused by the current when crossing in minimum time can be calculated using the formula:
Drift=Velocity of water×Time taken
Let vw be the velocity of water.
From the drift information:
120=vw×600
Solving for vw:
vw=120600=0.2 m/s
Step 3: Calculate the width of the river
In the case of crossing in the shortest path, the boat's velocity with respect to the water and the current's velocity combine to give the resultant velocity across the river.
Let vbw be the velocity of the boat with respect to water. The effective velocity across the river (perpendicular to the current) can be expressed using Pythagorean theorem:
vg=√v2bw−v2w
where vg is the velocity of the boat with respect to the ground.
The time taken to cross the river using the shortest path is given by:
Width of the river=vg×t2
Substituting vg:
Width=√v2bw−v2w×750
Step 4: Relate the two cases
From the first case, the width of the river can also be expressed as:
Width=vbw×t1
Equating the two expressions for width:
vbw×600=√v2bw−(0.2)2×750
Step 5: Solve for vbw
Squaring both sides:
(vbw×600)2=(v2bw−0.04)×(7502)
Expanding and simplifying:
360000v2bw=562500v2bw−3000
Rearranging gives:
562500v2bw−360000v2bw=3000
202500v2bw=3000
v2bw=3000202500=167.5
vbw=√167.5≈0.122 m/s
Step 6: Calculate the width of the river
Substituting vbw back into the width equation:
Width=0.122×600≈73.2 m
Final Answers:
(a) Width of the river: approximately 73.2 m
(b) Velocity of the boat with respect to water: approximately 0.122 m/s
(c) Speed of the current: 0.2 m/s
1. Time taken to cross the river in minimum time (perpendicular to the current) = 10 minutes
2. Drift caused during this time = 120 m
3. Time taken to cross the river taking the shortest path = 12.5 minutes
Since we need to work in standard units (meters and seconds), we convert the time from minutes to seconds.
t1=10 min=10×60=600 s
t2=12.5 min=12.5×60=750 s
The drift caused by the current when crossing in minimum time can be calculated using the formula:
Drift=Velocity of water×Time taken
Let vw be the velocity of water.
120=vw×600
Solving for vw:
vw=120600=0.2 m/s
In the case of crossing in the shortest path, the boat's velocity with respect to the water and the current's velocity combine to give the resultant velocity across the river.
vg=√v2bw−v2w
where vg is the velocity of the boat with respect to the ground.
Width of the river=vg×t2
Substituting vg:
Width=√v2bw−v2w×750
From the first case, the width of the river can also be expressed as:
Width=vbw×t1
Equating the two expressions for width:
vbw×600=√v2bw−(0.2)2×750
Squaring both sides:
(vbw×600)2=(v2bw−0.04)×(7502)
Expanding and simplifying:
360000v2bw=562500v2bw−3000
Rearranging gives:
562500v2bw−360000v2bw=3000
202500v2bw=3000
v2bw=3000202500=167.5
vbw=√167.5≈0.122 m/s
Substituting vbw back into the width equation:
Width=0.122×600≈73.2 m
(a) Width of the river: approximately 73.2 m
(b) Velocity of the boat with respect to water: approximately 0.122 m/s
(c) Speed of the current: 0.2 m/s
Topper's Solved these Questions
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Solved Examples
7 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise 5.1
15 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Integer
9 Videos
- KINETIC THEORY OF GASESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASESEXERCISE - Compression
2 Videos
Similar Questions
A river of width 80m is flowing at 6 m/s. A motorboat crosses the river in 10s and reaches a point directly across on the other river bank. The velocity of motorboat in still water is :
View SolutionA river is flowing towards with a velocity of 5ms−1. The boat velocity is 10ms−1. The boat crosses the river by shortest path. Hence,
View SolutionA river is flowing towards with a velocity of 5ms−1. The boat velocity is 10ms−1. The boat crosses the river by shortest path. Hence,
View SolutionA swimmer crosses the river along the line making an angle of 45∘ with the direction of flow. Velocity of the river water is 5ms. Swimmer takes 12 seconds to cross the river of width 60 m. The velocity of the swimmer with respect to water will be:
View SolutionA swimmer crosses the river along the line making an angle of 45∘ with the direction of flow. Velocity of the river water is 5ms. Swimmer takes 12 seconds to cross the river of width 60 m. The velocity of the swimmer with respect to water will be:
View Solution2 km wide river flowing with a rate of 5 km/hr. A man can swim in the still water with10 km/hr. He wants to cross the river along shortest path find
Crossing time
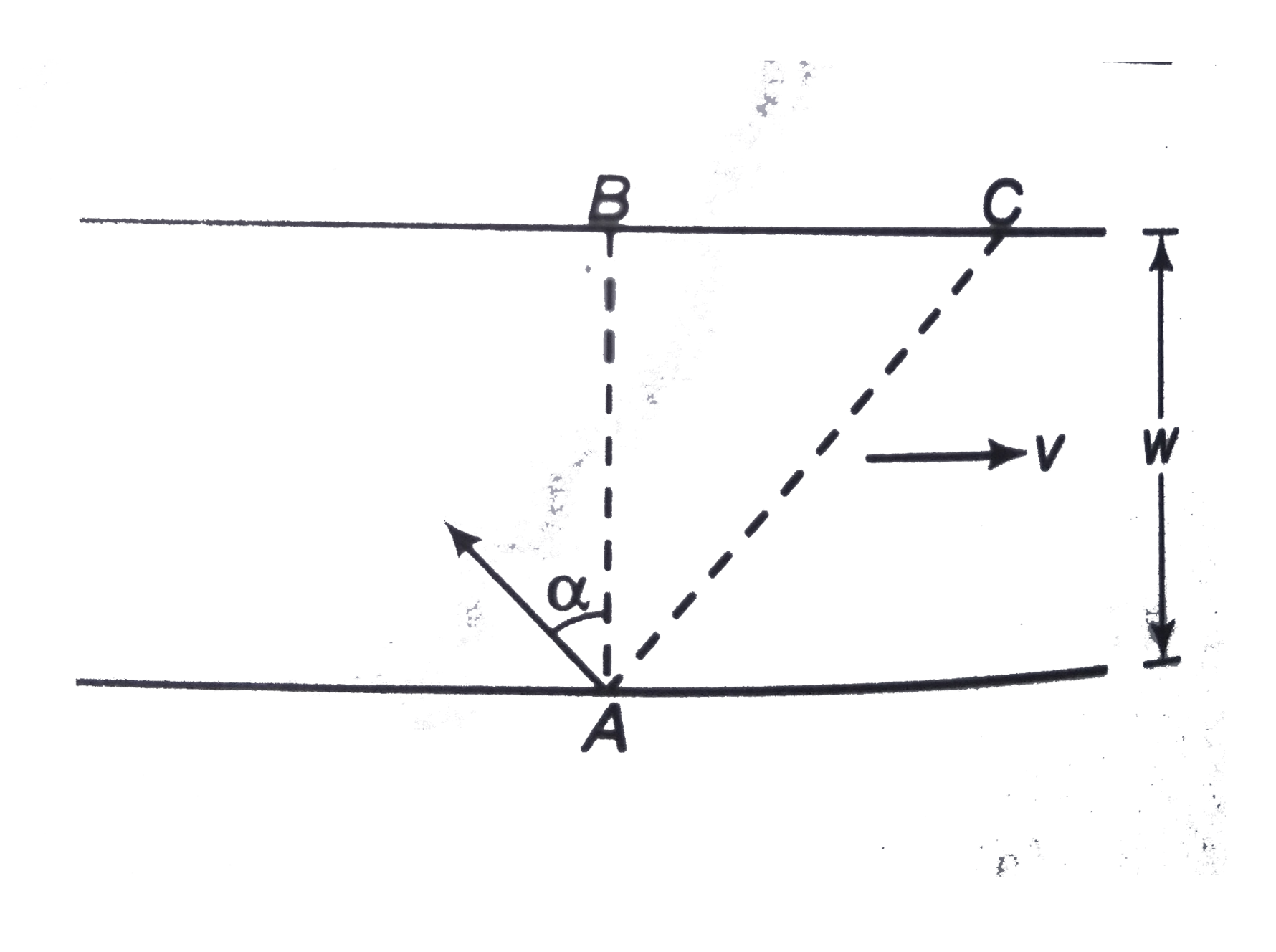
View SolutionA man in a boat crosses a river from point A. If he rows perpendicular to the banks he reaches point C (BC=120m) in 10 min. If the man heads at a certain angle α to the straight line AB (AB is perpendicular to the banks) against the current he reaches point B in 12.5 min. Find the width of the river w, the rowing velocity u, the speed of the river current v and the angle α. Assume the velocity of the boat relative to water to be constant and the same magnitude in both cases.
View SolutionVelocity of boat in still water is 13 m/s. If water flows ina river with a velocity of 5 m/s. What is the difference in times taken by him to cross the river in the shortest path and in the shortest time. If the width of the river is 10 m.
View SolutionThe velocity of a boat in still water is 10 m/s. If water flows in the river with a velocity of 6 m/s what is the difference in times taken to cross the river in the shortest path and the shortest time. The width of the river is 80 m.
View SolutionA swimmer crosses a river of width d flowing at velocity v. While swimming, he keeps himself always at an angle of 120∘ with the river flow and on reaching the other end. He finds a drift of d/2 in the direction of flow of river. The speed of the swimmer with respect to the river is :
View Solution
