A particle is projected up an inclined plane of inclination β at an elevation ∝ to the horizontal. Find the ratio between tan∝andtanβ, if the particle strikes the plane horizontally.
The correct Answer is:(2)
To solve the problem, we need to find the ratio between tanα and tanβ given that a particle is projected up an inclined plane at an angle α to the horizontal and strikes the plane horizontally.
1. Understanding the Problem:
- A particle is projected at an angle α to the horizontal.
- The inclined plane has an angle β with the horizontal.
- The particle strikes the inclined plane horizontally.
2. Identify the Conditions:
- When the particle strikes the plane horizontally, it means that at the point of impact, the vertical component of its velocity is zero.
3. Using Kinematic Equations:
- The time of flight for the projectile can be derived using the vertical motion equations. The time taken to reach the maximum height is given by:
t=usinαg
- The total time of flight until it strikes the inclined plane is twice this time (up and down):
T=2t=2usinαg
4. Finding the Maximum Height:
- The maximum height H reached by the projectile is given by:
H=u2sin2α2g
5. Finding the Range on the Inclined Plane:
- The horizontal range R of the projectile can be calculated as:
R=u2sin(2α)g
- However, since the particle strikes the plane horizontally, we need to consider the effective range along the inclined plane.
6. Relating Height and Range to the Incline:
- The height H can be related to the angle of the incline β using the tangent function:
tanβ=HR
7. Substituting Values:
- Substitute H and R into the equation:
tanβ=u2sin2α2gu2sin(2α)g
- Simplifying this gives:
tanβ=sin2α2sin(2α)
- Since sin(2α)=2sinαcosα, we can write:
tanβ=sin2α4sinαcosα=sinα4cosα=14tanα
8. Finding the Ratio:
- Rearranging gives us:
tanα=4tanβ
- Therefore, the ratio is:
tanαtanβ=4
Final Answer:
The ratio between tanα and tanβ is:
tanαtanβ=4
- A particle is projected at an angle α to the horizontal.
- The inclined plane has an angle β with the horizontal.
- The particle strikes the inclined plane horizontally.
- When the particle strikes the plane horizontally, it means that at the point of impact, the vertical component of its velocity is zero.
- The time of flight for the projectile can be derived using the vertical motion equations. The time taken to reach the maximum height is given by:
t=usinαg
- The total time of flight until it strikes the inclined plane is twice this time (up and down):
T=2t=2usinαg
- The maximum height H reached by the projectile is given by:
H=u2sin2α2g
- The horizontal range R of the projectile can be calculated as:
R=u2sin(2α)g
- However, since the particle strikes the plane horizontally, we need to consider the effective range along the inclined plane.
- The height H can be related to the angle of the incline β using the tangent function:
tanβ=HR
- Substitute H and R into the equation:
tanβ=u2sin2α2gu2sin(2α)g
- Simplifying this gives:
tanβ=sin2α2sin(2α)
- Since sin(2α)=2sinαcosα, we can write:
tanβ=sin2α4sinαcosα=sinα4cosα=14tanα
- Rearranging gives us:
tanα=4tanβ
- Therefore, the ratio is:
tanαtanβ=4
The ratio between tanα and tanβ is:
tanαtanβ=4
Topper's Solved these Questions
- KINEMATICS-2Book:CENGAGE PHYSICS ENGLISHChapter:KINEMATICS-2Exercise:Exercise Comprehension
Explore 21 Videos
- KINEMATICS-1Book:CENGAGE PHYSICS ENGLISHChapter:KINEMATICS-1Exercise:Integer
Explore 9 Videos
- KINETIC THEORY OF GASESBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASESExercise:Compression
Explore 2 Videos
Similar Questions
A particle is projected up an inclined plane of inclination β at na elevation ∝ to the horizontal. Show that
(a) tan∝=cotβ+2tanβ, if the particle strikes the plane at right angles
(b) tan∝=2tanβ, if the particle strikes the plane horizontally.
View SolutionA particle is projected up an inclined plane of inclination β at na elevation ∝ to the horizon. Show that tan∝=cotβ+2tanβ, if the particle strikes the plane at right angles
View SolutionA particle is projected up an inclines plane. Plane is inclined at an angle θ with horizontal and particle is projected at an angle α with horizontal. If particle strikes the plane horizontally prove that tanα=2tanθ
View SolutionA particle is projected from the bottom of an inclined plane of inclination 30∘. At what angle α (from the horizontal) should the particle be projected to get the maximum range on the inclined plane.
View SolutionA particle is projected with a certain velocity at an angle ∝ above the horizontal from the foot of an inclined plane of inclination 30∘. If the particle strikes the plane normally, then ∝ is equal to.
View SolutionA particle is projected horizontally with a speed u from the top of plane inclined at an angle θ with the horizontal. How far from the point of projection will the particle strike the plane ?

View SolutionA particle is projected from the bottom of an inclined plane of inclination 30∘ with velocity of 40m/s at an angle of 60∘ with horizontal. Find the speed of the particle when its velocity vector is parallel to the plane. Take g=10m/s2.
View SolutionA particle is projected with a certain velocity at an angle α above the horizontal from the foot of an inclined plane of inclination 30∘. If the particle strikes the plane normally then α is equal to
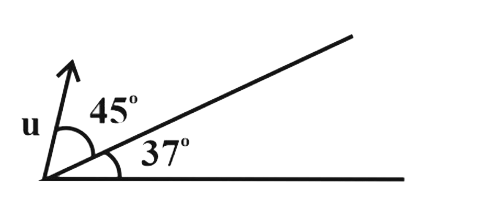
View SolutionA particle is projected on a frictionless inclined plane of inclination 37∘ at an angle of projection 45∘ from the inclined plane as shown in the figure. If after the first collision from the plane, the particle returns to its point of projection, then what is the value of the reciprocal of the coefficient of restitution between the particle and the plane?
View SolutionA ball falls freely form a height onto and smooth inclined plane forming an angle a with the horizontal. Find the ratio of the distance between the points at which the jumping ball strikes the inclined plane. Assume the impacts to be elastic.
View Solution