A swimmer crosses the river along the line making an angle of 45∘ with the direction of flow. Velocity of the river water is 5ms. Swimmer takes 12 seconds to cross the river of width 60 m. The velocity of the swimmer with respect to water will be:
10ms
5ms
5√5ms
5√2ms
The correct Answer is:B
To solve the problem, we need to determine the velocity of the swimmer with respect to the water. Let's break down the solution step by step.
Step 1: Understand the Given Information
- The swimmer is crossing a river at an angle of 45∘ to the direction of the river flow.
- The velocity of the river water is 5m/s.
- The width of the river is 60m.
- The time taken to cross the river is 12s.
Step 2: Calculate the Velocity of the Swimmer in the Y-Direction
The swimmer crosses the river of width 60m in 12s. The velocity in the y-direction (the direction across the river) can be calculated using the formula:
Vy=Width of riverTime taken=60m12s=5m/s
Step 3: Determine the Components of the Swimmer's Velocity
Since the swimmer is swimming at an angle of 45∘ to the direction of the river flow, we can denote the swimmer's velocity as V. The components of the swimmer's velocity can be expressed as:
Vx=Vcos(45∘)andVy=Vsin(45∘)
Given that Vy=5m/s, we can find V:
Vsin(45∘)=5m/s⟹V⋅1√2=5⟹V=5√2m/s
Step 4: Calculate the Components of the Swimmer's Velocity
Now we can find the x-component of the swimmer's velocity:
Vx=Vcos(45∘)=5√2⋅1√2=5m/s
Step 5: Combine the Velocities
The swimmer's velocity vector can be represented as:
→Vswimmer=Vx^i+Vy^j=5^i+5^jm/s
Step 6: Calculate the Velocity of the Swimmer with Respect to Water
The velocity of the river current is:
→Vwater=5^im/s
To find the velocity of the swimmer with respect to the water, we subtract the velocity of the water from the velocity of the swimmer:
→Vswimmer, water=→Vswimmer−→Vwater=(5^i+5^j)−(5^i)=5^jm/s
Step 7: Find the Magnitude of the Velocity
The magnitude of the swimmer's velocity with respect to the water is:
|→Vswimmer, water|=5m/s
Final Answer
The velocity of the swimmer with respect to water is 5m/s.
---
- The swimmer is crossing a river at an angle of 45∘ to the direction of the river flow.
- The velocity of the river water is 5m/s.
- The width of the river is 60m.
- The time taken to cross the river is 12s.
The swimmer crosses the river of width 60m in 12s. The velocity in the y-direction (the direction across the river) can be calculated using the formula:
Since the swimmer is swimming at an angle of 45∘ to the direction of the river flow, we can denote the swimmer's velocity as V. The components of the swimmer's velocity can be expressed as:
Now we can find the x-component of the swimmer's velocity:
The swimmer's velocity vector can be represented as:
The velocity of the river current is:
The magnitude of the swimmer's velocity with respect to the water is:
The velocity of the swimmer with respect to water is 5m/s.
Similar Questions
A man crosses the river in shortest time and reaches at an angle θ=60∘ to the direction of flow of water. If the speed of water is vw=4km/hr, find the speed of the man:
View SolutionA swimmer crosses a river with minimum possible time 10 Second. And when he reaches the other end starts swimming in the direction towards the point from where he started swimming. Keeping the direction fixed the swimmer crosses the river in 15 sec. The ratio of speed of swimmer with respect to water and the speed of river flow is (Assume contant speed of river & swimmer)
View SolutionA man wishes to cross a river in a boat. If he crosses the river in minimum time he takes 10min with a drift of 120m. If he crosses the river taking shortest route, he takes 12.5min. Find the velocity of the boat with respect to water.
View SolutionA man crosses a river in a boat. If he cross the river in minimum time he takes 10min with a drift 120m. If he crosses the river taking shortest path, he takes 12.5min, find
(a) width of the river
(b) velocity of the boat with respect to water
(c) speed of the currentView SolutionA bridge across a river makes an angles of 450 with the river bank as shown in Figure. If the lengthof the bridge across the river is 150m, what is the width of the river?
View SolutionA swimmer crosses a river of width d flowing at velocity v. While swimming, he keeps himself always at an angle of 120∘ with the river flow and on reaching the other end. He finds a drift of d/2 in the direction of flow of river. The speed of the swimmer with respect to the river is :
View SolutionA swimmer starts to swim from point a to cross a river. He wants to reach point B on the opposite side of the river. The line AB makes an angle 60∘ with the river flow as shown. The velocity of the swimmer in still water is same as that of the water
(i) In what direction should he try to direct his velocity ? Calculate angle between his velocity ? Calculate angle between his velocity and river velocity.
(ii) Find the ratio of the time taken to cross the river in this situation to the minimum time in which he can cross this river.
 View Solution
View SolutionA boat is rowed across a river at the rate of 4.5kmhr. The river flows at the rate of 6kmhr. The velocity of boat in ms is:
View SolutionA man swims across a river with speed of Vm perpendicular to the flow direction of river. If the water flows with a speed Vw with what resullant velocity does the man cross the river ?
View SolutionTwo swimmers start a race. One who reaches the point C first on the other bank wins the race.A makes his strokes in a direction of 370 to the river flow with velocity 5km/hr relative to water. B makes his strokes in a direction 1270 to the river flow with same relative velocity.River is flowing with speed of 2km/hr and is 100m wide.speeds of A and B on the ground are 8km/hr and 6km/hr respectively.
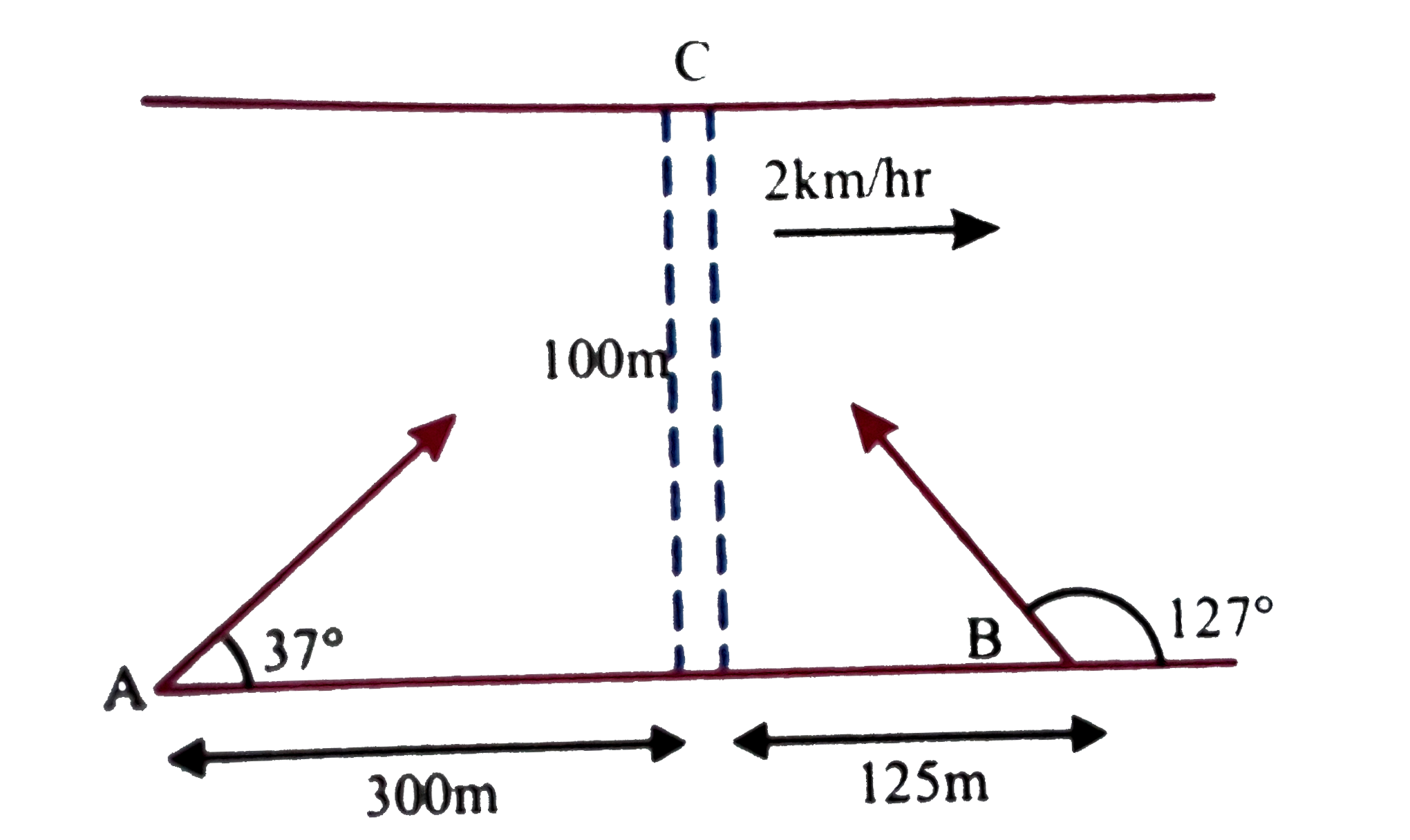 View Solution
View Solution
