A river is flowing towards with a velocity of 5ms−1. The boat velocity is 10ms−1. The boat crosses the river by shortest path. Hence,
(a)The direction of boat’s velocity is 30∘ west of north
(b)The direction of boat’s velocity is north-west
(c)Resultant velocity is 5√3ms−1
(d)Resultant velocity is 5√2ms−1
The correct Answer is:ac
To solve the problem of a boat crossing a river with a current, we will analyze the situation step by step.
Step 1: Understand the Given Information
- Velocity of the river (Vr) = 5 m/s (flowing towards the east)
- Velocity of the boat (Vb) = 10 m/s (the boat crosses the river)
Step 2: Determine the Shortest Path
To cross the river by the shortest path, the boat must head upstream at an angle such that its resultant velocity allows it to reach the opposite bank directly across from its starting point.
Step 3: Set Up the Diagram
We can visualize the situation with a right triangle:
- The vertical side represents the width of the river (let's denote it as 'd').
- The horizontal side represents the velocity of the river (Vr = 5 m/s).
- The hypotenuse represents the velocity of the boat (Vb = 10 m/s).
Step 4: Apply Trigonometry
Using trigonometric relationships, we can find the angle (θ) at which the boat must head to cross directly:
- The sine of the angle θ can be expressed as:
sin(θ)=VrVb=510=12
- From this, we find:
θ=30∘
Step 5: Determine the Direction of the Boat
Since the river flows east and the boat heads upstream, the angle θ is measured from the north towards the west. Thus, the direction of the boat is:
- 30° west of north.
Step 6: Calculate the Resultant Velocity of the Boat
To find the resultant velocity of the boat relative to the ground, we can use the Pythagorean theorem:
Vr=√Vb2−Vr2=√102−52=√100−25=√75=5√3m/s
Step 7: Conclusion
Based on the calculations:
- The direction of the boat is 30° west of north.
- The resultant velocity of the boat relative to the ground is 5√3 m/s.
Final Answer
- The correct options are:
- Direction of the boat: 30° west of north (Option A is correct).
- Resultant velocity: 5√3 m/s (Option C is correct).
- Velocity of the river (Vr) = 5 m/s (flowing towards the east)
- Velocity of the boat (Vb) = 10 m/s (the boat crosses the river)
To cross the river by the shortest path, the boat must head upstream at an angle such that its resultant velocity allows it to reach the opposite bank directly across from its starting point.
We can visualize the situation with a right triangle:
- The vertical side represents the width of the river (let's denote it as 'd').
- The horizontal side represents the velocity of the river (Vr = 5 m/s).
- The hypotenuse represents the velocity of the boat (Vb = 10 m/s).
Using trigonometric relationships, we can find the angle (θ) at which the boat must head to cross directly:
- The sine of the angle θ can be expressed as:
sin(θ)=VrVb=510=12
- From this, we find:
θ=30∘
Since the river flows east and the boat heads upstream, the angle θ is measured from the north towards the west. Thus, the direction of the boat is:
- 30° west of north.
To find the resultant velocity of the boat relative to the ground, we can use the Pythagorean theorem:
Vr=√Vb2−Vr2=√102−52=√100−25=√75=5√3m/s
Based on the calculations:
- The direction of the boat is 30° west of north.
- The resultant velocity of the boat relative to the ground is 5√3 m/s.
- The correct options are:
- Direction of the boat: 30° west of north (Option A is correct).
- Resultant velocity: 5√3 m/s (Option C is correct).
Topper's Solved these Questions
- INTRODUCTION TO VECTORS & FORCES Book:VMC MODULES ENGLISHChapter:INTRODUCTION TO VECTORS & FORCES Exercise:JEE Main ( ARCHIVE ) ( LEVEL-1)
Explore 12 Videos
- INTRODUCTION TO VECTORS & FORCES Book:VMC MODULES ENGLISHChapter:INTRODUCTION TO VECTORS & FORCES Exercise:JEE Advanced ( ARCHIVE LEVEL-2)
Explore 12 Videos
- INTRODUCTION TO VECTORS & FORCES Book:VMC MODULES ENGLISHChapter:INTRODUCTION TO VECTORS & FORCES Exercise:level 1
Explore 75 Videos
- GRAVITATIONBook:VMC MODULES ENGLISHChapter:GRAVITATIONExercise:JEE Advance (Archive) TRUE/FALSE
Explore 1 Video
- JEE MAIN - 5Book:VMC MODULES ENGLISHChapter:JEE MAIN - 5Exercise:PART I : PHYSICS (SECTION - 2)
Explore 5 Videos
Similar Questions
A river is flowing with a velocity of 2m/s. If the width of river in 100 m and swimmer wants to cross river is shortest time, what time (in sec) would he take if velocity of swimmer in still water is 4 m/s ?
View SolutionVelocity of boat in still water is 13 m/s. If water flows ina river with a velocity of 5 m/s. What is the difference in times taken by him to cross the river in the shortest path and in the shortest time. If the width of the river is 10 m.
View SolutionThe velocity of a boat in still water is 10 m/s. If water flows in the river with a velocity of 6 m/s what is the difference in times taken to cross the river in the shortest path and the shortest time. The width of the river is 80 m.
View SolutionWidth of a river is 30m, velocity is 4m/s and rowing velocity is 5m/s
(a) Make the velocity diagram for crossing the river in shortest time. Then, find this shortest time, net velocity of boatman and drigt along the river.
(b) Can the boatman reach a point just oppsite on the other shore? If yes then make the velocity diagram, the direction in which the should row his boat and the time taken to cross the river in this case.
(c) How long will it iake hom to row 10m up the stream and then back to his starting point?
View SolutionA 400m wide river is flowing at a rate of 2.0ms−1. A boat is sailing with a velocity of 10ms−1 with respect to the water, in a direction perpendicular to the river.
(a) Find the time taken by the boat to reach the opposite bank.
(b) How far from the point directly opposite to the starting point does the boat reach the opposite bank ?
( c) In what direction does the boat actually move ?
View Solution2 km wide river flowing with a rate of 5 km/hr. A man can swim in the still water with10 km/hr. He wants to cross the river along shortest path find
Crossing time
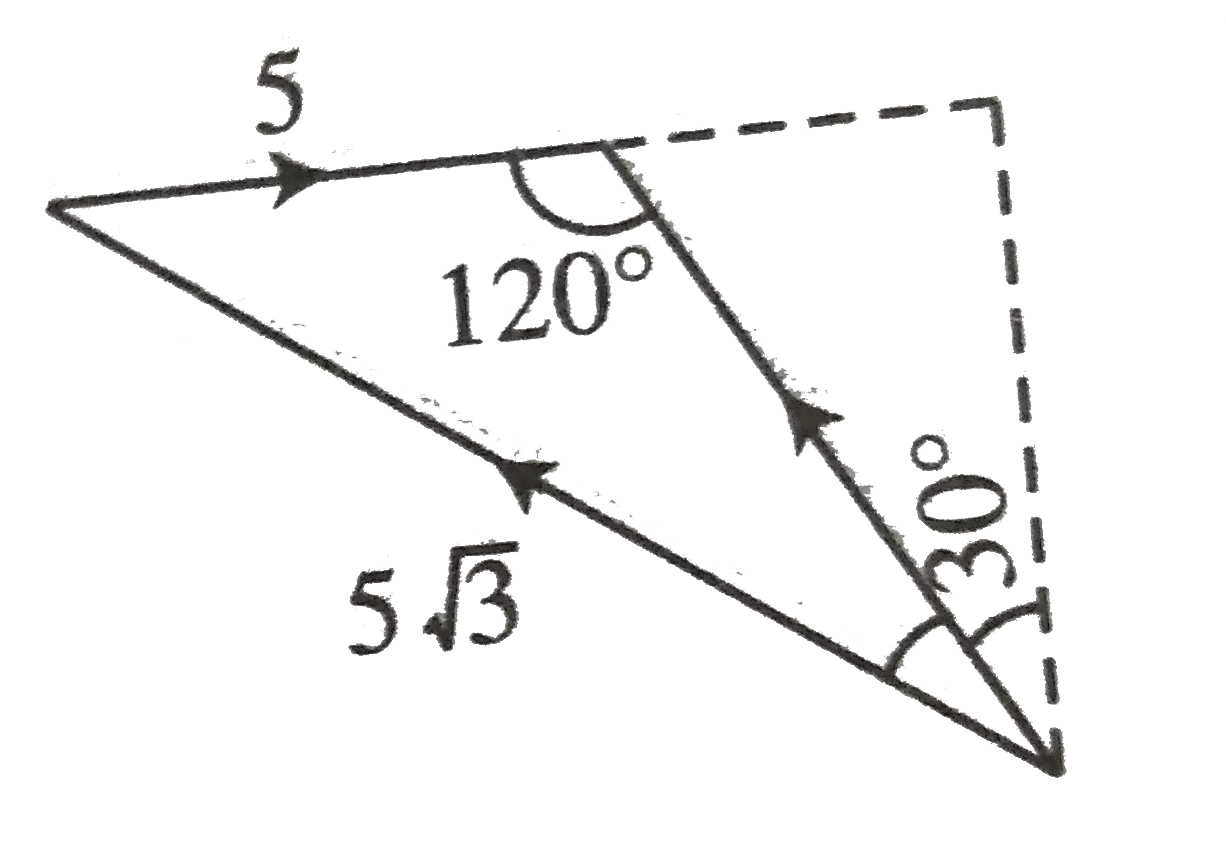
View SolutionA motor boat is to reach at a point 30∘ upstream on the outer side of a river flowing with velocity 5ms−1. The velocity of motor boat with respect to water is 5(√3)ms−1. The driver should steer the boat at an angle.
View SolutionAt a harbour, a boat is standing and wind is blowing at a speed of √2m/sec. due to which , the flag on the boat flutters along north− east. Now the boat enters in to river, which is flowing with a velocity of 2m/sec. Due north. The boat starts with zero velocity relative to the river and its constant acceleration relative to the river is 0.2m/sec2 due east. In which direction will the flag flutter at 10 seconds ?
View SolutionA boat is moving towards east velocity 4 m/s w.r.t still water and river is flowing towards north with velocity 2 m/s and the wind blowing towards north with velocity 6 m/s. the direction of the flag blown over by the wind hpisted on the boat is
View SolutionThe width of river is 1 km. The velocity of boat is 5 km/hr. The boat covered the width of river with shortest will possible path in 15 min. Then the velocity of river stream is:
View Solution