A ray incident on the face of a prism is refracted and escape through an adjacent face. What is the maximum permissible angle of the prism, if it is made of glass with a refractive index of μ=1.5?
The correct Answer is:A, D
To solve the problem of finding the maximum permissible angle of a prism made of glass with a refractive index of μ=1.5, we can follow these steps:
Step 1: Understand the Concept of Critical Angle
The critical angle θC is the angle of incidence above which total internal reflection occurs. It can be calculated using the formula:
θC=sin−1(1μ)
where μ is the refractive index of the material.
Step 2: Calculate the Critical Angle
Given μ=1.5:
θC=sin−1(11.5)=sin−1(23)
Using a calculator, we find:
θC≈42∘
Step 3: Relate Angles in the Prism
For a prism, when a ray of light enters and exits, the angles of incidence and refraction can be related. Let r1 be the angle of refraction at the first face, and r2 be the angle of refraction at the second face. The angle A of the prism can be expressed as:
A=r1+r2
Step 4: Apply the Condition for Maximum Angle
To maximize the angle A, we can use the symmetry of the situation. The maximum angle occurs when r1=r2. Thus, we can write:
A=2r1
Since r1 must be less than or equal to the critical angle θC:
r1≤θC
This leads to:
A≤2θC
Step 5: Substitute the Critical Angle
Substituting the value of the critical angle:
A≤2×42∘=84∘
Conclusion
The maximum permissible angle of the prism is:
84∘
The critical angle θC is the angle of incidence above which total internal reflection occurs. It can be calculated using the formula:
θC=sin−1(1μ)
where μ is the refractive index of the material.
Given μ=1.5:
θC=sin−1(11.5)=sin−1(23)
Using a calculator, we find:
θC≈42∘
For a prism, when a ray of light enters and exits, the angles of incidence and refraction can be related. Let r1 be the angle of refraction at the first face, and r2 be the angle of refraction at the second face. The angle A of the prism can be expressed as:
A=r1+r2
To maximize the angle A, we can use the symmetry of the situation. The maximum angle occurs when r1=r2. Thus, we can write:
A=2r1
Since r1 must be less than or equal to the critical angle θC:
r1≤θC
This leads to:
A≤2θC
Substituting the value of the critical angle:
A≤2×42∘=84∘
The maximum permissible angle of the prism is:
84∘
Topper's Solved these Questions
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Subjective Questions
8 Videos
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Level 2 Single Correct
41 Videos
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Objective Questions
1 Video
- REFLECTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFLECTION OF LIGHTEXERCISE - Subjective
9 Videos
- SEMICONDUCTORSBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORSEXERCISE - Subjective
12 Videos
Similar Questions
A ray of light incident at an angle θ on a refracting face of a prism emerges from the other face normally. If the angle of the prism is 5∘ and the prism is made of a material of refractive index 1.5, the angle of incidence is.
View SolutionA ray of light incident at an angle θ on a refracting face of a prism emerges from the other face normally. If the angle of the prism is 5∘ and the prism is made of a material of refractive index 1.5, the angle of incidence is.
View Solution
Knowledge Check
A ray of light incident at an angle θ on a refracting face of a prism emerges from the other face normally. If the angle of the prism is 5∘ and the prism is made of a material of refractive index 1.5, the angle of incidence is.
A7.5∘B5∘C15∘D2.5∘
At what angle should a ray of light be incident on the face of a prism of refracting angle 60∘ so that it just suffers total internal reflection at the other face ? The refractive index of the prism is 1.524.
View Solution
A ray of light incident on one of the face of a prism as shown. After refraction through this surface ray is incident on base of the prism. The refractive index of the prism is √3. The net deviation of the ray due to prism is
View SolutionIf a light ray incidents normally on one of the faces of the prism of refractive index 2 and the emergent ray just grazes the second face of the prism, then the angle of deviation is
View SolutionIf a light ray incidents normally on one of the faces of the prism of refractive index 2 and the emergent ray just grazes the second face of the prism, then the angle of deviation is
View Solution
A ray of light is incident normally on one face of a prism as shown in figure. The refractive index of the material of the prism is 53 and the prism is immersed in water of refractive index 43, then
View SolutionA ray of light is incident on one face of a prism (m=√3) at an angle of 60∘. The refracting angle of the prism is also 60∘. Find the angle of emergence .
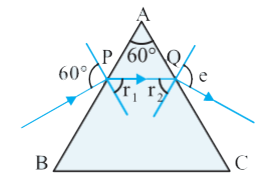
View SolutionA prism is made of glass of unknown refractive index. A parallel beam of light is incident on a face of the prism. By rotating the prism, the minimum angle of deviation is measured to be 40∘. What is the refractive index of the prism ? If the prism is placed in water (μ=1.33), predict the new angle of minimum deviation of the parallel beam. The refracting angle of prism is 60∘.
View Solution
