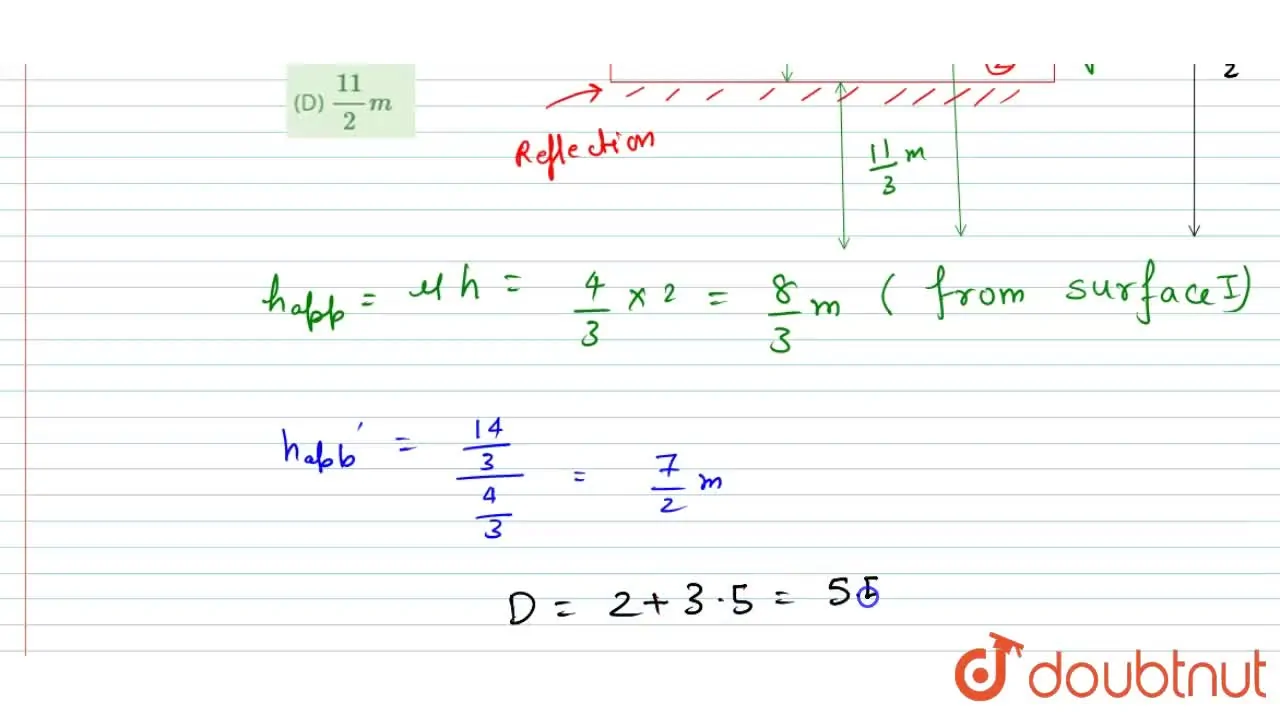
A bird is flying over a swimming pool at a height of 2m from the water surface. If the bottom is perfectly plane reflecting surface and depth of swimming pool is 1m, then the distance of final image of bird from the bird itself is μw=4/3
(A) 113m
(B) 233m
(C) 114m
(D) 112m
The correct Answer is:D
happ=μh=43×2=83m
Distanceommirror=1+happ=113m. So, mirror
will make image at same distance (=11/3m from itself).
Now in third refraciton, depth of second
image,
d=113+1=143m
dapp=dμ=143×34=72m
The desired distance is therefore,
(dapp+h)
or (72+2)mor112m
Related Playlists
- BOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Single Correct Option3videos
REFRACTION OF LIGHT
- BOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - more than one correct option1videos
REFRACTION OF LIGHT
- BOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Subjective Questions8videos
REFRACTION OF LIGHT
- BOOK - DC PANDEY ENGLISHCHAPTER - REFLECTION OF LIGHTEXERCISE - Subjective9videos
REFLECTION OF LIGHT
- BOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORSEXERCISE - Subjective12videos
SEMICONDUCTORS
Similar Questions
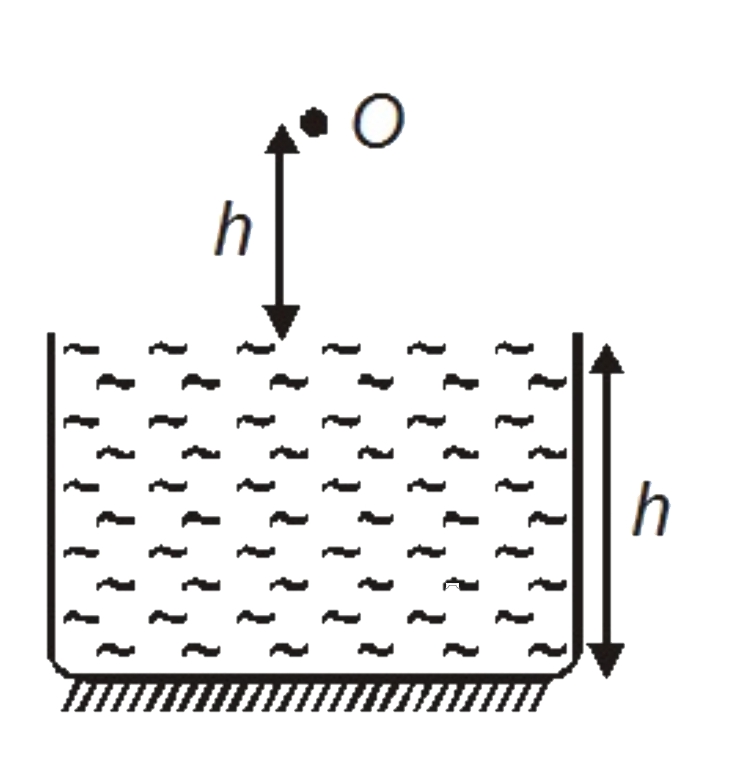
An object O is placed at a height h = 12 cm above water (μ=43) surface in a beaker . The lower surface of the beaker is polished , as shown in the figure . The location of final image will be
 View Solution
View SolutionA fish lies at the bottom of a 4m deep water lake. A bird flies 6m above the water surface and refractive index of water is 4/3 . Then the distance between
View SolutionA vessel having perfectly reflecting plane botton is filled with water (μ=4/3) to depth d. A point source of light is placed at a height h above the surface of water. Find the distance of final image from water surface.
View SolutionAn under water swimmer is at a depth of 12 m below the surface of water. A bird is at a height of 18 m from the surface of water, directly above his eyes. For the swimmer the bird appears to be at a distance from the surface of water equal to (Refractive Index of water is 4/3)
View SolutionWater is filled in a container upto a height of 5cm. There is a concave mirror of radius of curvature 40cm. A person just above the surface observes image of 'A'. The distance of image from water surface is (μwater=1.33):
_2019_CBT7_E01_003_Q01.png) View Solution
View SolutionA fish in a lake (refractive index 4/3 for water ) is viewed through a convex lens. From water surface, the lens is placed in air at half of the distance of the fish from the water surface, so that the image is formed at the fish itself. The focal length of the lens is how many times the depth of fish in water?
View SolutionAn object is at a distance of d=2.5cm from the surface of a glass sphere with a radius R=10cm. Find the position of the final image produced by the sphere. The refractive index of glass is μ=1.5.
View SolutionIn a pond of water, a flame is held 2 m above the surface of the water. A fish is at depth of 4 m from the water surface. Refractive index of water is 43. The apparent height of the flame from the eyes of fish is -
View SolutionA bird is sitting on the top of an 80 m high tree. From a point on the ground, the angle of elevation of the bird is 45∘. The bird flies away horizontally in such a way that it remained at a constant height from the ground. After 2 seconds, the angle of elevation of the bird from the same point is 30∘. Find the speed of flying of the bird. (Take √3=1.732).
View SolutionAn aeroplane is flying over two houses which are at a distance of 300 m from each other. If at some moment from the aeroplane the angle of depression of two houses on the same side are 45∘ and 60∘, at what height the plane is flying ?
View Solution