An object is at a distance of d=2.5cm from the surface of a glass sphere with a radius R=10cm. Find the position of the final image produced by the sphere. The refractive index of glass is μ=1.5.
The correct Answer is:A, B, C, D
To find the position of the final image produced by the glass sphere, we will use the formula for refraction at a spherical surface. The formula is given as:
μ2V=μ1U=μ2−μ1R
Where:
- μ1 is the refractive index of the medium from which light is coming (air, in this case, μ1=1).
- μ2 is the refractive index of the glass (μ2=1.5).
- U is the object distance (given as d=2.5cm).
- V is the image distance we want to find.
- R is the radius of curvature of the glass sphere (given as R=10cm).
Step 1: Determine the object distance U
Since the object is located in front of the spherical surface, we take the object distance as negative:
U=−2.5cm
Step 2: Apply the refraction formula
Using the formula, we can express the relationship as:
1.5V=1−2.5=1.5−110
Step 3: Solve for V
First, we calculate the right-hand side:
1.5−110=0.510=0.05
Now, we can set up the equation:
1.5V=0.05
Cross-multiplying gives:
1.5=0.05V
Now, solving for V:
V=1.50.05=30cm
Step 4: Determine the position of the image
The image formed by the first surface is at 30cm to the right of the surface.
Step 5: Consider the second surface of the sphere
The distance from the first image to the second surface of the sphere is:
Distance to second surface=R=10cm
Thus, the total distance from the object to the second surface is:
Total distance=30cm+10cm=40cm
Step 6: Apply the refraction formula again for the second surface
Now we need to find the image formed by the second surface. We will again use the refraction formula:
1V2=1.540=1.5−1−10
Calculating the right-hand side:
0.5−10=−0.05
Thus, we have:
1.5V2=−0.05
Cross-multiplying gives:
1.5=−0.05V2
Solving for V2:
V2=1.5−0.05=−30cm
Final Result
The final image is located at 30cm to the left of the second surface of the sphere.
Summary
The position of the final image produced by the sphere is:
Final Image Position=−30cm (to the left of the second surface)
- μ1 is the refractive index of the medium from which light is coming (air, in this case, μ1=1).
- μ2 is the refractive index of the glass (μ2=1.5).
- U is the object distance (given as d=2.5cm).
- V is the image distance we want to find.
- R is the radius of curvature of the glass sphere (given as R=10cm).
Since the object is located in front of the spherical surface, we take the object distance as negative:
U=−2.5cm
Using the formula, we can express the relationship as:
1.5V=1−2.5=1.5−110
First, we calculate the right-hand side:
1.5−110=0.510=0.05
Now, we can set up the equation:
1.5V=0.05
Cross-multiplying gives:
1.5=0.05V
Now, solving for V:
V=1.50.05=30cm
The image formed by the first surface is at 30cm to the right of the surface.
The distance from the first image to the second surface of the sphere is:
Distance to second surface=R=10cm
Thus, the total distance from the object to the second surface is:
Total distance=30cm+10cm=40cm
Now we need to find the image formed by the second surface. We will again use the refraction formula:
1V2=1.540=1.5−1−10
Calculating the right-hand side:
0.5−10=−0.05
Thus, we have:
1.5V2=−0.05
Cross-multiplying gives:
1.5=−0.05V2
Solving for V2:
V2=1.5−0.05=−30cm
The final image is located at 30cm to the left of the second surface of the sphere.
The position of the final image produced by the sphere is:
Final Image Position=−30cm (to the left of the second surface)
Topper's Solved these Questions
- REFRACTION OF LIGHTBook:DC PANDEY ENGLISHChapter:REFRACTION OF LIGHTExercise:Subjective Questions
Explore 8 Videos
- REFRACTION OF LIGHTBook:DC PANDEY ENGLISHChapter:REFRACTION OF LIGHTExercise:Level 2 Single Correct
Explore 41 Videos
- REFRACTION OF LIGHTBook:DC PANDEY ENGLISHChapter:REFRACTION OF LIGHTExercise:Objective Questions
Explore 1 Video
- REFLECTION OF LIGHTBook:DC PANDEY ENGLISHChapter:REFLECTION OF LIGHTExercise:Subjective
Explore 9 Videos
- SEMICONDUCTORSBook:DC PANDEY ENGLISHChapter:SEMICONDUCTORSExercise:Subjective
Explore 12 Videos
Similar Questions
A point object is situated at a distance of 36 cm from the centre of the sphere of radius 12 cm and refractive index 1.5. Locate the position of the image due to refraction through sphere.
View SolutionFind the surface area of a sphere of radius: 10.5 cm (ii) 5.6 cm (iii) 14 cm
View SolutionFind the surface area of a sphere of radius: 10.5 cm (ii) 5.6 cm (iii) 14 cm
View SolutionA point object is placed at the center of a glass sphere of radius 6cm and refractive index 1.5. The distance of virtual image from the surface is
View SolutionA small object is placed 20 cm in front of a block of glass 10 cm thick and its farther side silvered. The image is formed 22 cm behind the silvered face. Find the refractive index of glass.
View Solution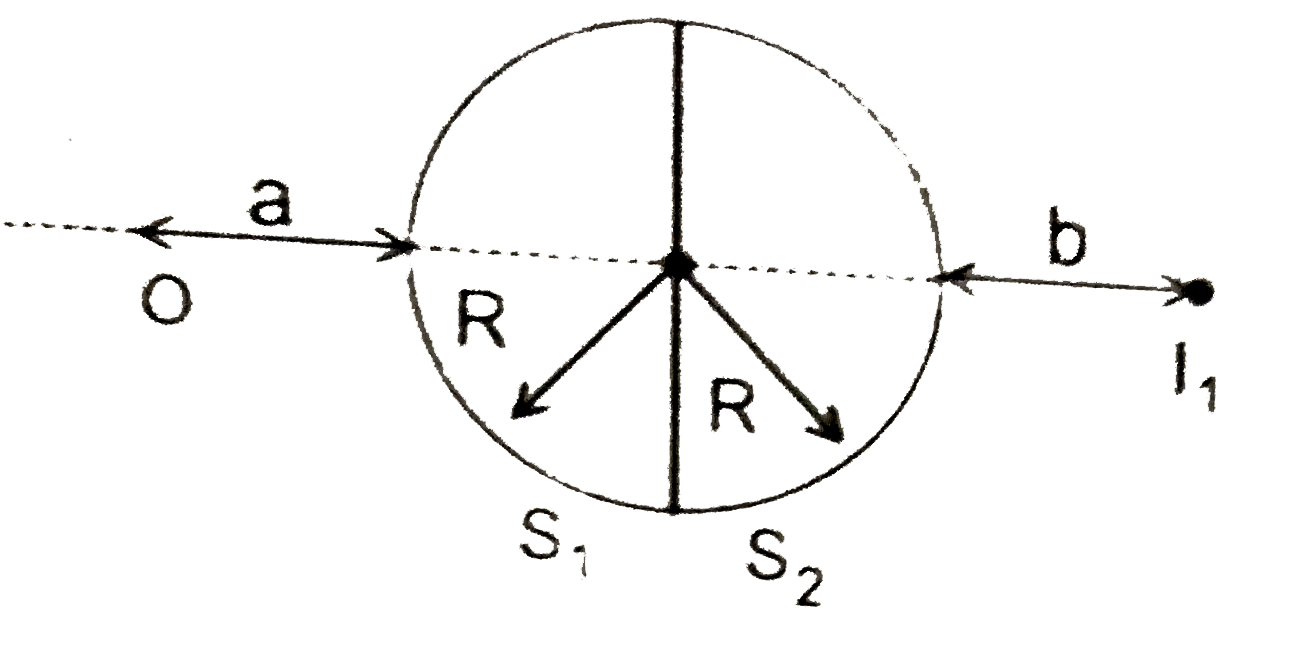
An object O kept a distance a=10cm from the surface of a glass hemisphere S1 of radius R. S2 is another glass hemisphere kept side by side of same radius R=5cm as shown in figure l1 is the image formed due to object O (b=6cm). Now if S2 is removed and S1 is kept at the same place and its flat surface is silvered then find te distance l1l2 (where l1 si image formed by silvered S1 only) (μ glass =3/2)
View SolutionFind the surface area of a sphere of radius:(i) 10.5 cm (ii) 5.6 cm (iii) 14 cm
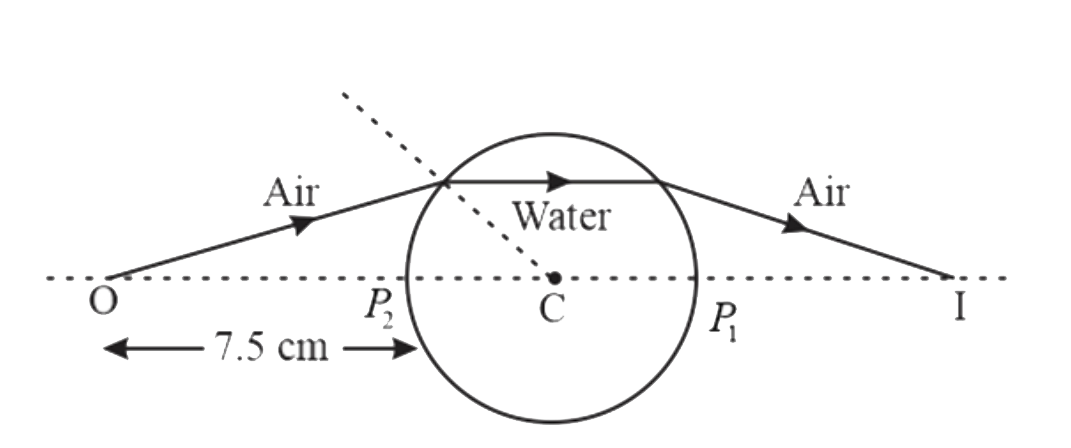
View SolutionA thin walled glass sphere of radius 2.5 cm is filled with water. An object (O) is placed at 7.5 cm from the effect of glass wall, at what distance the image (I) of the object , measured from the centre of sphere is formed ? (refractive index of water = 1.333)
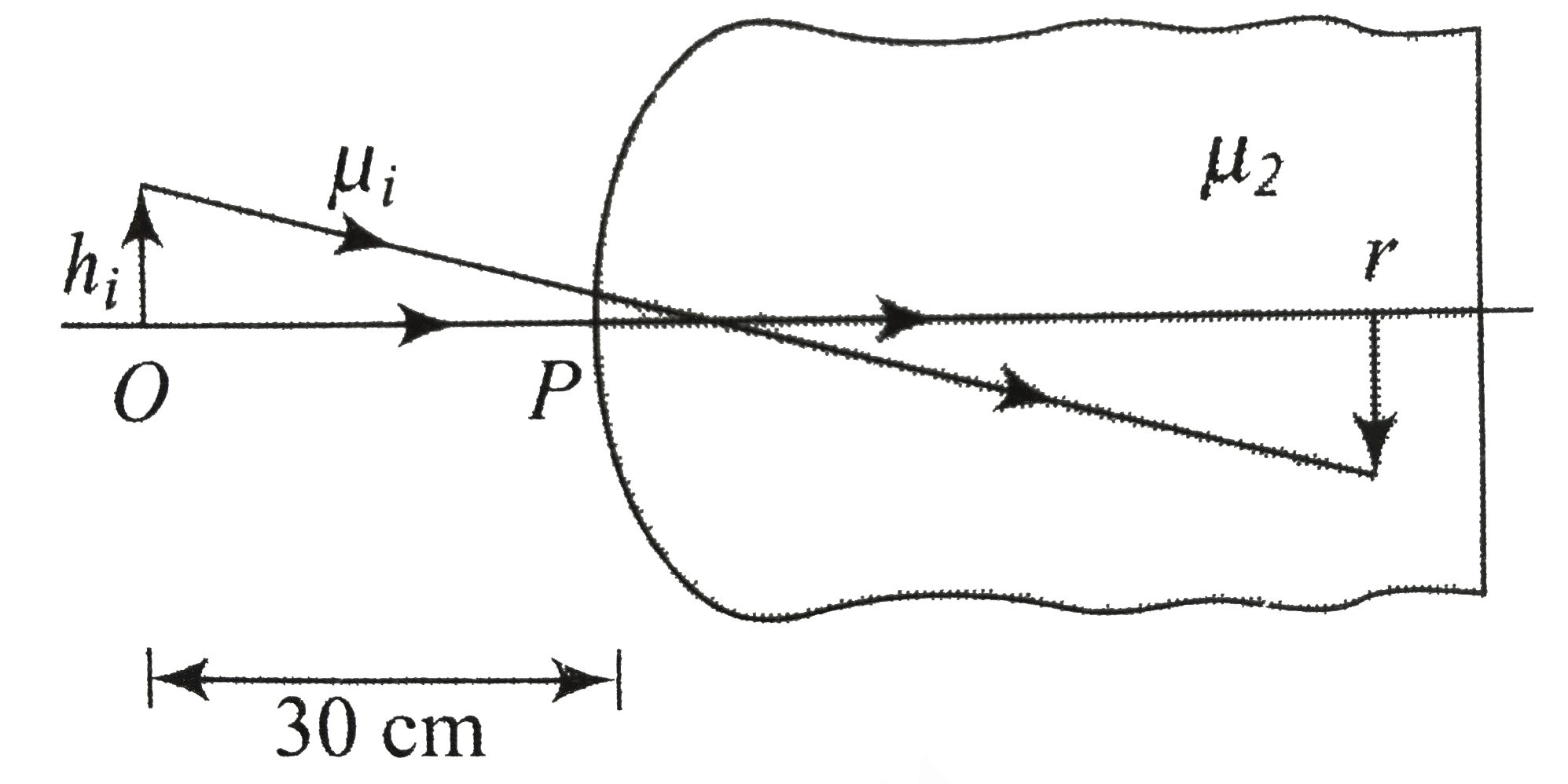
View SolutionA small object of height 0.5 cm is placed in front of a convex surface of glass (μ−1.5) of radius of curvature 10cm. Find the height of the image formed in glass.
View SolutionThe figure ,shows a transparent sphere of radiusRand refractive indexμ.An object Ois placed at a distance x from the pole of the first surface so that a real image is formed at the pole of the exactly opposite surface.
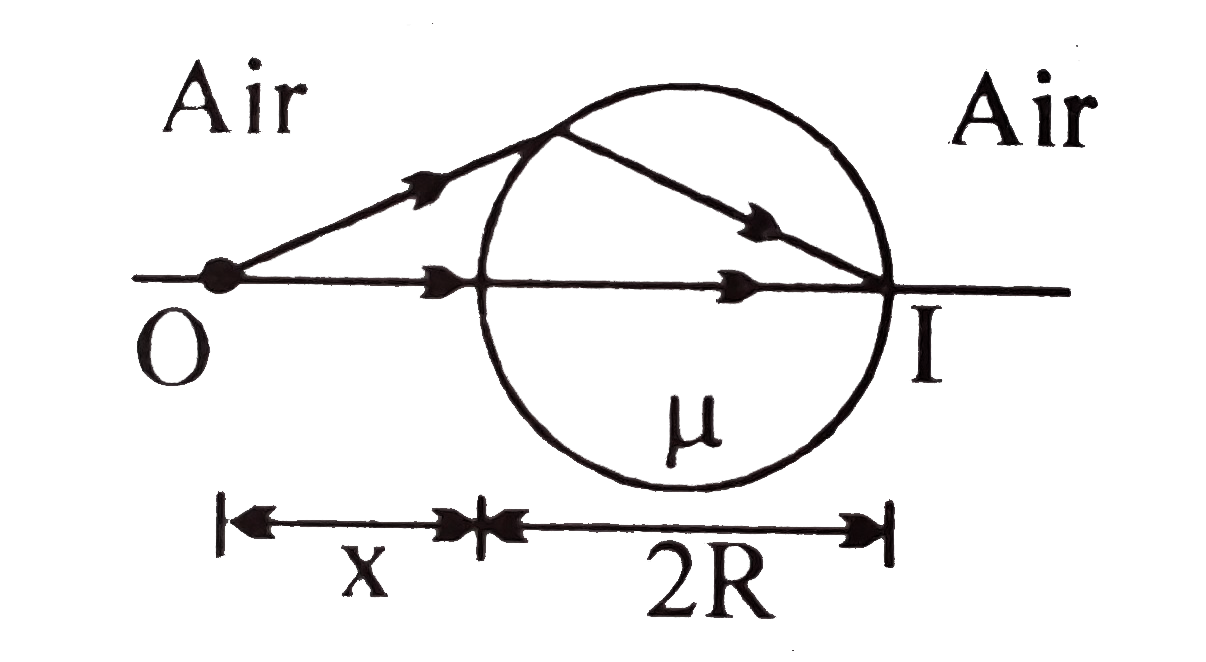 if an object is Placed at a distanceR from the pole of first surface ,then the real image is formed at a distance Rfrom thepole of the second surface.The refractive indexμ of the spher is given by
if an object is Placed at a distanceR from the pole of first surface ,then the real image is formed at a distance Rfrom thepole of the second surface.The refractive indexμ of the spher is given by
View Solution