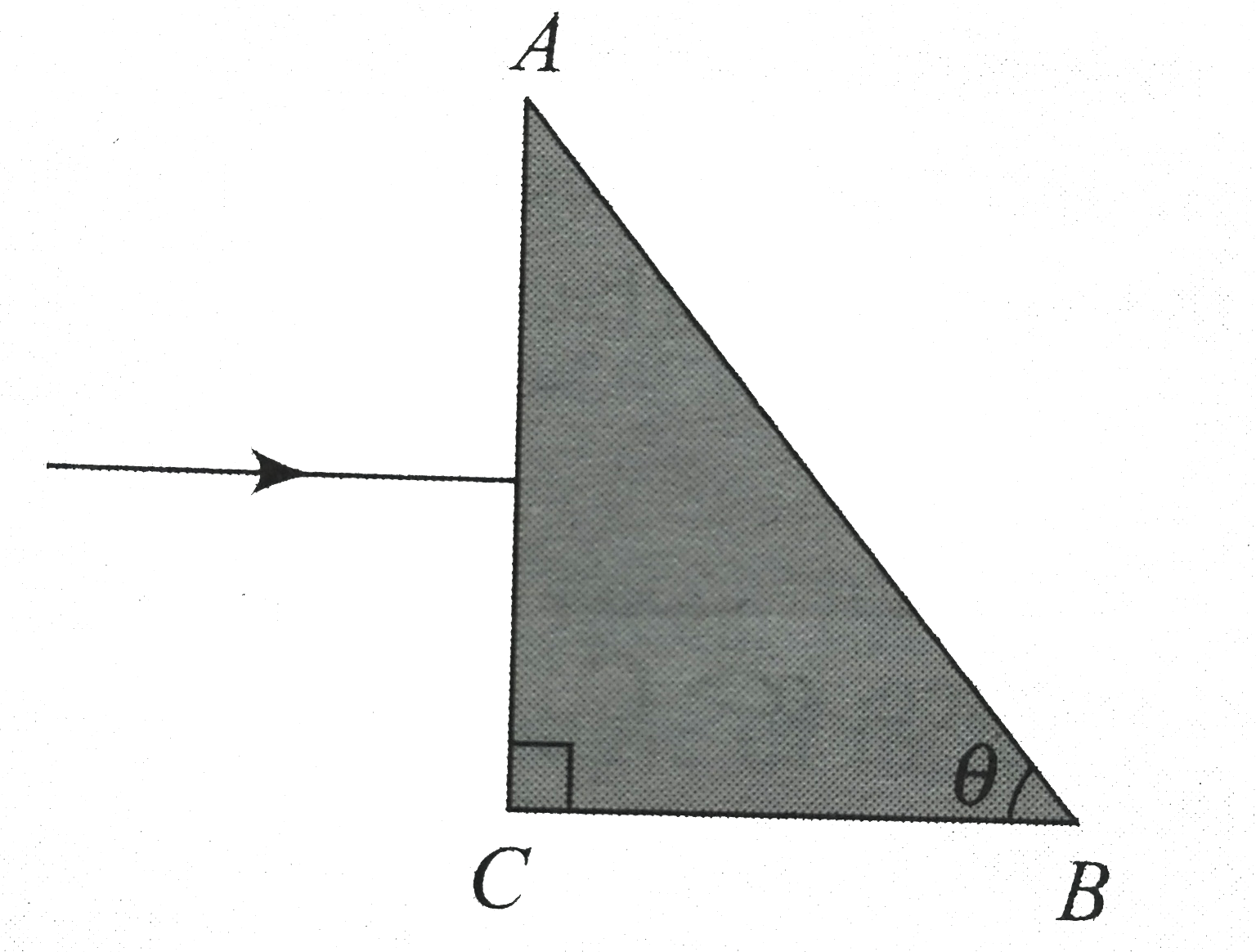
In the figure ABC is the cross-section of a right angled prism and BCDE is the cross-section of a glass slab. The value of theta so that light incident normally on the face AB does not cross the face BC is

The correct Answer is:A
Now,
or
or
From Eq. (i), we see that
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Single Correct Option3videos
REFRACTION OF LIGHT
- BOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - more than one correct option1videos
REFRACTION OF LIGHT
- BOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Subjective Questions8videos
REFRACTION OF LIGHT
- BOOK - DC PANDEY ENGLISHCHAPTER - REFLECTION OF LIGHTEXERCISE - Subjective9videos
REFLECTION OF LIGHT
- BOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORSEXERCISE - Subjective12videos
SEMICONDUCTORS
Similar Questions
What should be the value of angle θ so that light entering normally through the surface AC of a prism (n=3/2) does not cross the second refracting surface AB?
 View Solution
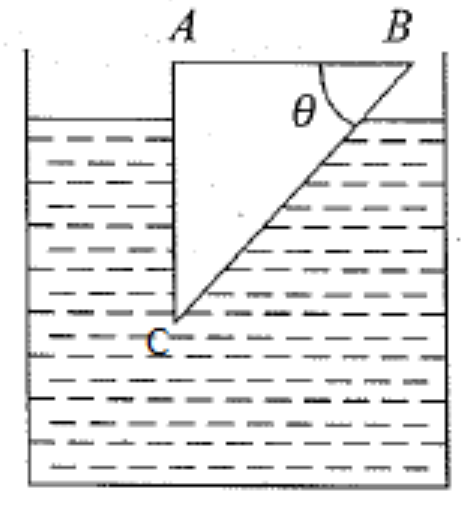
View SolutionA glass prism of refractive index 1.5 is immersed in water of refractive index 4/3. A light ray incident normally on face AB is totally reflected at face AC if
 View Solution
View SolutionAn equilateral triangular prism is made of glass (μ=1.5). A ray of light is incident normally on one of the faces. The angle between the incident and emergent ray is
View SolutionA glass prism of refractive index 1.5 is immersed in water (refractive index 4/3) . A light beam normally on the face AB is totally reflected to reach on the face BC if
 View Solution
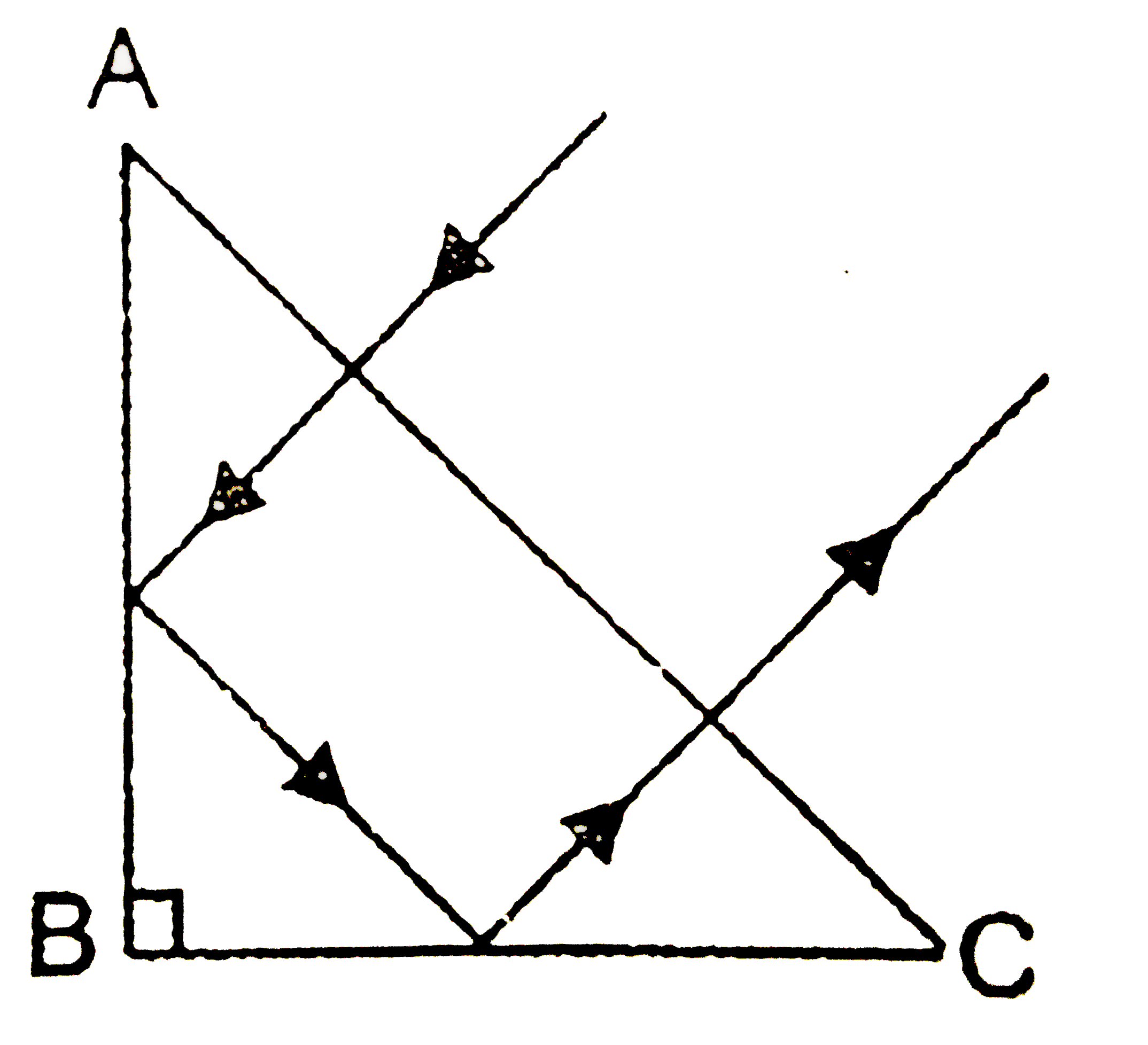
View SolutionAt what values fo the refractive index of a recantangular prism can a ray travel as shwon in figure. The section of the prism is an isoseless triangle and the ray in normally incident onto the face AC.
 View Solution
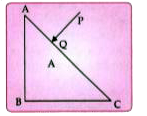
View SolutionA ray of light PQ is incident normally on the hypotenuse of a right angled prism ABC as shown in the diagram given below:
What is the value of the angle of deviation of the ray ?
 View Solution
View SolutionA light ray is incident normally on the face AB of a right-angled prism ABC(μ==1.50) as shown in figure. What is the largest angle ϕ for which the light ray is totally reflected at the surface AC ?
 View Solution
View Solution(i) Plot a graph to show variation of the angle of deviation as function of angle of incidence for light passing through a prism. Derive an expression for refractive index of the prism in terms of angle of minimum deviation and angles of prism.
(ii) What is dispersion of light ? Why is its cause ?
(iii) A ray of light incident normally on one face of a right isosceles prism is totally reflected as shown in fig . what must be the minimum value of refractive index of glass ? Give relevant calculations
 View Solution
View Solution(a) Show using a proper diagram how unpolarised light can be linearly polarised by reflection from a transparent glass surface.
(b) The figure shows a ray of light falling normally on. the face AB of an equilateral glass prism having refractive index 3/2, placed A in water ofrefractive index 4/3. Will this ray suffer total internal reflection on striking the face AC? Justify your answer.View SolutionA ray of light is incident normally on one face of an equilateral prism of refractive index 1.5. The angle of deviation is: (Given sin−1.23=42∘)
View Solution
