A sphere (μ=43) of radius 1m has a small cavity of diameter 1cm at its centre. An observer who is looking at it from tight, sees the magnification of diameter of the cavity as
43
34
1
0.5
The correct Answer is:A
To solve the problem of finding the magnification of the diameter of the cavity in a sphere with a refractive index of μ=43, we can follow these steps:
Step 1: Understand the Geometry
We have a sphere of radius R=1m with a small cavity at its center. The diameter of the cavity is d=1cm=0.01m.
Step 2: Identify the Relevant Parameters
The refractive index of the sphere is given as μ=43. The observer is looking at the cavity from the side, which means we need to consider how light rays will behave as they pass through the sphere.
Step 3: Determine the Object and Image Distances
Since the cavity is at the center of the sphere, any light rays coming from the cavity will emerge normally from the surface of the sphere. Therefore, the object distance u and the image distance v can be considered equal in this case.
Given that the radius of the sphere is 1m, we can set:
- u=−1m (the negative sign indicates that the object is on the same side as the incoming light)
- v=−1m (similarly, the image distance is also negative)
Step 4: Calculate the Magnification
The magnification M is given by the formula:
M=μ1μ2⋅vu
Where:
- μ1 is the refractive index of the medium from which the light is coming (air, which is approximately 1)
- μ2 is the refractive index of the sphere (43)
Substituting the values:
- μ1=1
- μ2=43
Now substituting into the magnification formula:
M=143⋅−1−1=34
Step 5: Final Magnification Calculation
Since v and u are equal and cancel each other out, we can simplify the magnification to:
M=34⋅1=43
Conclusion
Thus, the magnification of the diameter of the cavity as seen by the observer is 43.
---
We have a sphere of radius R=1m with a small cavity at its center. The diameter of the cavity is d=1cm=0.01m.
The refractive index of the sphere is given as μ=43. The observer is looking at the cavity from the side, which means we need to consider how light rays will behave as they pass through the sphere.
Since the cavity is at the center of the sphere, any light rays coming from the cavity will emerge normally from the surface of the sphere. Therefore, the object distance u and the image distance v can be considered equal in this case.
- u=−1m (the negative sign indicates that the object is on the same side as the incoming light)
- v=−1m (similarly, the image distance is also negative)
The magnification M is given by the formula:
M=μ1μ2⋅vu
Where:
- μ1 is the refractive index of the medium from which the light is coming (air, which is approximately 1)
- μ2 is the refractive index of the sphere (43)
- μ1=1
- μ2=43
M=143⋅−1−1=34
Since v and u are equal and cancel each other out, we can simplify the magnification to:
M=34⋅1=43
Thus, the magnification of the diameter of the cavity as seen by the observer is 43.
Topper's Solved these Questions
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Single Correct Option
3 Videos
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - more than one correct option
1 Video
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Subjective Questions
8 Videos
- REFLECTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFLECTION OF LIGHTEXERCISE - Subjective
9 Videos
- SEMICONDUCTORSBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORSEXERCISE - Subjective
12 Videos
Similar Questions
A glass sphere of radius 5 cm has a small bubble at a distance 2 cm from its centre.The bubble is viewed along a Diameter of the sphere from the side on which it lies.How far from the surface will it appear.Refractive index of glass is 1.5
2.5 cm behind the surface
View SolutionA glass sphere (μ=1.5) of radius 20 cm has a small air bubble 4 cm below its centre. The sphere is viewed from outside and along a vertical line through the bubble. The apparent depth of the bubble below the surface of sphere is (in cm)
View SolutionA uniform solid sphere of radius R has a cavity of radius 1m cut from it if centre of mass of the system lies at the periphery of the cavity then

View SolutionA uniform solid sphere of radius R has a cavity of radius 1m cut from it if centre of mass of the system lies at the periphery of the cavity then

View SolutionA conducting sphere of radius R2 has a spherical cavity of radius R1 which is non concentric with the sphere. A point charge q1 is placed at a distance r from the centre of the cavity. For this arrangement, answer the following questions.
If q1 is shifted to the centre of the cavity, then: (choose the correct alternative)
View SolutionA conducting sphere of radius R2 has a spherical cavity of radius R1 which is non concentric with the sphere. A point charge q1 is placed at a distance r from the centre of the cavity. For this arrangement, answer the following questions.
Electric potential at the centre of the cavity will be :
View SolutionA particle of mass m is placed at a distance of 4R from the centre of a huge uniform sphere of mass M and radius R. A spherical cavity of diameter R is made in the sphere as shown in the figure. If the gravitational interaction potential energy of the system of mass m and the remaining sphere after making the cavity is λGMm28R. Find the value of λ
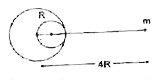
View SolutionA sphere of radius 2R and mas M has a spherical cavity of radius R as shown in the figure. Find the value of gravitational field at a point P at a distance of 6R from centre of the sphere.

View SolutionA glass sphere of (μ=1.5) with a radius of 15.0cm has a tiny air bubble 5cm above its centre. The sphere is viewed looking down along the extended radius containing the bubble. What is the apparent depth of the bubble below the surface of the sphere?
View SolutionA solid sphere of mass M and radius R has a spherical cavity of radius R/2 such that the centre of cavity is at a distance R/2 from the centre of the sphere. A point mass m is placed inside the cavity at a distanace R/4 from the centre of sphere. The gravitational force on mass m is
View Solution
