A particle A is projected with an initial velocity of $60 m / s$ at an angle $30^{\circ}$ to the horizontal. At the same time a second particle B is projected in opposite direction with initial speed of $50 m / s$ from a point at a distance of 100 m from A. If the particles collide in air, find (a)the angle of projection $α$ of particle B, (b) time when the collision takes place and (c) the distance of P from A, where collision occurs. $(g = 10 m / s^{2})$

Video Solution

Text Solution

Verified by Experts

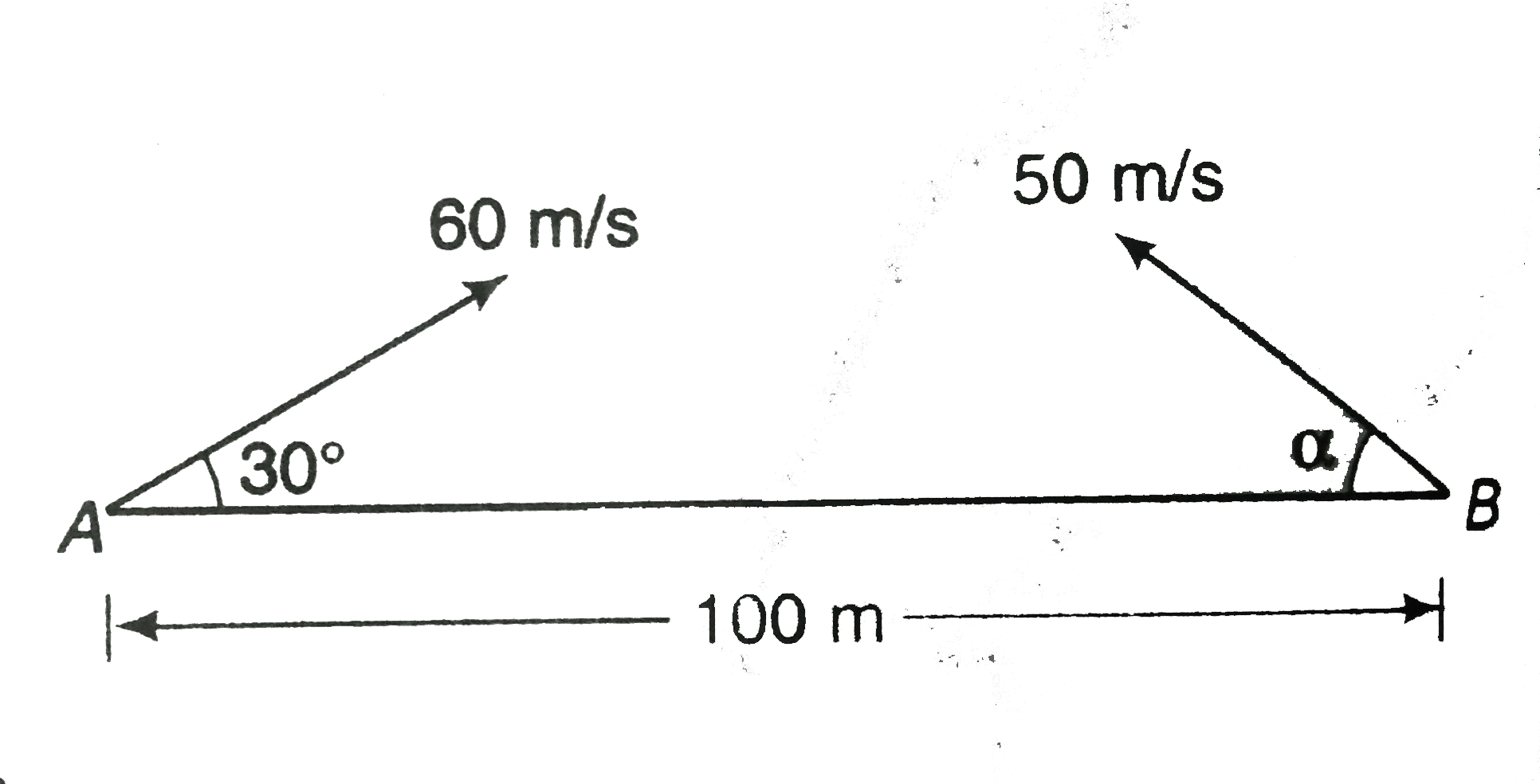
The correct Answer is:A, B, C, D

(a) Taking x and y-directions as shown in figure.
Here, $a_{A} = - g ˆ j$
$a_{B} = - g ˆ j$
$u_{A x} = 60 {cos 30}^{\circ} = 30 \sqrt{3} m / s$
$u_{A y} = 60 {sin 30}^{\circ} = 30 m / s$
$u_{B x} = - 50 cos α$
and $u_{B y} = 50 sin α$
Relative acceleration between the two is zero as $a_{A} = a_{B}$ . Hence, the relative motion
between the two is uniform. It can be assumed that B is at rest and A is moving with $u_{A B}$ .
Hence, the two particles will collide, if $u_{A B}$ is along AB. This is possible only when
$u_{A y} = u_{B y}$
i.e. component of relative velocity along y-axis should be zero.
or $30 = 50 sin α$
$∴ α = {sin}^{- 1} (3 / 5) = 37^{\circ}$
(b) Now, $| u_{A B} | = u_{A x} - u_{B x}$
$= (30 \sqrt{3} + 50 cos α) m / s$
$= (30 \sqrt{3} + 50 \times \frac{4}{5}) m / s$
$= (30 \sqrt{3} + 40) m / s$
Therefore, time of collision is
$t = \frac{A B}{| u_{A B} |} = \frac{100}{30 \sqrt{3} + 40}$
or $t = 1.09 s$
(c) Distance of point P from A where collision takes place is
$d = \sqrt{{(u_{A x} t)}_{A x}^{2} + {(u_{A y} t - \frac{1}{2} >^{2})}_{A y}^{2}}$
$= \sqrt{{(30 \sqrt{3} \times 1.09)}^{2} + {(30 \times 1.09 - \frac{1}{2} \times 10 \times 1.09} {\times 1.09)}^{2}}$
or $d = 62.64 m$ .

|

Updated on:21/07/2023

Class 11 PHYSICS PROJECTILE MOTION

Topper's Solved these Questions

Similar Questions

DC PANDEY ENGLISH-PROJECTILE MOTION-Level - 2 Subjective

Home
Ask Doubt
Profile