A particle is projected with a velocity 10 m//s at an angle 37∘ to the horizontal. Find the location at which the particle is at a height 1 m from point of projection.
The correct Answer is:1.6 m, 8 m.
To solve the problem of finding the location of a particle projected with a velocity of 10 m/s at an angle of 37 degrees to the horizontal when it reaches a height of 1 meter, we can follow these steps:
Step 1: Identify the components of the initial velocity
The initial velocity u can be resolved into horizontal and vertical components:
- ux=ucosθ
- uy=usinθ
Given:
- u=10m/s
- θ=37∘
Using trigonometric values:
- cos37∘=45
- sin37∘=35
Calculating the components:
ux=10⋅45=8m/s
uy=10⋅35=6m/s
Step 2: Write the equation for vertical motion
The vertical position y of the particle as a function of time t is given by:
y=uyt−12gt2
where g is the acceleration due to gravity (approximately 10m/s2).
Step 3: Set the height to 1 meter
We need to find the time when the particle reaches a height of 1 meter:
1=6t−12⋅10t2
This simplifies to:
1=6t−5t2
Rearranging gives:
5t2−6t+1=0
Step 4: Solve the quadratic equation
Using the quadratic formula t=−b±√b2−4ac2a:
Here, a=5, b=−6, and c=1.
t=6±√(−6)2−4⋅5⋅12⋅5
t=6±√36−2010
t=6±√1610
t=6±410
Calculating the two possible values for t:
1. t=1010=1s
2. t=210=0.2s
Step 5: Find the horizontal distance at these times
The horizontal distance x is given by:
x=uxt
Calculating for both times:
1. For t=1s:
x=8⋅1=8m
2. For t=0.2s:
x=8⋅0.2=1.6m
Final Result
The particle reaches a height of 1 meter at two locations:
- At x=8m (on the way up)
- At x=1.6m (on the way down)
The initial velocity u can be resolved into horizontal and vertical components:
- ux=ucosθ
- uy=usinθ
- u=10m/s
- θ=37∘
- cos37∘=45
- sin37∘=35
ux=10⋅45=8m/s
uy=10⋅35=6m/s
The vertical position y of the particle as a function of time t is given by:
y=uyt−12gt2
where g is the acceleration due to gravity (approximately 10m/s2).
We need to find the time when the particle reaches a height of 1 meter:
1=6t−12⋅10t2
This simplifies to:
1=6t−5t2
Rearranging gives:
5t2−6t+1=0
Using the quadratic formula t=−b±√b2−4ac2a:
Here, a=5, b=−6, and c=1.
t=6±√(−6)2−4⋅5⋅12⋅5
t=6±√36−2010
t=6±√1610
t=6±410
Calculating the two possible values for t:
1. t=1010=1s
2. t=210=0.2s
The horizontal distance x is given by:
x=uxt
Calculating for both times:
1. For t=1s:
x=8⋅1=8m
2. For t=0.2s:
x=8⋅0.2=1.6m
The particle reaches a height of 1 meter at two locations:
- At x=8m (on the way up)
- At x=1.6m (on the way down)
Topper's Solved these Questions
- KINEMATICS-2DBOOK - ALLENCHAPTER - KINEMATICS-2DEXERCISE - Exercise (S-1)
30 Videos
- KINEMATICS-2DBOOK - ALLENCHAPTER - KINEMATICS-2DEXERCISE - Exercise (S-2)
15 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - EXERCISE 4
9 Videos
- MOTION IN A PALNEBOOK - ALLENCHAPTER - MOTION IN A PALNEEXERCISE - SOLVED EXAMPLE
28 Videos
Similar Questions
A particle A is projected with an initial velocity of 60m/s at an angle 30∘ to the horizontal. At the same time a second particle B is projected in opposite direction with initial speed of 50m/s from a point at a distance of 100 m from A. If the particles collide in air, find (a)the angle of projection α of particle B, (b) time when the collision takes place and (c) the distance of P from A, where collision occurs. (g=10m/s2)
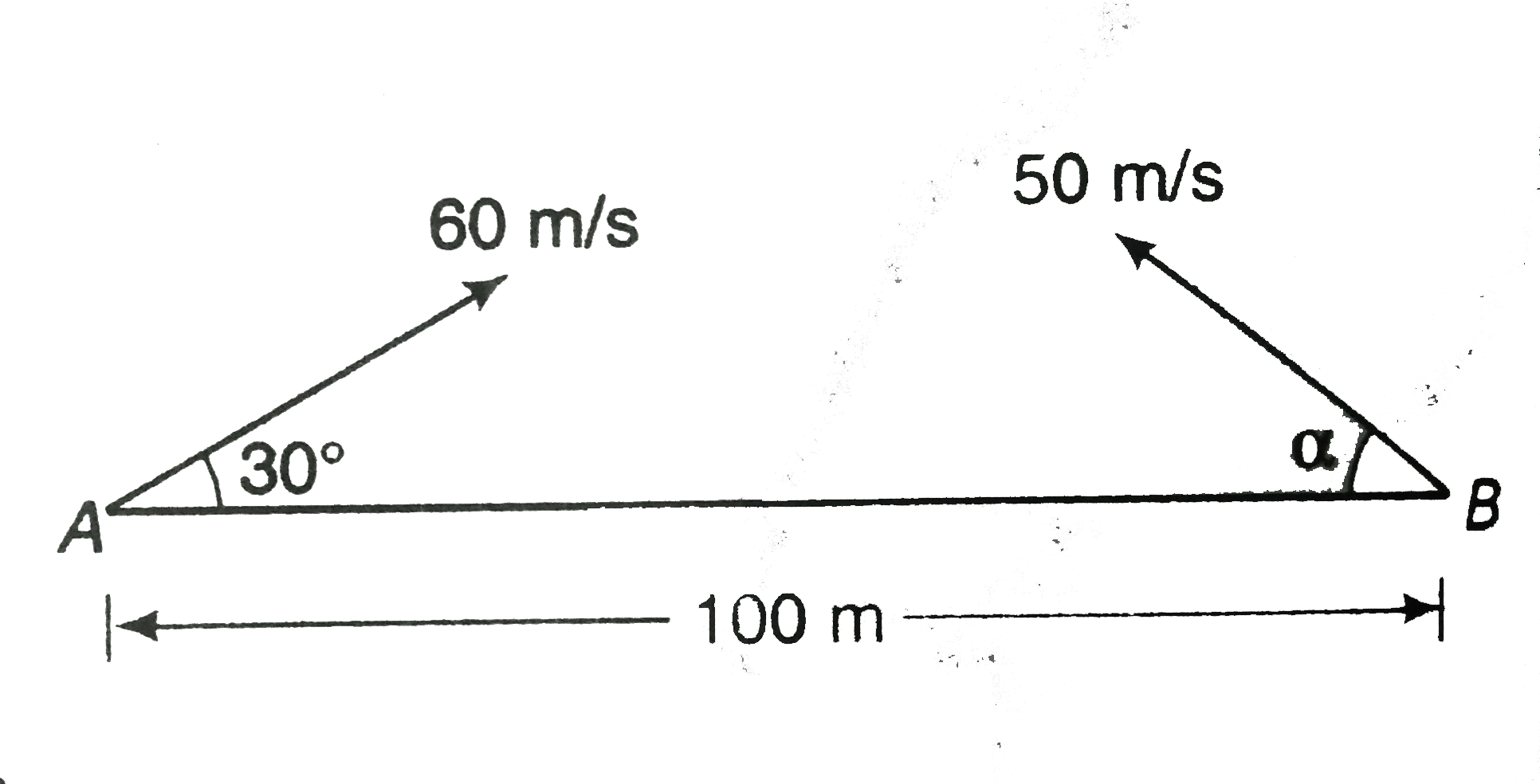
View SolutionA particle is projected with speed 10m/s at angle 60∘ with the horizontal. Then the time after which its speed becomes half of initial.
View SolutionA particle is projected with velocity of 10ms at an angle of 15° with horizontal.The horizontal range will be(g=10ms2)
View SolutionA particle is projected with a speed u at an angle θ to the horizontal. Find the radius of curvature.
At the point where the particle is at a highest half of the maximum height H attained by it.
View SolutionA particle is projected with a speed of 10m/s at an angle 37∘ from horizontal, angular speed to particle with respect to point of projection at t=0.5sec. Is (16X61) rad/sec then calculate X :
View SolutionA particle of mass m is projected with speed u at an angle θ with the horizontal. Find the torque of the weight of the particle about the point of projection when the particle is at the highest point.
View SolutionA particle of mass m is projected with speed u at an angle θ with the horizontal. Find the torque of the weight of the particle about the point of projection when the particle is at the highest point.
View SolutionA particle of mass m is projected with a velocity v at an angle of θ with horizontal. The angular momentum of the particle at the highest point of its trajectory is equal to :
View SolutionA particle is projected upwards with a velocity of 100m/s at an angle of 60∘ with the vertical. Find the time when the particle will move perpendicular to its initial direction, taking g=10m/s2.
View SolutionA ball is projected with 20√2m/s at angle 45∘ with horizontal.The angular velocity of the particle at highest point of its journey about point of projection is
View Solution
