The Speed Of a particle moving in a plane is equal to the magnitude of its instantaneous velocity, v=∣vl=√v2x+v2y.
(a) Show that the rate of change of the speed is dvdt=vxax+vyay√v2x+v2y.
(b) Show that the rate of change of speed can be expressed as dvdt is equal toat the component of a that is parallelto v.
The correct Answer is:A
To solve the problem step by step, we will address both parts (a) and (b) of the question regarding the rate of change of speed of a particle moving in a plane.
Part (a)
1. Understanding Speed and Velocity:
The speed v of a particle is given by the magnitude of its instantaneous velocity:
v=|v|=√v2x+v2y
where vx and vy are the components of the velocity in the x and y directions, respectively.
2. Differentiating Speed with Respect to Time:
To find the rate of change of speed, we differentiate v with respect to time t:
dvdt=ddt(√v2x+v2y)
3. Applying the Chain Rule:
Using the chain rule for differentiation:
dvdt=12√v2x+v2y⋅ddt(v2x+v2y)
4. Differentiating the Inside Function:
Now, we differentiate v2x+v2y:
ddt(v2x+v2y)=2vxdvxdt+2vydvydt
Here, dvxdt=ax and dvydt=ay are the components of acceleration.
5. Substituting Back:
Substituting this back into our equation, we have:
dvdt=12√v2x+v2y⋅(2vxax+2vyay)
6. Simplifying:
Simplifying gives:
dvdt=vxax+vyay√v2x+v2y
Thus, we have shown that:
dvdt=vxax+vyay√v2x+v2y
Part (b)
1. Understanding the Components of Acceleration:
The acceleration vector a can be expressed in terms of its components:
a=ax^i+ay^j
2. Finding the Component of Acceleration Parallel to Velocity:
The component of acceleration at that is parallel to the velocity v can be found using the dot product:
at=a⋅v|v|
3. Calculating the Dot Product:
The dot product a⋅v is:
a⋅v=axvx+ayvy
4. Magnitude of Velocity:
The magnitude of the velocity is:
|v|=√v2x+v2y
5. Substituting into the Expression for at:
Therefore, we can express at as:
at=axvx+ayvy√v2x+v2y
6. Relating dvdt and at:
From part (a), we found that:
dvdt=vxax+vyay√v2x+v2y
Thus, we can conclude that:
dvdt=at
Final Results
- For part (a):
dvdt=vxax+vyay√v2x+v2y
- For part (b):
dvdt=at
The speed v of a particle is given by the magnitude of its instantaneous velocity:
v=|v|=√v2x+v2y
where vx and vy are the components of the velocity in the x and y directions, respectively.
To find the rate of change of speed, we differentiate v with respect to time t:
dvdt=ddt(√v2x+v2y)
Using the chain rule for differentiation:
dvdt=12√v2x+v2y⋅ddt(v2x+v2y)
Now, we differentiate v2x+v2y:
ddt(v2x+v2y)=2vxdvxdt+2vydvydt
Here, dvxdt=ax and dvydt=ay are the components of acceleration.
Substituting this back into our equation, we have:
dvdt=12√v2x+v2y⋅(2vxax+2vyay)
Simplifying gives:
dvdt=vxax+vyay√v2x+v2y
dvdt=vxax+vyay√v2x+v2y
The acceleration vector a can be expressed in terms of its components:
a=ax^i+ay^j
The component of acceleration at that is parallel to the velocity v can be found using the dot product:
at=a⋅v|v|
The dot product a⋅v is:
a⋅v=axvx+ayvy
The magnitude of the velocity is:
|v|=√v2x+v2y
Therefore, we can express at as:
at=axvx+ayvy√v2x+v2y
From part (a), we found that:
dvdt=vxax+vyay√v2x+v2y
Thus, we can conclude that:
dvdt=at
- For part (a):
dvdt=vxax+vyay√v2x+v2y
- For part (b):
dvdt=at
Topper's Solved these Questions
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - SCQ_TYPE
39 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - MCQ_TYPE
23 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Comprehension
7 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises
45 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
Similar Questions
Let v and a be the instantaneous velocity and acceleration of a particle moving in a plane. Then rate of change of speed dvdt of the particle is equal to
View SolutionA particle is moving on a circular path with constant speed v. The magnitude of the change in its velocity after it has described an angle of 90∘ is :
View Solution
Knowledge Check
A particle is moving on a circular path with a constant speed v. Its change of velocity as it moves from A to B is:
 A2vsin(2θ)BzeroC2vsin(θ2)D2vcos(θ2)
A2vsin(2θ)BzeroC2vsin(θ2)D2vcos(θ2)A particle moves in the x-y plane with velocity vx=8t−2andvy=2. If it passes through the point x =14 and y = 4 at t =2s the equation of the path is
A1. x=y3−y2+2B2. x=y2−y+2C3. x=y2−3y+2D4. x=y3−2y2+2
A particle is moving with constant speed v in xy plane as shown in figure. The magnitude of its angular velocity about point O is

View SolutionA particle id moving in xy - plane with y=x/2andvx=4−2t. Choose the correct options.
View SolutionA particle is moving along x-axis. Its velocity v with x co-ordinate is varying as v=√x. Then
View SolutionA particle is moving in a circle of radius r with speed v as shown in the figure. The magnitude of change in velocity in moving from P to Q is
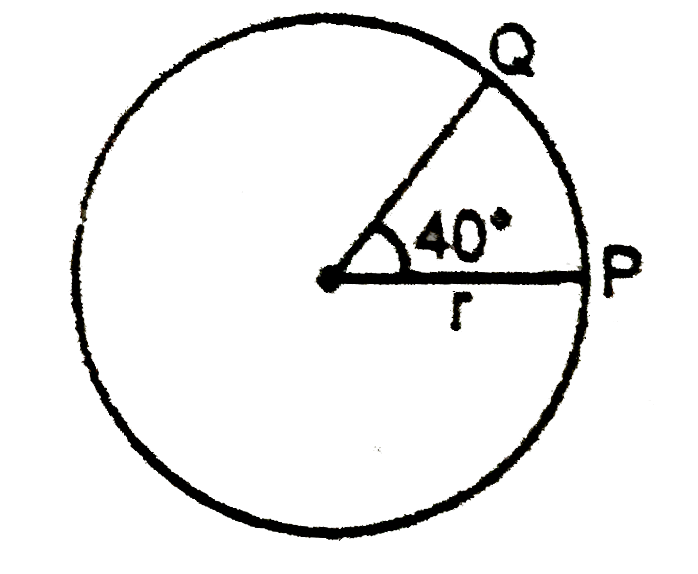
View SolutionA particle is moving in a circle of radius r with speed v as shown in the figure. The magnitude of change in velocity in moving from P to Q is
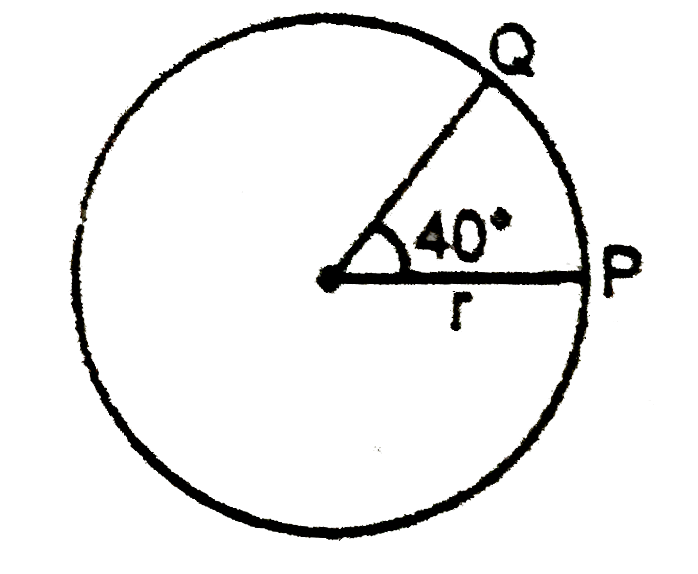
View SolutionA particle moving with a speed v changes direction by an angle θ, without change in speed.
View Solution
