A particle id moving in xy - plane with y=x/2andvx=4−2t. Choose the correct options.
Initial velocities in x and y directions are negative.
Initial velocities in x and y directions are positive.
Motion is first retarded, then accelerated.
Motion is first accelerated, then retarded.
The correct Answer is:B, C
To solve the problem step-by-step, we will analyze the motion of the particle in the xy-plane given the equations provided.
Step 1: Understand the given equations
We have two equations:
1. The relationship between y and x:
y=x2
2. The velocity in the x-direction:
vx=4−2t
Step 2: Find the relationship between velocities
From the equation y=x2, we can differentiate both sides with respect to time t:
dydt=12dxdt
Here, dxdt is the velocity in the x-direction (vx) and dydt is the velocity in the y-direction (vy). Thus, we can write:
vy=12vx
Step 3: Substitute the expression for vx
Substituting vx=4−2t into the equation for vy:
vy=12(4−2t)=2−t
Step 4: Analyze initial velocities
At time t=0:
- For vx:
vx=4−2(0)=4m/s
- For vy:
vy=2−0=2m/s
Thus, the initial velocities are:
- ux=4m/s
- uy=2m/s
Step 5: Determine accelerations
Next, we will find the acceleration in both x and y directions by differentiating the velocities with respect to time.
1. For vx:
ax=dvxdt=d(4−2t)dt=−2m/s2
2. For vy:
ay=dvydt=d(2−t)dt=−1m/s2
Step 6: Analyze the motion
Both accelerations are negative, indicating that the motion is retarding in both x and y directions. Since both accelerations are constant and negative, the motion is uniformly retarded.
Step 7: Conclusion
Based on the analysis, we can conclude:
- The initial velocities are positive.
- The motion is uniformly retarded in both directions.
Thus, the correct option is option 2.
We have two equations:
1. The relationship between y and x:
y=x2
2. The velocity in the x-direction:
vx=4−2t
From the equation y=x2, we can differentiate both sides with respect to time t:
dydt=12dxdt
Here, dxdt is the velocity in the x-direction (vx) and dydt is the velocity in the y-direction (vy). Thus, we can write:
vy=12vx
Substituting vx=4−2t into the equation for vy:
vy=12(4−2t)=2−t
At time t=0:
- For vx:
vx=4−2(0)=4m/s
- For vy:
vy=2−0=2m/s
- ux=4m/s
- uy=2m/s
Next, we will find the acceleration in both x and y directions by differentiating the velocities with respect to time.
ax=dvxdt=d(4−2t)dt=−2m/s2
2. For vy:
ay=dvydt=d(2−t)dt=−1m/s2
Both accelerations are negative, indicating that the motion is retarding in both x and y directions. Since both accelerations are constant and negative, the motion is uniformly retarded.
Based on the analysis, we can conclude:
- The initial velocities are positive.
- The motion is uniformly retarded in both directions.
Topper's Solved these Questions
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise Assertion - Reasoning
5 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise Comprehension
21 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise Single Correct
76 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Integer
9 Videos
- KINETIC THEORY OF GASESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASESEXERCISE - Compression
2 Videos
Similar Questions
Knowledge Check
A particle moves in the x-y plane with velocity vx=8t−2andvy=2. If it passes through the point x =14 and y = 4 at t =2s the equation of the path is
A1. x=y3−y2+2B2. x=y2−y+2C3. x=y2−3y+2D4. x=y3−2y2+2
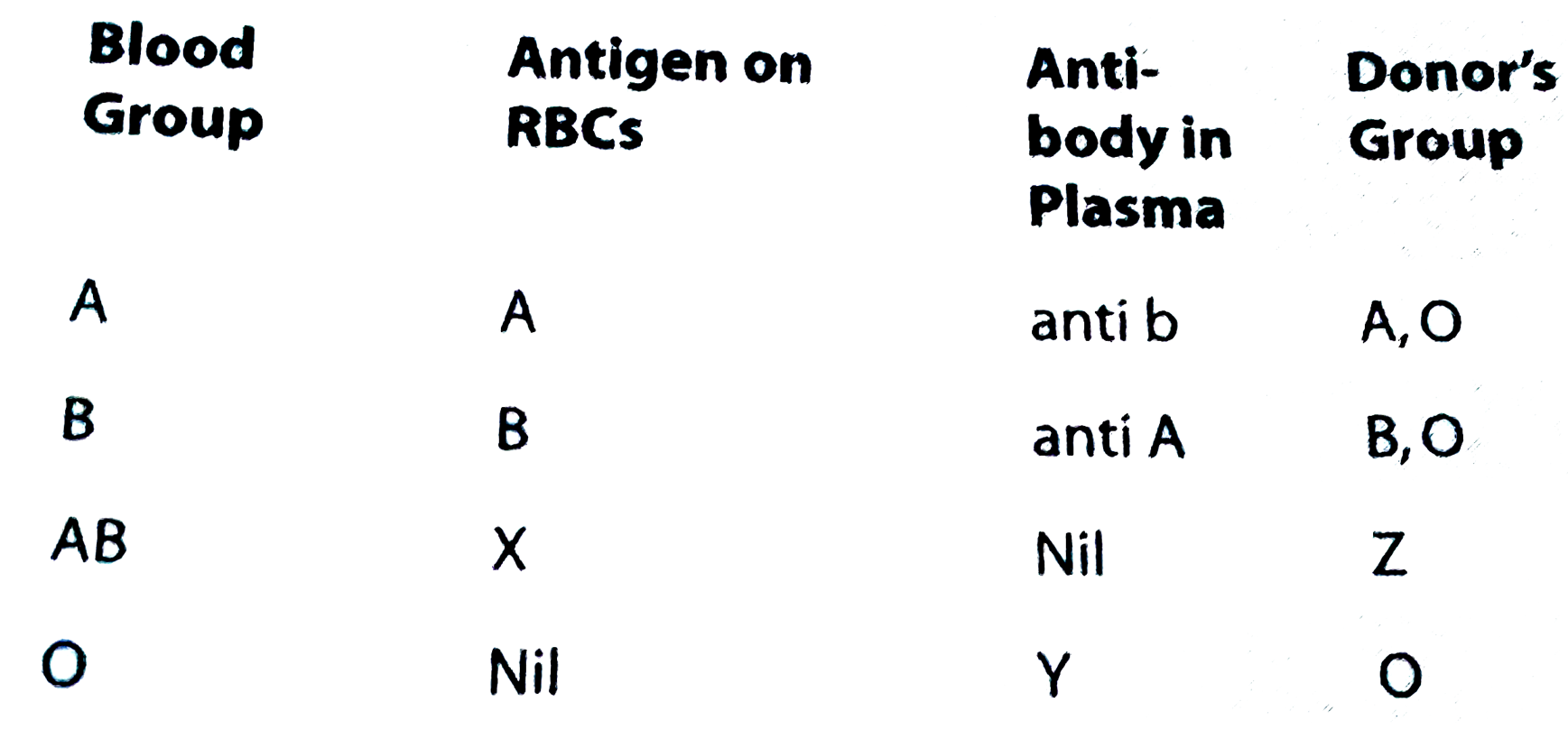
Choose the correct option for X, Y and Z.
View SolutionA particle moves in the x-y plane with velocity vx=8t−2andvy=2. If it passes through the point x=14andy=4att=2s, the equation of the path is
View SolutionA particle moves in the x-y plane with velocity vx=8t−2andvy=2. If it passes through the point x=14andy=4 at t = 2 s, the equation of the path is
View SolutionThe coordinates of a particle moving in XY-plane vary with time as x=4t2,y=2t. The locus of the particle is a : -
View SolutionThe coordinates of a particle moving in XY-plane very with time as x=4t2,y=2t. The locus of the particle is
View SolutionThe co-ordinates of a particle moving in xy-plane vary with time as x=at2,y=bt. The locus of the particle is :
View SolutionA particle is moving in x-y plane with its x and y co-ordinates varying with time as, x=2tandy=10t−16t2. Find trajectory of the particle.
View Solution
