A particle moving with a speed v changes direction by an angle $θ$ , without change in speed.

A

(a)The change in the magnitude of its velocity is zero

B

(b)The change in the magnitude of its velocity is The magnitude of the change in its velocity is 2 v sin $(θ / 2)$

C

(c)The magnitude of the change in its velocity is $v (1 - cos θ)$

D

(d)The magnitude of the change in its velocity is 2v sin (θ/2)

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, C

${- v}_{1} = v ˆ i, {- v}_{f} = V cos θ ˆ i + V sin θ ˆ j \Rightarrow △ - V = (V cos θ - v) ˆ i + v sin θ ˆ j$
$∣ ∣ △ - v ∣ ∣ = \sqrt{v^{2} {cos}^{2} θ + v^{2} - 2 v^{2} cos θ + v^{2} {sin}^{2} θ}$
$= \sqrt{2 v^{2} - 2 v^{2} {cos}^{2} θ} = 2 v sin (θ / 2)$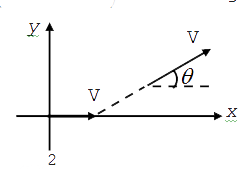

Updated on:21/07/2023

|

Class 12 PHYSICS Motion in Two Dimensions

Topper's Solved these Questions

Similar Questions

Knowledge Check

A particle is thrown with a speed is at an angle $θ$ with the horizontal. When the particle makes an angle $ϕ$ with the horizontal, its speed changes to v, then
A $v = v_{0} cos θ$
B $v = v_{0} cos θ \cdot cos ϕ$
C $v = v_{0} cos θ \cdot sec ϕ$
D $v = v_{0} sec θ \cdot cos ϕ$

VMC MODULES ENGLISH-Motion in Two Dimensions-Level -1

Home
Ask Doubt
Profile