Question

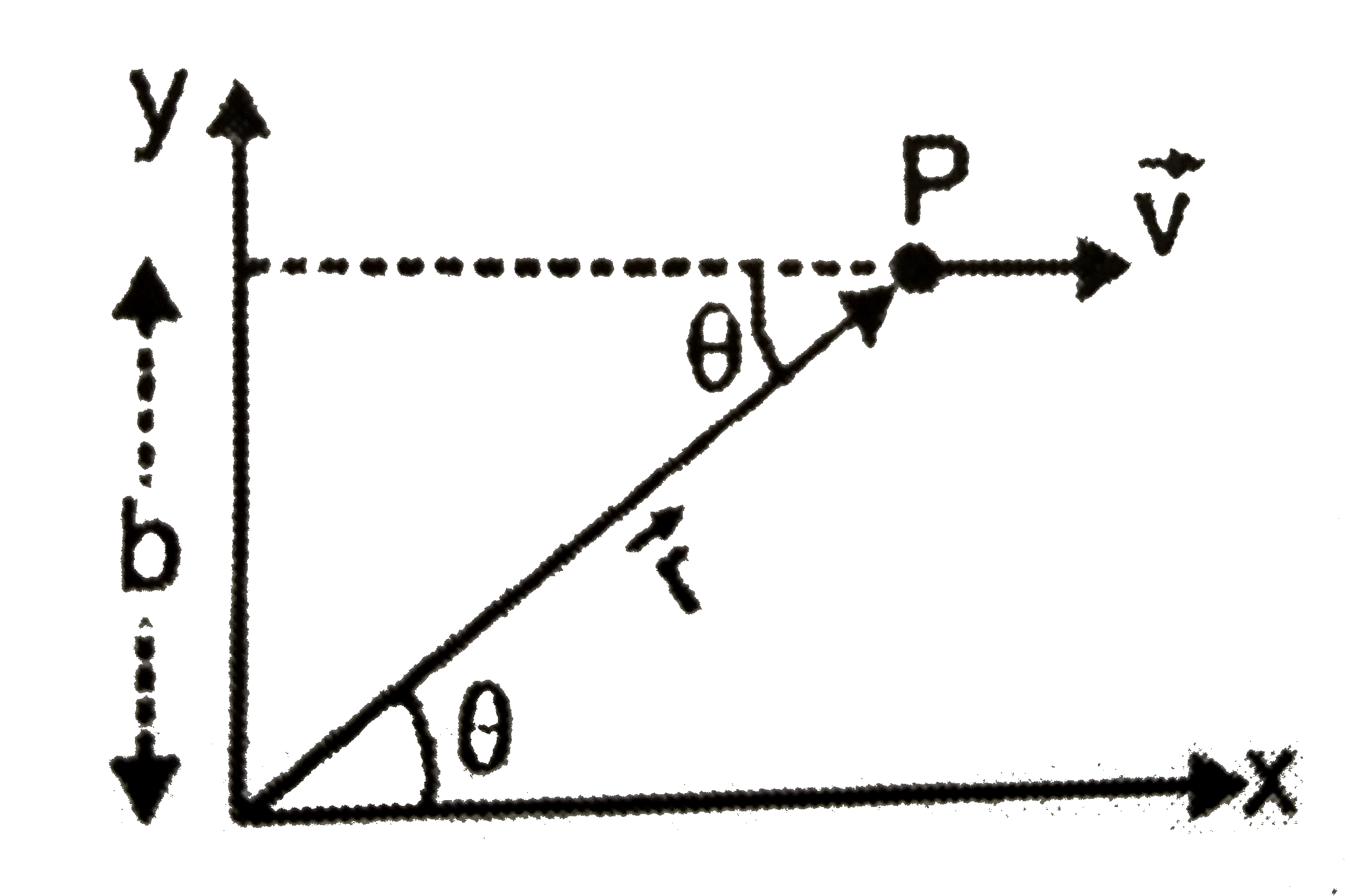
A particle is moving with constant speed v along the line y = a in positive x -direction. Find magnitude of its angular velocity about orgine when its position makes an angle $\theta$ with x-axis.

Explanation

To solve the problem of finding the magnitude of the angular velocity of a particle moving along the line $$y=a$$ at a constant speed $$v$$ when its position makes an angle $$\theta$$ with the x-axis, we can follow these steps:

1. Understand the Position of the Particle:
The particle is moving along the line \( y = a \). At a certain instant, it makes an angle \( \theta \) with the x-axis. The coordinates of the particle can be expressed as \( (x, a) \).

2. Determine the Radius Vector:
The radius vector \( r \) is the distance from the origin to the particle. Since the particle is at position \( (x, a) \), we can express \( r \) using the right triangle formed:
\( r = \sqrt{x^2 + a^2} \)

3. Relate the Radius to the Angle:
From the right triangle, we can express \( r \) in terms of \( a \) and \( \theta \):
\( r \sin \theta = a \implies r = \frac{a}{\sin \theta} \)

4. Calculate the Component of Velocity:
The particle moves with a constant speed \( v \) along the x-axis. To find the component of this velocity that is perpendicular to the radius vector, we note that the angle between the velocity vector (which is along the x-axis) and the radius vector can be determined. The perpendicular component of the velocity \( V_{\perp} \) is given by:
\( V_{\perp} = v \sin \theta \)

5. Use the Relation Between Linear Velocity and Angular Velocity:
The relationship between linear velocity \( V \), angular velocity \( \omega \), and radius \( r \) is given by:
\( V = r \omega \)
Rearranging this gives:
\( \omega = \frac{V_{\perp}}{r} \)

6. Substitute the Values:
Now substituting \( V_{\perp} \) and \( r \) into the equation for \( \omega \):
\( \omega = \frac{v \sin \theta}{\frac{a}{\sin \theta}} = \frac{v \sin^2 \theta}{a} \)

7. Final Result:
Thus, the magnitude of the angular velocity \( \omega \) about the origin when the particle's position makes an angle \( \theta \) with the x-axis is:
\( \omega = \frac{v \sin^2 \theta}{a} \)