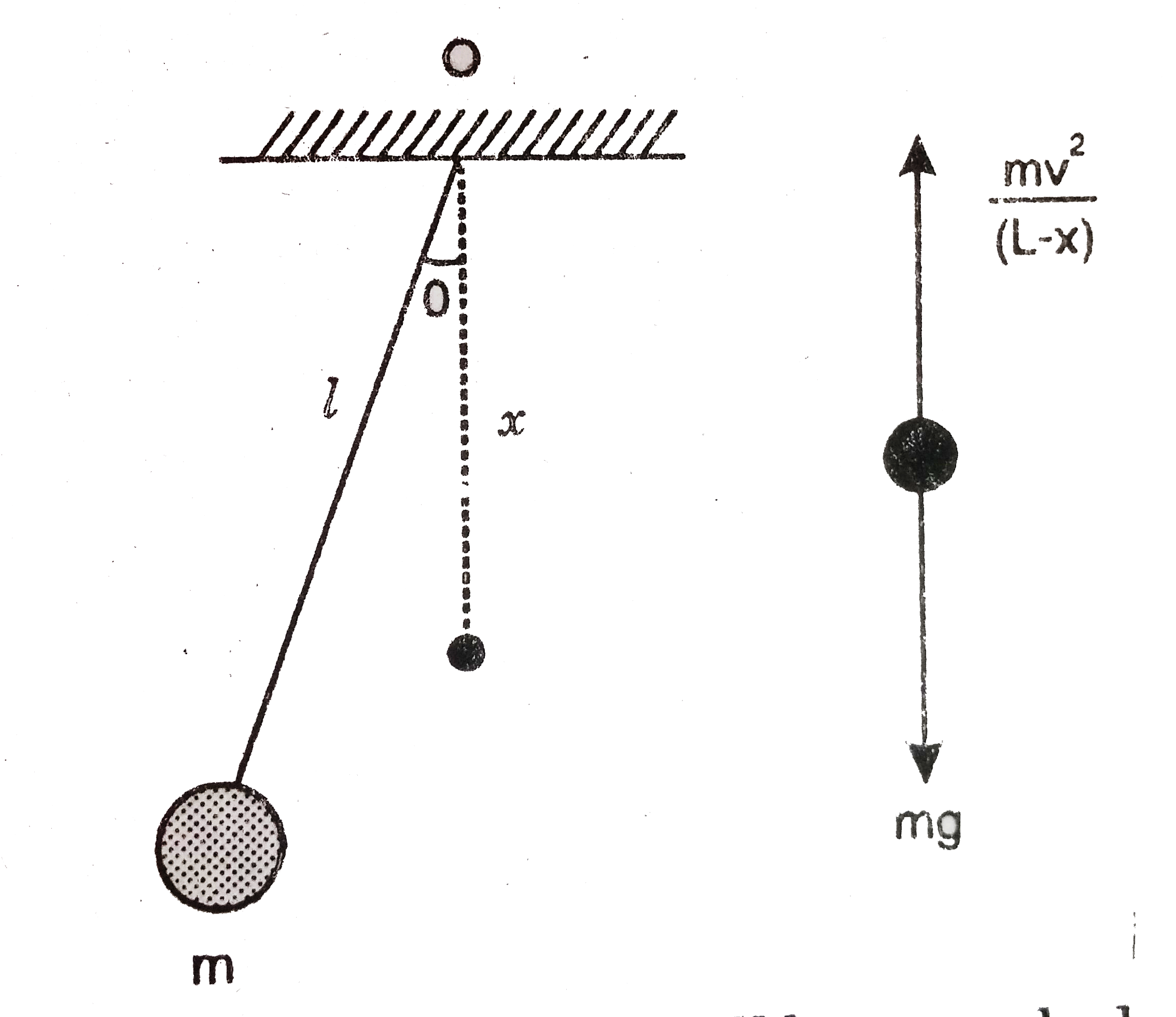
A simple pendulum of length L having a bob of mass m is deflected from its rest position by an angle θ and released figure. The string hits a peg which is fixed at distance x below the point of suspension and the bob starts going in a circle centred at the peg. a. Assuming that initially the bob has a height less thasn the peg, show that the maximum height reached by the bob equals its initialheight. b. If the pendulum is released with θ=900 and m=L2 find the maximum height reached by the bob above its lowest positon before the string becomes slack. c. Find the minimum value of x/L for which the bob goes in a complete circle about the pet when the pendulum is released from θ=900.
The correct Answer is:A, B, C
a. When the bob has an initial height less than the peg and the released from rest ure let body travels from A to B since, total energy at A
=Totale≠rgyatB,<br>:.(KE)_A+(PE)_A=(KE)_B+(PE)_B<br>rarr(PE)_=(PE)_A<br>∵(KE)A=(KE)_B=0<br>Sothemaxiμmheightreachedbythebobisequal→∈itialheight.<br>b.Whenthependu––misre≤asedwiththeta ==90^0 and x=l/2, ure the path of the particle is shown in ure.

At point C the string wil becomke slack and so the particle wil start making projectile motion.
Apply law of conservation.
(12)mv2c−0=mg(L2)(1−cosα)
[because distance between A and C ]
In the vertical direction is
L2=(1−cosα)
→V4c=gL(1−cosα)............i
Again, from the feebody diagram ure
mv2cL2=mgcosα......ii
[becuseTC=0]
From equation i and equation ii
gL(1−cosα)=gL2cosα
→1−cosα=12cosα
→32cosα=1
→cosα=(23)......iii
to find highest positon C1 before the string becomes slack.
BF=L2+L2cosθ
=L2+L2×23
=L(12+13)
So, BF=(5L6)
c. If the principle has to complete a vertical circle at the point C
mv2cL−x=mg.......i
Again applying energy principle between A and C
(12)mv2c−0=mg(OC)
→(12)mv2c=mg(L−2(l−x))
=mg(2x−L)
→v2c=2g(2x−L) .......ii
From equation i and ii
g(L−x)=2g(2x−L)
→L−x=4x−2L
→5x=3L
∴xL=35=0.6
So, the rates (xL) should be 0.6.
Topper's Solved these Questions
- WORK AND ENERGYBOOK - HC VERMA ENGLISHCHAPTER - WORK AND ENERGYEXERCISE - Question for Short Answer
16 Videos
- WORK AND ENERGYBOOK - HC VERMA ENGLISHCHAPTER - WORK AND ENERGYEXERCISE - Objective -2
8 Videos
- WAVE MOTION AND WAVES ON A STRINGBOOK - HC VERMA ENGLISHCHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Question for short Answer
7 Videos
Similar Questions
A simple pendulum of mass m and length L is held in horizontal position. If it is released from this position, then find the angular momentum of the bob about the point of suspension when it is vertically below the point of suspension.
View SolutionA simple pendulum of length l and mass m is initially at its lowest position. It is given the minimum horizontal speed necessary after to move in a circular path about the point of suspension. The tension in the string at the lowest positive of the bob is
View SolutionA simple pendulm has a length L and a bob of mass M. The bob is vibrating with amplitude a .What is the maximum tension in the string?
View SolutionA simple pendulum of length 'l' and having bob of mass 'm' is doing angular SHM inside water A constant buoyant force equal to half the weight of he bob is acting on the ball. Find the time period of oscillation?
View SolutionA simple pendulum of length l and bob of mass m is displaced from its equilibrium position O to a position P so that hight of P above O is h . If is then released. What is the tension in the string when the bob passes through the through the equilibrium position O ? Neglect friction. v is the velocity of the bob at O
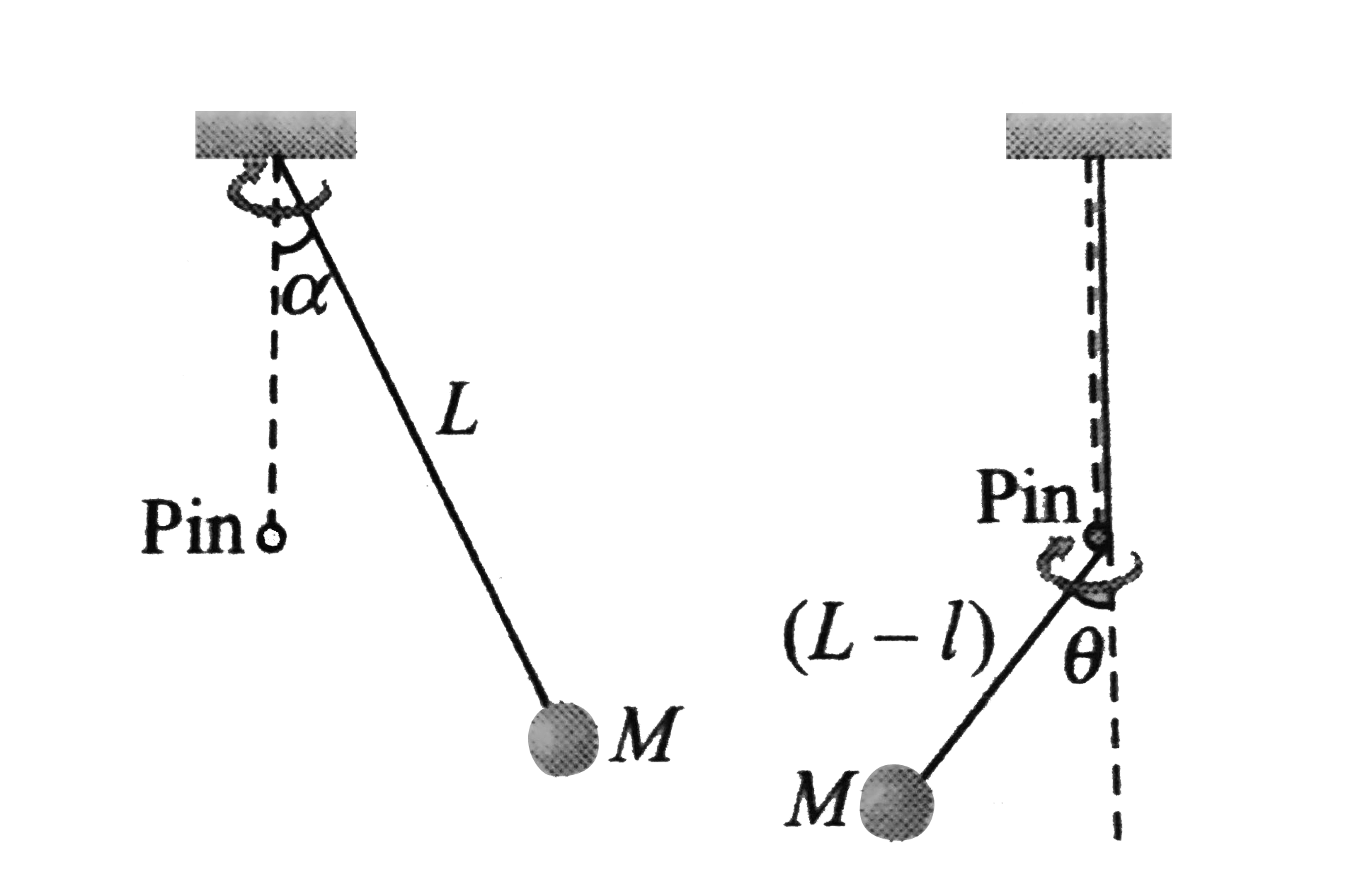
View SolutionA simple pendulum consisting of a mass M attached to a string of length L is released from rest at an angle α. A pin is located at a distance l below the pivot point. When the pendulum swings down, the string hits the pin as shown in figure. The maximum angle θ which the string makes with the vertical after hitting the pin is
View SolutionA simple pendulum , made of a string of length l and a bob of mass m, is released from a small angle θ0 it strikes a block of mass M, kept on a horizontal surface at its lowest point of oscillations , elastically , it bounces back and goes up to an angle θ1 then M is given by :
View SolutionA simple pendulum , made of a string of length l and a bob of mass m, is released from a small angle θ0 it strikes a block of mass M, kept on a horizontal surface at its lowest point of oscil,ations , elastically , it bounces back and goes up to an angle θ)(1−) then M is given by :
View SolutionA simple pendulum of length l and mass m free to oscillate in vertical plane. A nail is located at a distance d=l−a vertically below the point of suspension of a simple pendulum. The pendulum bob is released from the position where the string makes an angle of 90∘ from vertical. Discuss the motion of the bob if (a) l=2a and (b) l=2.5a.
View SolutionSuppose the amplitude of a simple pendulum having a bob of mass m is θ0. Find the tension in the string when the bob is at its extreme position.
View Solution
