A heavy particle is usspended by a 1.5 m long string . It is given a horizontal velocityof $\sqrt{57} \frac{m}{s} .$ a. Find the angle made by the string with the upward vertical, when it becomes slack. B. Find the speed of the particle at this instant. c.Find the maximum height reached by the particle over the point of suspension. Take $g = 10 \frac{m}{s^{2}}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, B, C

$L = 1.5 m, u = \sqrt{57} \frac{m}{sec}$
a. $m g cos θ = \frac{m v^{2}}{L}$
$v^{2} = L g cos θ$
change in K.E.= work done
$\frac{1}{2} m v^{2} - \frac{1}{2} μ^{2} = m g h$
$\to v^{2} - 57 = - 2 \times 1.5 g (1 + cos θ)$ ……….ii
$\to v^{2} = 57 - 3 g (1 + cos θ)$

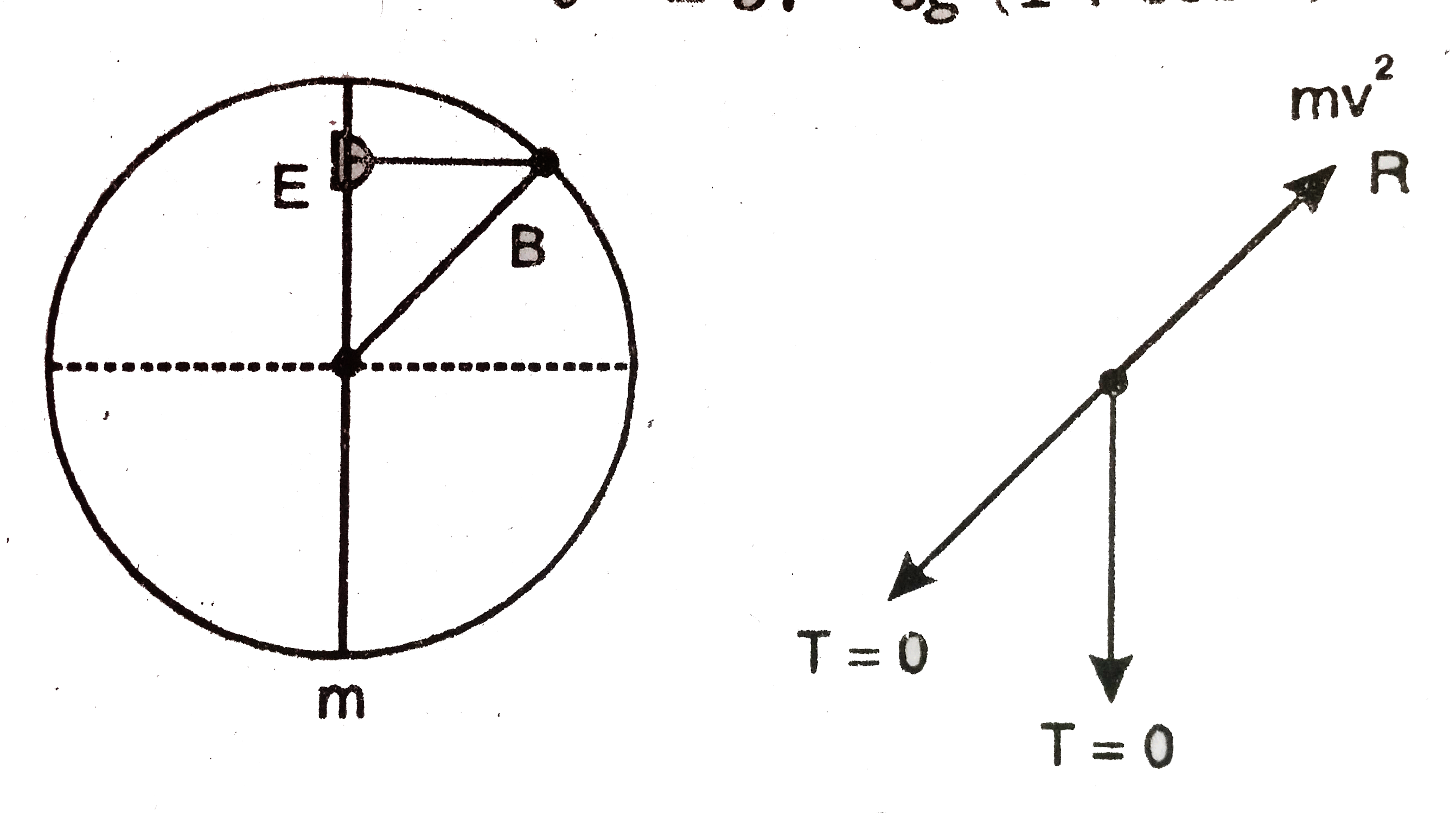
Putting the value of v from equaltion i.
$15 cos θ - 57 - 3 g (1 + cos θ)$
$\to 15 cos θ = 57 - 30 - 30 cos θ$
$\to 45 cos θ = 27$
$\to cos θ = \frac{3}{5}$
$\to θ = \frac{{cos}^{- 13}}{5} = 53^{0}$
b. $v = \sqrt{57 - 3 g (1 + cos θ)}$ from equation i
$\sqrt{9} = \frac{e}{sec .} < b r > c . a s t h e s t r \in g b e c o m e s l a c k a t p \oint B, t h e p a r t i c \leq w i l l ⋆ t m a k i g n p r o j e c t i o n t i \leq m o t i o n < b r >$ H=OF+DC $< b r >$ =1.5costheta+(u^2sin^2theta)/(2g) $< b r >$ (1.5)xx3/5xx(9xx(0.8)^2)/(2xx10) $< b r >$ 1.2m

Updated on:21/07/2023

|

Class 11 PHYSICS WORK, ENERGY AND POWER

Topper's Solved these Questions

Similar Questions

HC VERMA ENGLISH-WORK AND ENERGY-Exercises

Home
Ask Doubt
Profile