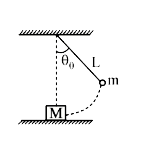
A simple pendulum , made of a string of length l and a bob of mass m, is released from a small angle θ0 it strikes a block of mass M, kept on a horizontal surface at its lowest point of oscillations , elastically , it bounces back and goes up to an angle θ1 then M is given by :
m2(θ0+θ1θ0−θ1)
m(θ0−θ1θ0+θ1)
m(θ0+θ1θ0−θ1)
m2(θ0−θ1θ0+θ1)
The correct Answer is:C
ω=√gl.
Velocity just before collision u=(√glθ0)l=√glθ0
v = velocity of ball after collision v=(√glθ1)l=√glθ1.
Momentum conservation : m u = Mv'-mv ………(i)
e=1:
v'+v=u ......(ii)
ω=√gl From (i) and (ii)
m(u+v)=M(u−v)
m(θ0+θ1)=M(θ0−θ1)
M=m(θ0+θ1θ0−θ1).
 .
.
Topper's Solved these Questions
- JEE MAIN REVISION TEST 8 (2020)BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST 8 (2020)EXERCISE - PHYSICS (SECTION 2)
5 Videos
- JEE MAIN REVISION TEST 5 (2020)BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST 5 (2020)EXERCISE - PHYSICS (SECTION 2)
5 Videos
- JEE MAIN REVISION TEST- 16BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST- 16EXERCISE - PHYSICS (SECTION 2)
5 Videos
Similar Questions
A simple pendulm has a length L and a bob of mass M. The bob is vibrating with amplitude a .What is the maximum tension in the string?
View SolutionWhat is the period of oscillation of a simple pendulum if its bob is made of ice ?
View SolutionA simple pendulum with a bob of mass m is suspended from the roof of a car moving with horizontal acceleration a
View SolutionA simple pendulum of mass m and length L is held in horizontal position. If it is released from this position, then find the angular momentum of the bob about the point of suspension when it is vertically below the point of suspension.
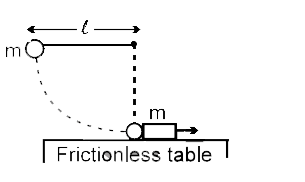
View SolutionThe bob of a simple pendulum of length l dropped from a horizontal position strikes a block of the same mass elastically, placed on a horizontal table (frictionless) as shown in the diagram, the block shall have kinetic energy-
View SolutionA stone tied to an inextensible string of length l=1m is kept horizontal. If it is released, find the angular speed of the stone when the string makes an angle θ=30∘ with horizontal.
View SolutionA rod of mass m is released on smooth horizontal surface making angle θ with horizontal. Then which of the following statement is correct ?
View SolutionA pendulum consists of a small mass m at the end of a string of length L. The pendulum is pulled aside making an angle θ with the vertical and released. Taking the suspension point as the axis, at the instant of release
View SolutionA bob of mass m attached with a string of length l tied to a point on ceiling is released from a position when its string is horizontal. At the bottom most point of its motion, an identical mass m gently stuck to it. Find the maximum angle from the vertical to which it rotates.
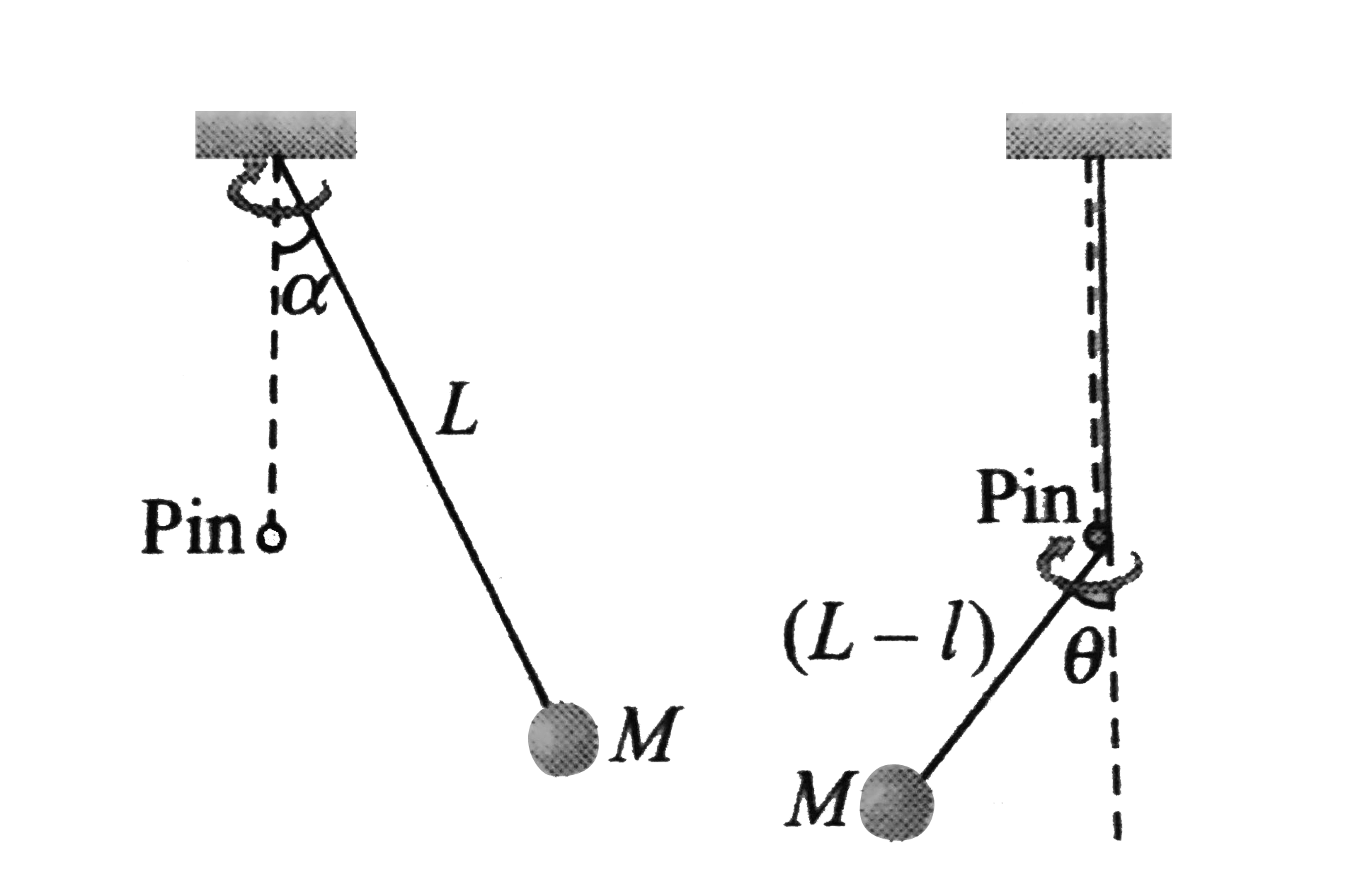
View SolutionA simple pendulum consisting of a mass M attached to a string of length L is released from rest at an angle α. A pin is located at a distance l below the pivot point. When the pendulum swings down, the string hits the pin as shown in figure. The maximum angle θ which the string makes with the vertical after hitting the pin is
View Solution
