Let a circle be given by 2x(x−1)+y(2y−b)=0,(a≠0,b≠0) . Find the condition on aandb if two chords each bisected by the x-axis, can be drawn to the circle from (a,b2)
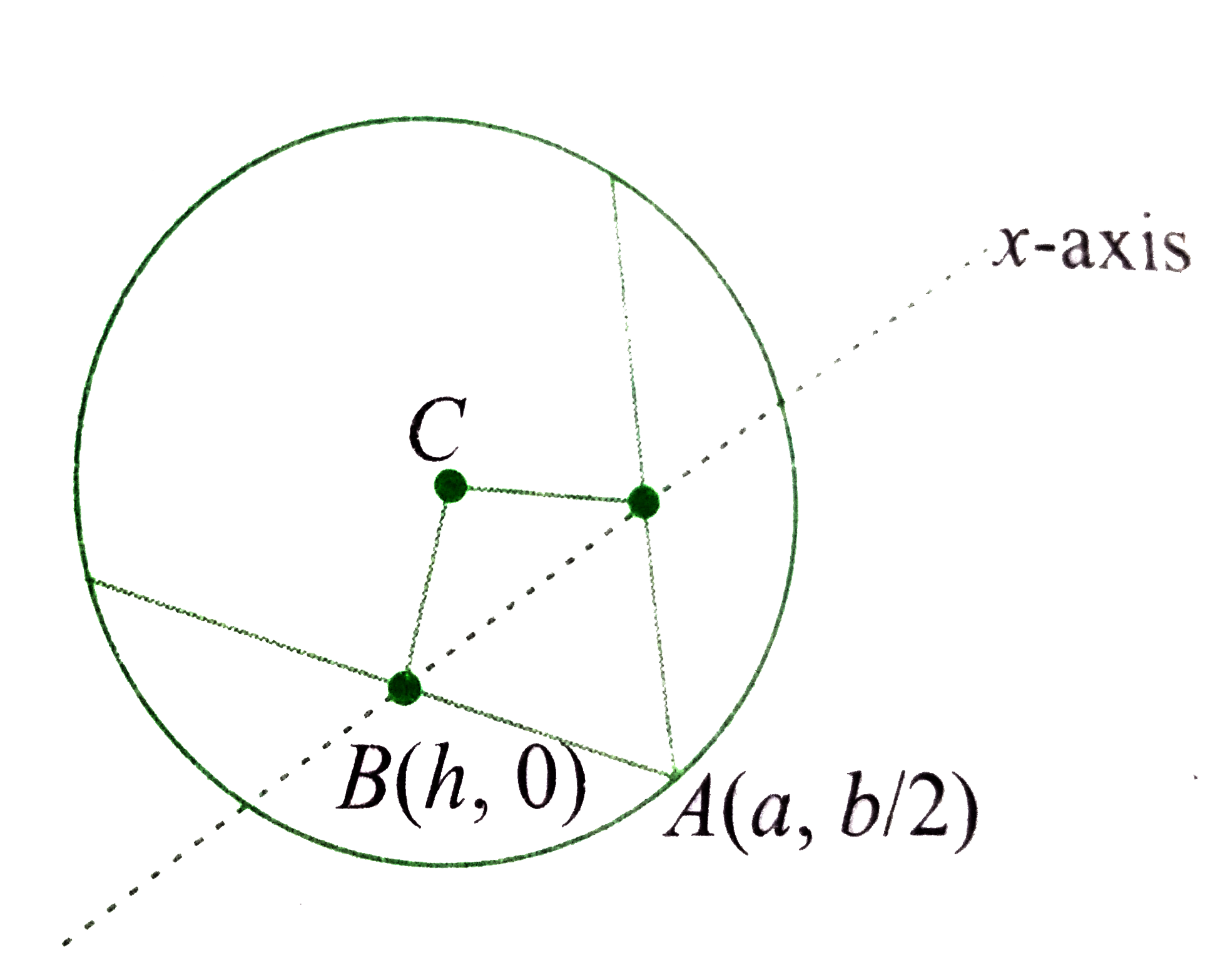
The given circle is
2x(x-a)+y(2y-b)=0(a,b cancel(=)0)
or x2+y2−ax−b2y=0
Its center is C(a/2,b/4).
Also, point A(a,b/2) lies on the circle.
Since chord is bisected at point B (h,0) on the x-axis, we have
(Slope of BC) × (Slope of AB ) =−1
or {(b/s)−0(a/2)−h}{(b/2)−0a−h}=−1
h2−3a2h+a22+b28=0
Since two such chords exist, the above equation must have tow distinct real roots, for which its discrimination must be positive i.e.,
or 9a24−4(a22+b28)>0
or a2>2b2
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - CONCEPT APPLICATION EXERCISE 4.1
1 Videos
- CIRCLE BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - CONCEPT APPLICATION EXERCISE 4.2
2 Videos
- CIRCLE BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - MATRIX MATCH TYPE
7 Videos
- BINOMIAL THEOREMBOOK - CENGAGE ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - Matrix
4 Videos
- CIRCLESBOOK - CENGAGE ENGLISHCHAPTER - CIRCLESEXERCISE - Comprehension Type
8 Videos
Similar Questions
Let a circle be given by 2x(x−a)+y(2y−b)=0,(a≠0,b≠0) . Find the condition on aandb if two chords each bisected by the x-axis, can be drawn to the circle from (a,b2)
View SolutionColumn I|Column II The length of the common chord of two circles of radii 3 units and 4 units which intersect orthogonally is k5 . Then k is equal to|p. 1 The circumference of the circle x2+y2+4x+12y+p=0 is bisected by the circle x2+y2−2x+8y−q=0. Then p+q is equal to|q. 24 The number of distinct chords of the circle 2x(x−√2)+y(2y−1)=0 , where the chords are passing through the point (√2,12) and are bisected on the x-axis, is|r. 32 One of the diameters of the circle circumscribing the rectangle ABCD is 4y=x+7. If AandB are the poinst (−3,4) and (5, 4), respectively, then the area of the rectangle is|s. 36
View Solution
Knowledge Check
Find the length of the chord intercepted by the circle x2+y2−8x−6y=0 on the line x−7y−8=0.
A5B√2C5√2D6
Find the length of the chord of contact with respect to the point on the director circle of circle x2+y2+2ax−2by+a2−b2=0 .
View SolutionFind the length of the chord of contact with respect to the point on the director circle of circle x2+y2+2ax−2by+a2−b2=0 .
View SolutionFind the number of common tangents that can be drawn to the circles x2+y2−4x−6y−3=0 and x2+y2+2x+2y+1=0
View SolutionFind the length of the chord intercepted by the circle x2+y2=25 on the line 2x -y + 5= 0
View SolutionFind the equation to the chord of contact of the tangents drawn from an external point (−3,2) to the circle x2+y2+2x−3=0.
View SolutionFind the equation to the chord of contact of the tangents drawn from an external point (−3,2) to the circle x2+y2+2x−3=0.
View SolutionThe least positive integral value of λ for which two chords bisected by x-axis can be drawn to the circle x2+y2−λax−ay−a2(λ2+1)=0, from point (a(λ−1),a(λ+1) is ..
View Solution
