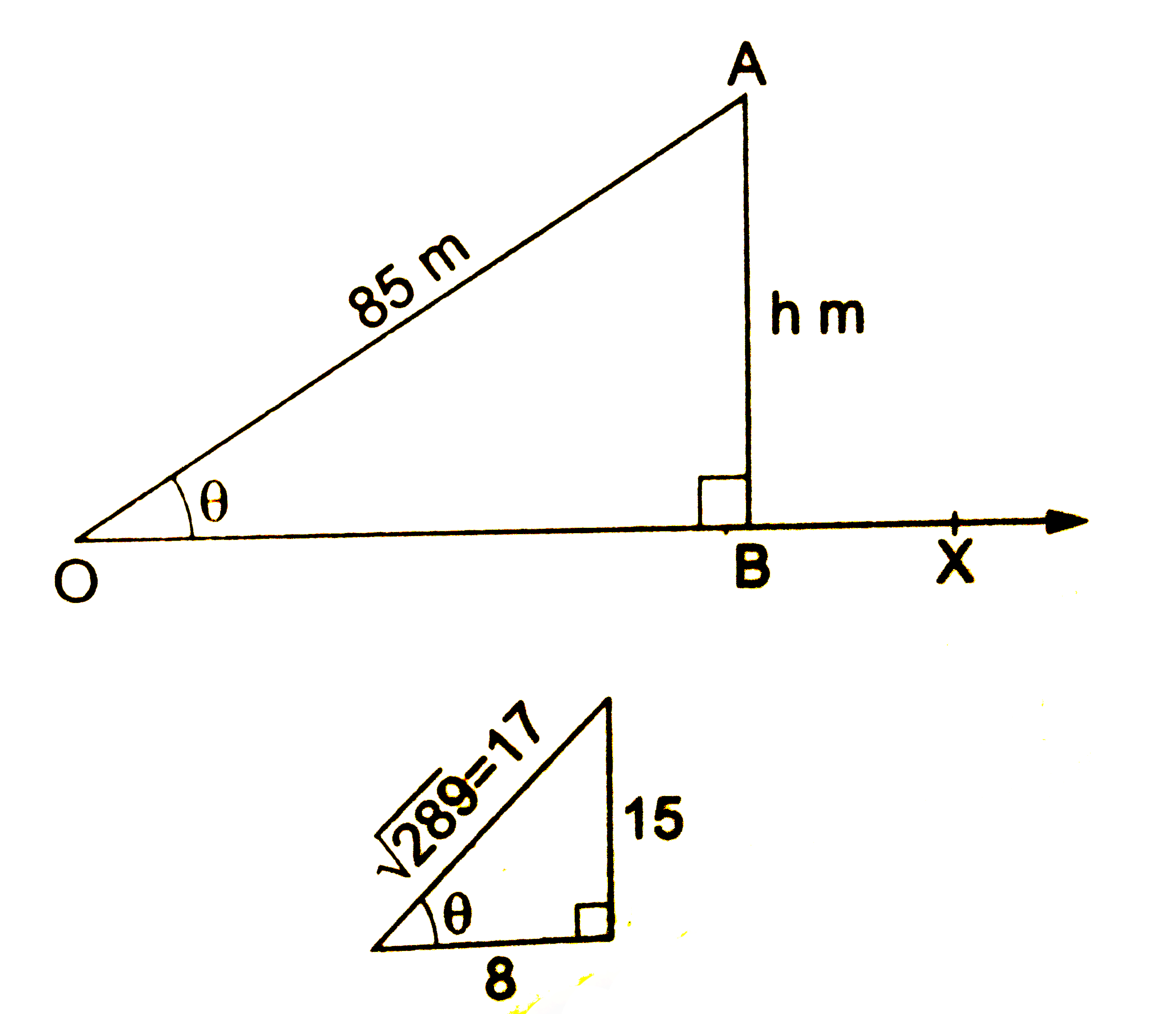
The length of a string between a kite and a point on the ground is 85 metres. If the string makes an angle θ with the ground level such that tan θ = 15 8 , how high is the kite? Assume that there is no slack in the string.

A

79 m

B

76 m

C

78 m

D

75 m

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:D

Let OX be the horizontal ground and let A be the position of the kite. Let O be the position of the observer and OA be the string. Draw A B ⊥ O X .
Then, ∠ B O A = θ and tan θ = 15 8 , O A = 85 m and ∠ O B A = 90 ∘ .
Let A B = h metres.
From height Δ O B A , we have
A B O A = sin θ = 15 17 [ ∵ tan θ = 15 8 ⇒ sin = 15 17 ]
⇒ h 85 = 15 17 ⇒ h = 15 17 × 85 = 75 .
Hence the height of the kite from the ground is 75 m .
ocr_image

Updated on:21/07/2023
|
Class 10MATHSSOME APPLICATIONS OF TRIGONOMETRY

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • A kite is flying at the height of 75m from the ground. The string makes an angle θ (where cotθ =815 ) with the level ground. Assuming that there is no slack in the string the new length of the string is equal to :

    A85 metre
    B65 metre
    C75 metre
    D40 metre

  • A kite is flying at the height of 75 m from the ground .The string makes an angle θ (where cotθ=815) with the level ground .As - summing that there is no slack in the string the length of the string is equal to :

    A85 metre
    B65 metre
    C75 metre
    D40 metre