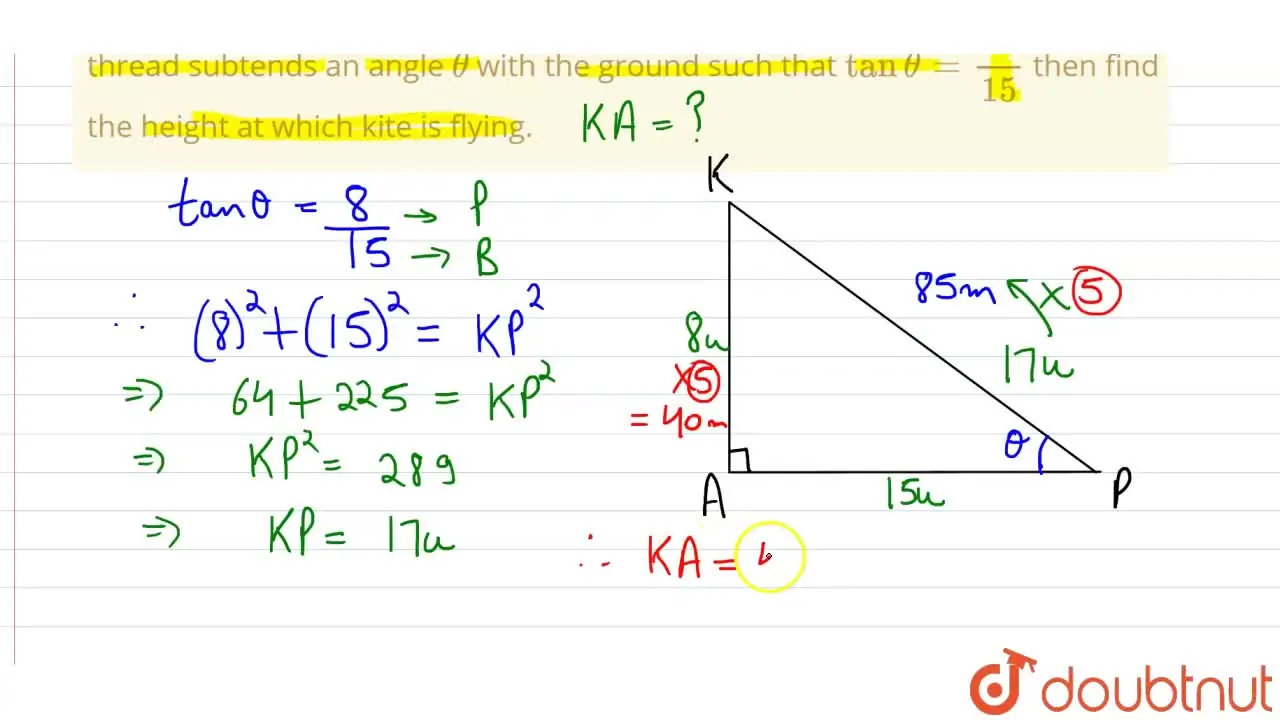
Length of the tight thread of a kite from a point on the ground is 85 m. If thread subtends an angle θ with the ground such that tanθ=815 then find the height at which kite is flying.
The correct Answer is:40 meter
Step by step video, text & image solution for Length of the tight thread of a kite from a point on the ground is 85 m. If thread subtends an angle theta with the ground such that tantheta=8/15 then find the height at which kite is flying. by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Related Playlists
- BOOK - LUCENT PUBLICATIONCHAPTER - HEIGHT AND DISTANCEEXERCISE - EXERCISE-12A56videos
HEIGHT AND DISTANCE
- BOOK - LUCENT PUBLICATIONCHAPTER - HEIGHT AND DISTANCEEXERCISE - EXERCISE-12B14videos
HEIGHT AND DISTANCE
- BOOK - LUCENT PUBLICATIONCHAPTER - GRAPHICAL SOLUTION OF LINEAR EQUATION EXERCISE - EXERCISE-3B8videos
GRAPHICAL SOLUTION OF LINEAR EQUATION
- BOOK - LUCENT PUBLICATIONCHAPTER - INDICES AND SURDS EXERCISE - Exercise - 2B14videos
INDICES AND SURDS
Similar Questions
The length of a string between a kite and a point on the ground is 90 metres. If the string makes an angle θ with the ground level such that tanθ=158, how high is the kite? Assume that there is no slack in the string.
View SolutionA kite with 68 metres string is in the sky.The string is making an angle θ with the ground so that tanθ=158 Find the height of the kite
View SolutionThe length of a string between kite and a point on the ground is 90 m. If the string makes an angle with the level ground and sinα=35. Find the height of the kite. There is no slack in the string.
View SolutionA vertical tower PQ subtends the same anlgle of 30∘ at each of two points A and B ,60 m apart on the ground .If AB subtends an angle of 120∘ at p the foot of the tower ,then find the height of the tower .
View SolutionA kite is flying at the height of 75m from the ground. The string makes an angle θ (where cotθ =815 ) with the level ground. Assuming that there is no slack in the string the new length of the string is equal to :
View SolutionA kite is flying in the sky. The length of string between a point on the ground and kite is 420 m. The angle of elevation of string with the ground is 30∘ Assuming that there is no slack in the string, then what is the height (in metres) of the kite?
View SolutionA kite is flying at a height of 60m above the ground. The string attached to the kite is temporarily tied to a point on the ground. The inclination of the string with the ground is 60o . Find the length of the string assuming that there is no slack in the string.
View SolutionA kite is flying at a height of 60 m above the ground. The string attached to kite is temporarily tied to a point on the ground. The inclination on the string with the ground is 60∘. Find the length of the string assuming that there is no slack in the string.
View SolutionThe angle of depression between a flying kite and 'a' point on the ground is 60 m. If the string makes 30∘. Find the horizontal distance between the ship and tower.
View SolutionA pole is standing erect on the ground which is horizontal. The tip of the poke is tied tight with a rope of length √12 m to a point on the ground. If the rope is making 30∘ angle with the horizontal , then the height of the pole is
View Solution