The squared length of the intercept made by the line $x = h$ on the pair of tangents drawn from the origin to the circle $x^{2} + y^{2} + 2 g x + 2 f y + c = 0$ is $\frac{4 c h^{2}}{{(g^{2} - c)}^{2}} (g^{2} + f^{2} - c)$ $\frac{4 c h^{2}}{{(f^{2} - c)}^{2}} (g^{2} + f^{2} - c)$ $\frac{4 c h^{2}}{{(f^{2} - f^{2})}^{2}} (g^{2} + f^{2} - c)$ (d) none of these

A

$\frac{4 c h^{2}}{(g^{2} - c^{2})} (g^{2} + f^{2} - c)$

B

$\frac{4 c h^{2}}{(f^{2} - c^{2})} (g^{2} + f^{2} - c)$

C

$\frac{4 c h^{2}}{{(g^{2} - f^{2})}^{2}} (g^{2} + f^{2} - c)$

D

none of these

Video Solution

Text Solution

Verified by Experts

The correct Answer is:2

The equation of the pair of tangents from (0,0) is $S S' = T^{2}$
or $(x^{2} + y^{2} + 2 g x + 2 f y + c) c = {(g x + f y + c)}^{2}$ (1)
The intersection points of the above pair with $x = h$ are given by
${(g h + f y + c)}^{2} = (h^{2} + y^{2} + 2 g h + 2 f y + c) c$
or $(f^{2} - c) y^{2} + 2 f g h y + h^{2} (g^{2} - c) = 0$

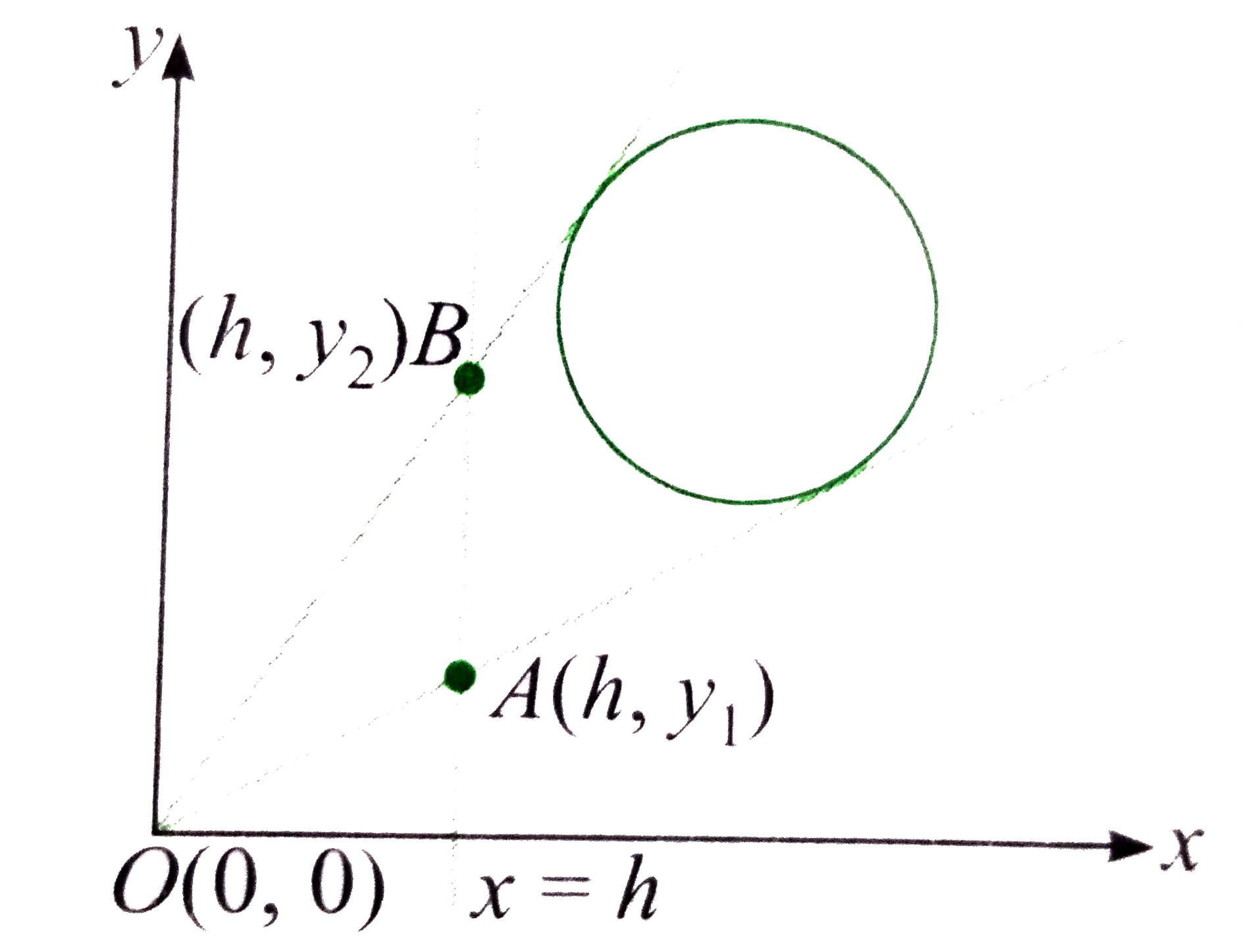
If its roots are $y_{1}$ and $y_{2}$ , then the length of intercept
$A B^{2} = {| y_{1} - y_{2} |}_{1}^{2}$
$= {(y_{1} + y_{2})}_{1}^{2} - 4 y_{1} y_{2}$
$= {(\frac{2 f g h}{f^{2} - c})}^{2} - 4 \frac{h^{2} (g^{2} - c)}{f^{2} - c}$
$= \frac{4 c h^{2}}{{(f^{2} - c)}^{2}} (g^{2} + f^{2} - c)$

|

Updated on:21/07/2023

Class 12 MATHS CIRCLE

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The length of the tangent drawn from any point on the circle $S = x^{2} + y^{2} + 2 g x + 2 f y + c = 0$ to the circle $S' = x^{2} + y^{2} + 2 g x + 2 f y + c' = 0$ where $c' > c$ is
A $\sqrt{c + c'}$
B $\sqrt{c - c'}$
C $\sqrt{c' - c}$
Dnone
Question 2 - Select One
The distance between the chords of contact of the tangents to the circle $x^{2} + y^{2} + 2 g x + 2 f y + c = 0$ from the origin and the point (g,f) is
A $g^{2} + f^{2}$
B $\frac{1}{2} (g^{2} + f^{2} + c)$
C $\frac{1}{2} \frac{g^{2} + f^{2} + c}{\sqrt{} (g^{2} + f^{2})}$
D $\frac{1}{2} \frac{g^{2} + f^{2} - c}{\sqrt{} (g^{2} + f^{2})}$
Question 3 - Select One
The condition so that the line
$(x + g) cos θ + (y + f) sin θ = k$
is a tangent to $x^{2} + y^{2} + 2 g x + 2 f y + c = 0$ is
A $g^{2} + f^{2} = c + k^{2}$
B $g^{2} + f^{2} = c^{2} + k$
C $g^{2} + f^{2} = c^{2} + k^{2}$
D $g^{2} + f^{2} = c + k$

CENGAGE-CIRCLE -Exercise (Single)

Home
Ask Doubt
Profile