If C1:x2+y2=(3+2√2)2 is a circle and PA and PB are a pair of tangents on C1, where P is any point on the director circle of C1, then the radius of the smallest circle which touches c1 externally and also the two tangents PA and PB is 2√3−3 (b) 2√2−1 2√2−1 (d) 1
2√3−3
2√2−1
2√2−1
1
The correct Answer is:4
AQ=3+2√2
PQ=3√2+4
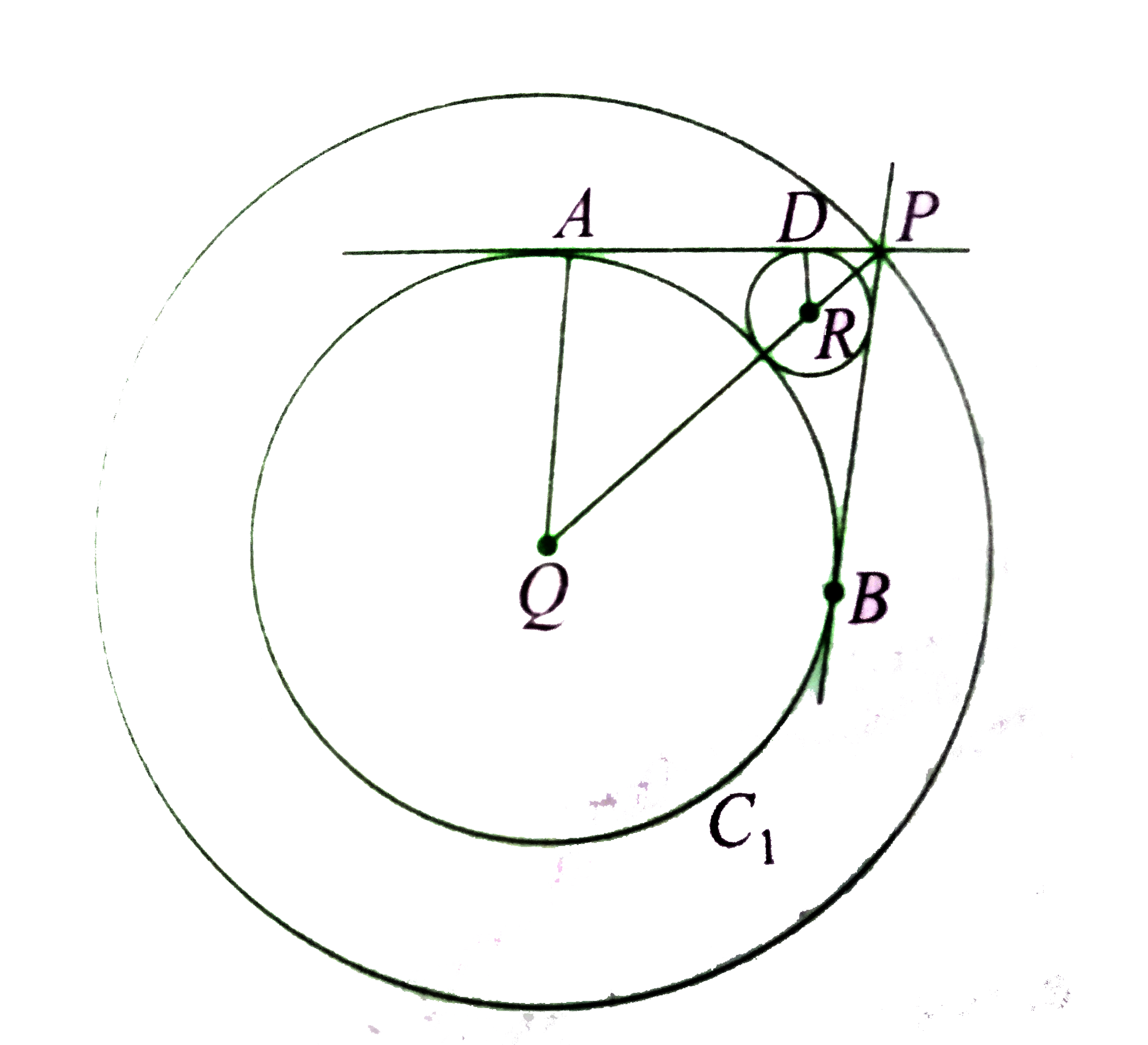
Let r be the required radius. Then,
3√2+4=3+2√2+r+r√2
√2+1=r(1+√2) or r=1
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Multiple)
25 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Comprehension)
46 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise 4.6
5 Videos
- BINOMIAL THEORMBOOK - CENGAGECHAPTER - BINOMIAL THEORMEXERCISE - Question Bank
31 Videos
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank
32 Videos
Similar Questions
The radius of a circle, having minimum area, which touches the curve y=4−x2 and the lines, y=|x| is: (a) 4(√2+1) (b) 2(√2+1) (c) 2(√2−1) (d) 4(√2−1)
View SolutionA circle S of radius 'a' is the director circle of another circle S1,S1 is the director circle of circle S2 and so on. If the sum of the radii of all these circle is 2, then the value of 'a' is 2+√2 (b) 2−1√2 2−√2 (d) 2+1√2
View Solution
Knowledge Check
If C1:x2+y2=(3+2√2)2 be a circle. PA and PB are pair of tangents on C1 where P is any point on the director circle of C1, then the radius of the smallest circle which touches C1 externally and also the two tangents PA and PB is
A1B2C3D4
The radius of the circle passing through the points (1,2),(5,2)and(5,−2) is : (A) 5√2 (B) 2√5 (C) 3√2 (D) 2√2
View SolutionA circle x2+y2+4x−2√2y+c=0 is the director circle of the circle S1andS1 is the director circle of circle S2, and so on. If the sum of radii of all these circles is 2, then the value of c is k√2 , where the value of k is___________
View SolutionThe direction ratio o the lien OP are euqla and the length OP=√3. Then the cooredinates of the point P are (A) (−1,−1,−1) (B) (√3,√3,√3) (C) (√2,√2,√2) (D) (2,2,2)
View SolutionThe line 2x−y+1=0 is tangent to the circle at the point (2, 5) and the center of the circle lies on x−2y=4. The radius of the circle is 3√5 (b) 5√3 (c) 2√5 (d) 5√2
View SolutionIf A(0,0),B(1,0)andC(12,√32) then the centre of the circle for which the lines AB,BC,CA are tangents is
View SolutionC1 is a circle of radius 1 touching the x- and the y-axis. C2 is another circle of radius greater than 1 and touching the axes as well as the circle C1 . Then the radius of C2 is 3−2√2 (b) 3+2√2 3+2√3 (d) none of these
View SolutionA pair of tangents are drawn to a unit circle with centre at the origin and these tangents intersect at the point P enclosing an angle of 600 . The area enclosed by the tangents and the arc of the circle is, 2√3−π6 (b) √3−π3 π3−√3 (d) √3(1−π6)
View Solution
