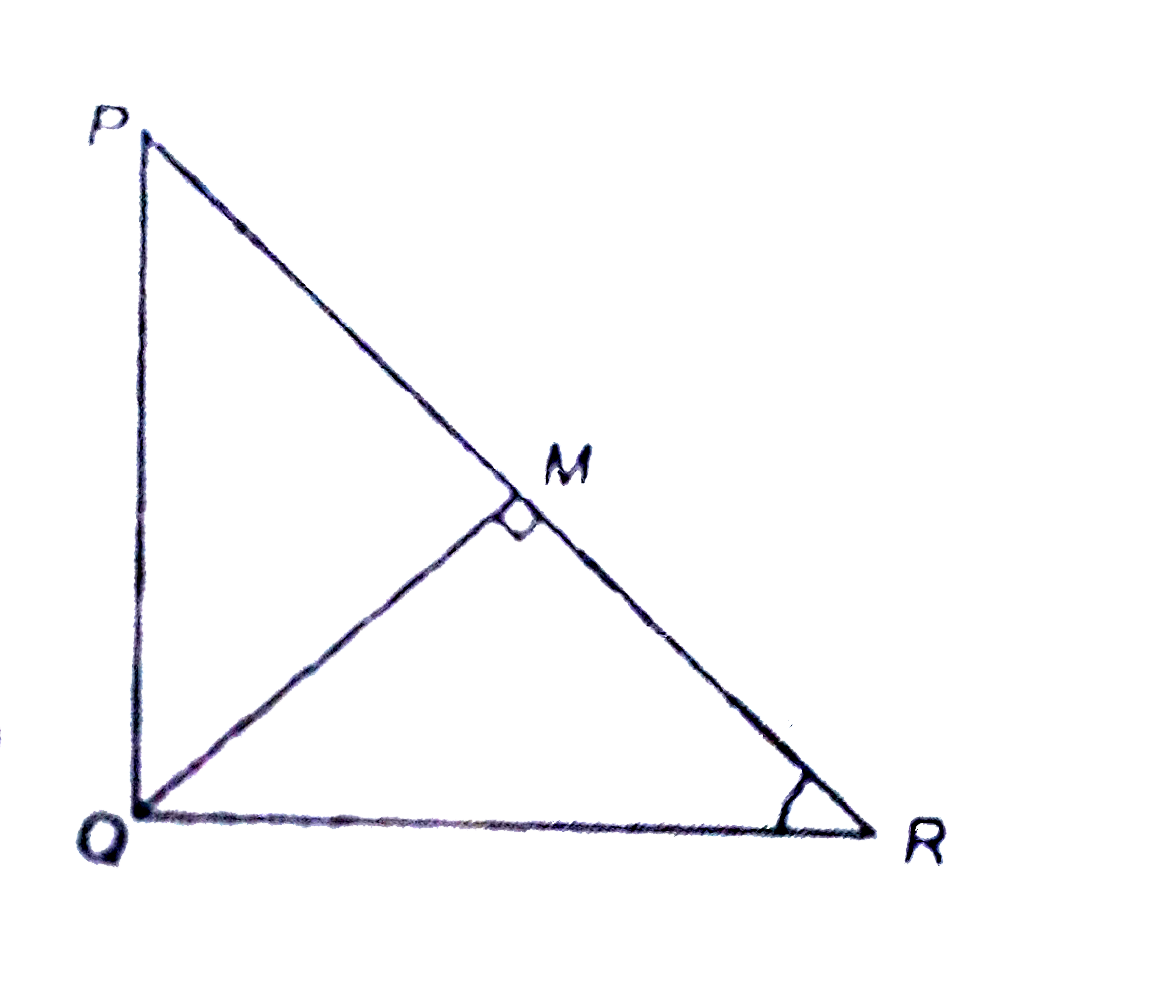
In $P Q R, Q M ⊥ P R$ and $P R^{2} - P Q^{2} = Q R^{2}$ . Prove that $Q M^{2} = P M \times M R$

Video Solution

Text Solution

Verified by Experts

Given that
$P R^{2} - P Q^{2} = Q R^{2} and Q M ⊥ P R$
$P R^{2} = P Q^{2} + Q R^{2}$
$∠ P Q R = 90^{\circ}$ ( converse of Pythagoras theorem)
In $△ Q M R and △ P M Q$
$∠ Q M R = ∠ P M Q (e a c h 90^{\circ}))$
$∠ M Q R = ∠ M P Q (e a c h 90^{\circ} = ∠ R)$
$△ Q M R ~ ∠ P M Q$ (AA similarity)
$\Rightarrow \frac{a r e a (△ Q M R)}{a r e a (△ P M Q)} = \frac{Q M^{2}}{P M^{2}}$
$\frac{\frac{1}{2} \times Q M \times R M}{\frac{1}{2} \times Q M \times P M} = \frac{Q M^{2}}{P M^{2}}$
$R M \times P M = Q M^{2}$

Updated on:21/07/2023