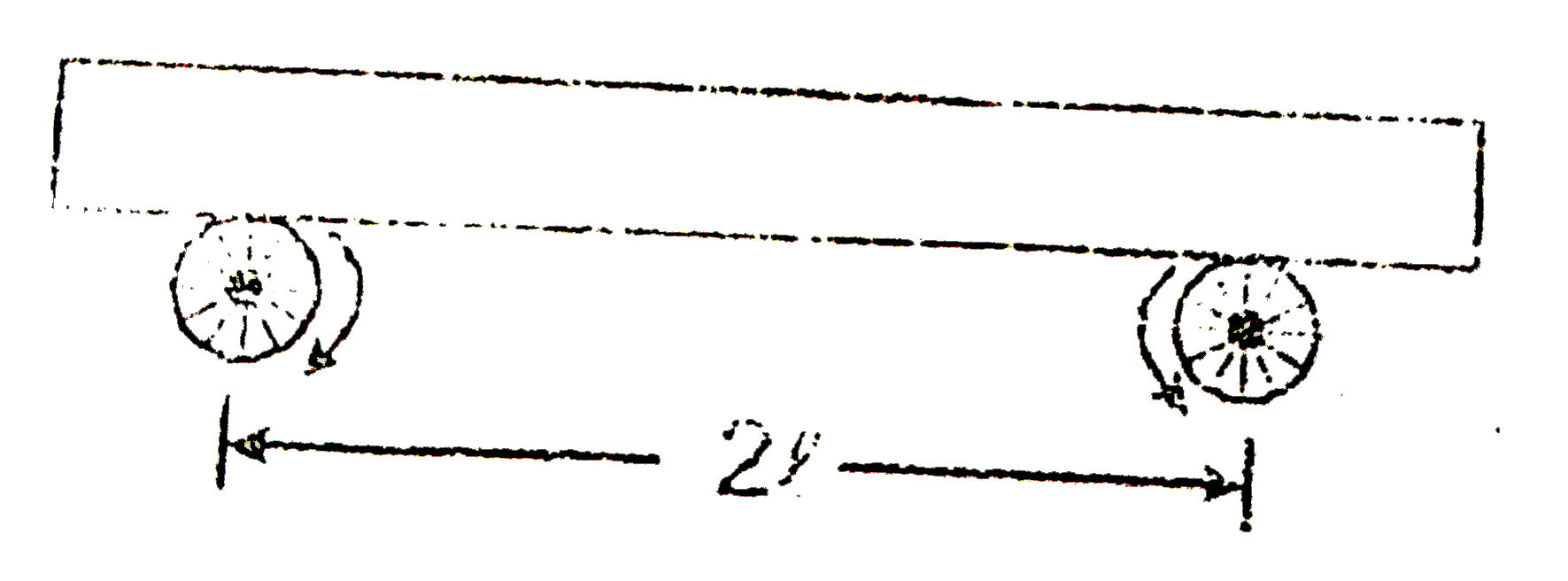
Two wheels which are rotated by some external source with constant angular velocity in opposite directions as shown in figure. A uniform plank of mass M is placed on it symmetrically. The friction co-efficient between each wheel and the plank is μ. find the frequency of oscillations, when plank is slightly displaced along its length and released.
The correct Answer is:2π√lμg
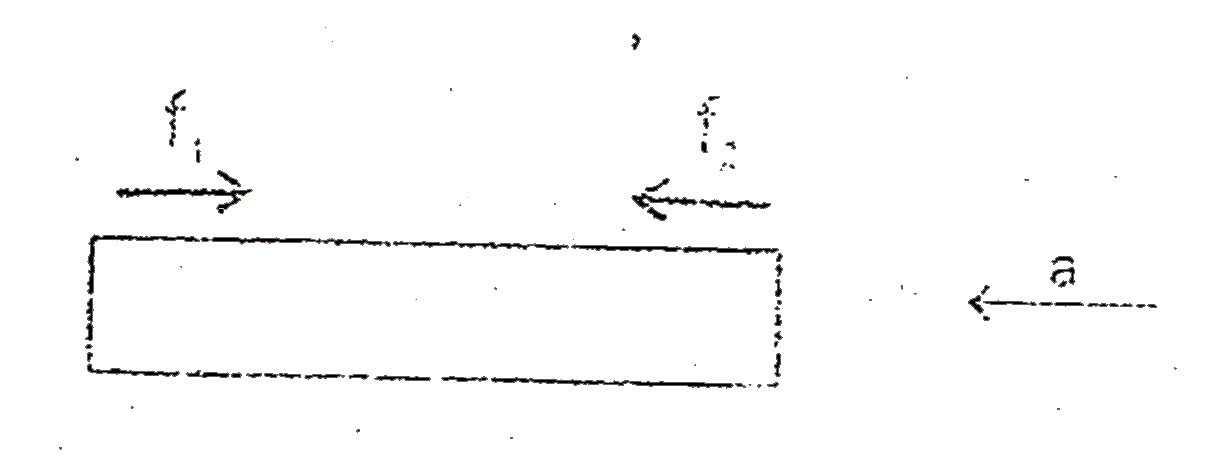
If plank is displaced by x toward right then

Let N1,N2 and f1,f2 are Normal and friction force at Point A and B by force balance
N1+N2=Mg1
amd Torque balance
Mg(l+X)=N2×2l_(2)
by equation (1) and (2)
N1=Mg2−Mgx2l,N2=Mg2+Mgx2l
So f1=μ(Mg2−Mgx2l)
and f2=μ(Mg2+Mgx2l)
F.B.D of M
f2−f1=Ma
μ.2Mgx2l=Ma=−d2xdt2.M
⇒d2xdt2=−μgxl
Time period =2π√lμg
Topper's Solved these Questions
Similar Questions
A uniform table of mas M stays horizontally and symmetrically on two wheels rotatig in opposite directions figure. The separsation between the wheels is L. The friction coefficeint between each whee, and the plate is μ. Find the time period of oscilationof the pate if it is slightly displaced along its length and released.
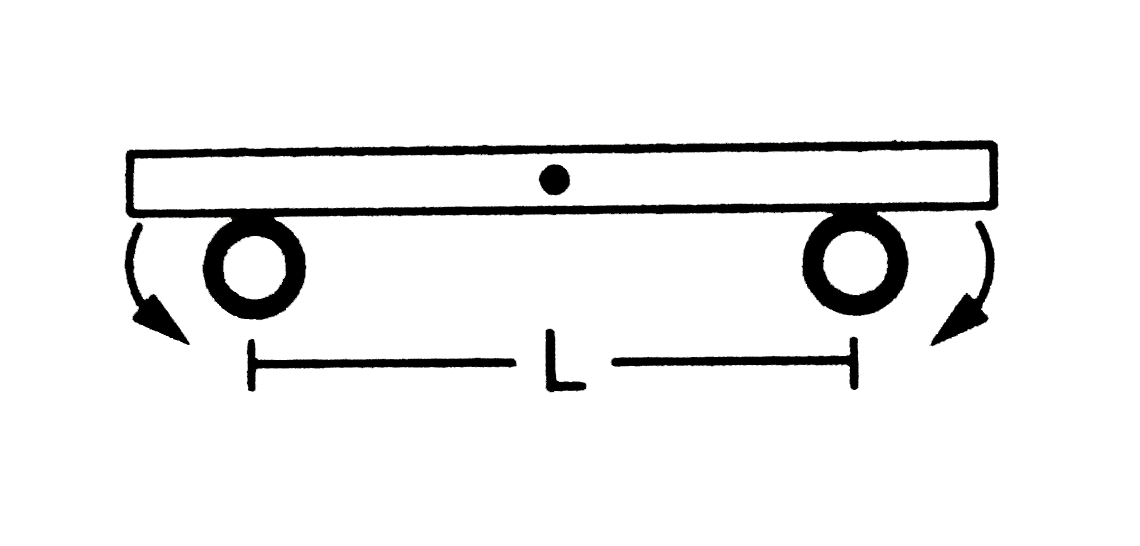
View SolutionA uniform plate of mass M stays horizontally symmetrically on two wheels rotating in opposite directions. The separation between the wheels is L. the friction coefficient between each and the plate is μ. Find the time period of oscillation of the plate if it is slightly displaced along its length and released.

View Solution
Knowledge Check
A pendulum of mass m and length L is connected to a spring as shown in figure. If the bob is displaced slightly from its mean position and released, it performs simple harmonic motion. The angular frequency of the oscillation of bob is
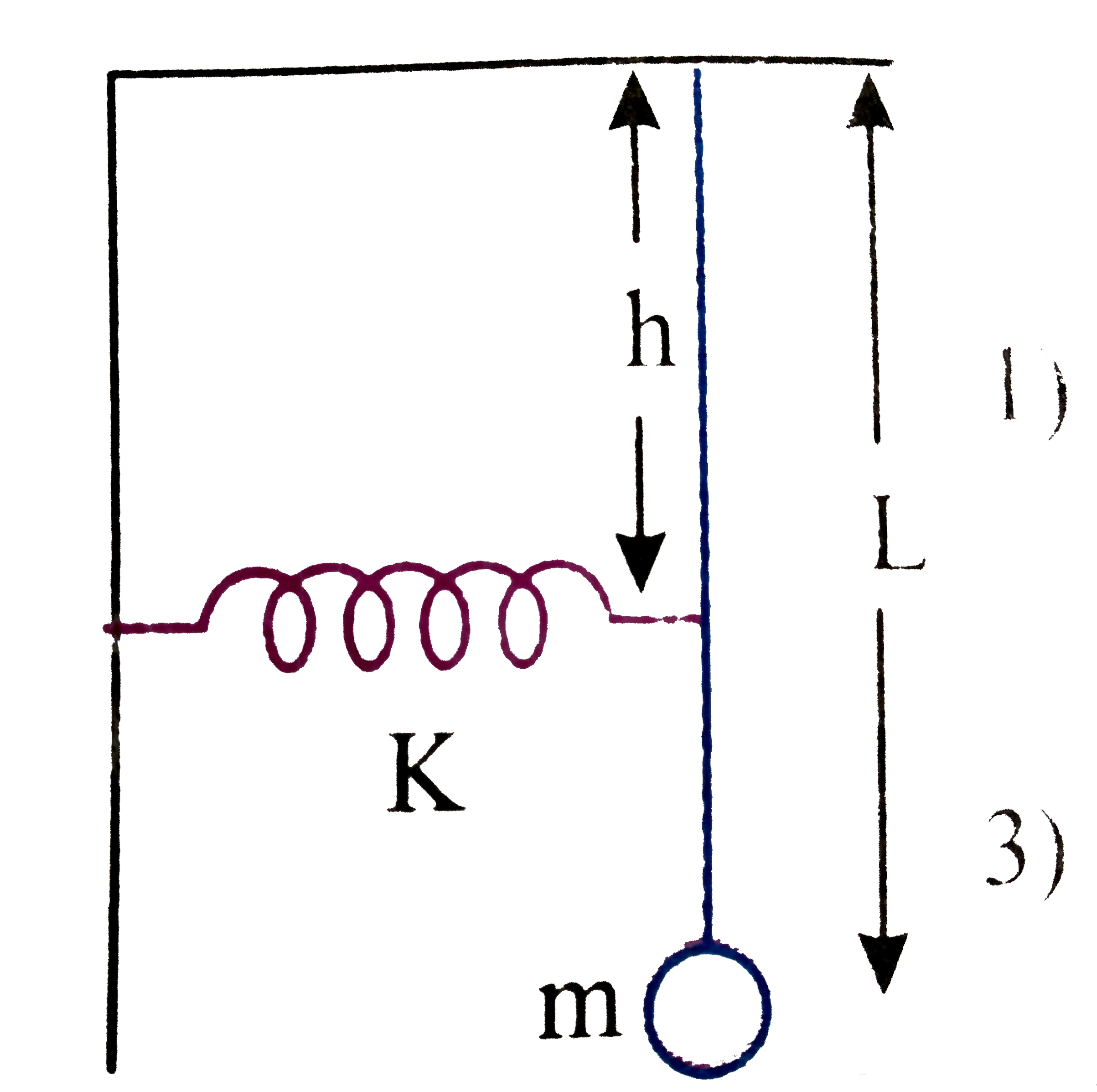 A√gLB√Kh2+mgLmL2C√3gLD√Kh2mL2
A√gLB√Kh2+mgLmL2C√3gLD√Kh2mL2A sphere of mass m is given some angular velocity about a horizontal axis through the center, and gently placed on a plank of mass m. The coefficient of friction between the two is μ. The plank rests on a smooth horizontal surface. The intial acceleration of the sphere relative to the plank will be:
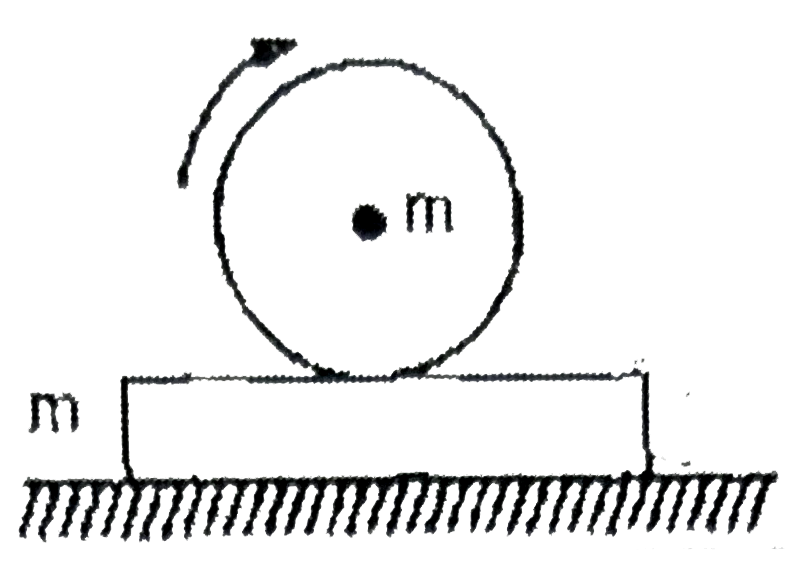 AzeroBμgC75μgD2μg
AzeroBμgC75μgD2μg
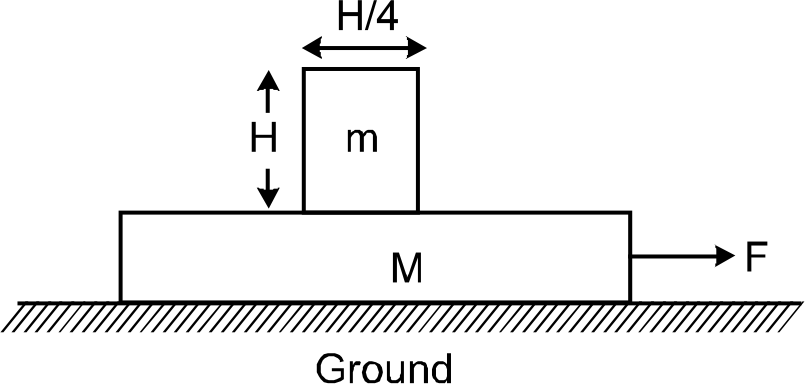
A block of mass m=2kg of shown dimensions is placed on a plank of mass M = 6Kg which is placed on smooth horizontal plane. The coefficient of friction between the block and the plank is μ=13. If a horizontal froce F is applied on the plank, then find the maximum value of F (in N) for which the block and the plank move together
View SolutionA plank rests symmetrically on two cylinders which are separated by a distance of 2l and rotate with uniform speed in opposite direction. If the plank is displaced a little and then released, show that the motion of the plank is simple harmonic. Calculate the period of oscillations of the peak. The co-efficient of friction between the plank and either cylinder is μ.
View SolutionA uniform horizontal plank is resting symmetrically in a horizontal position on two cylindrical droms , which are apinning in in opposite direction about their horizontal axes with equal angular velocity . The distance between the axes with equal angular velocity. The distance between the axes is 2L and the coefficient of friction between the plank and cylender is μ . If the plank is displaced slightly from the equilibrium position along its length and released, show that it performs simple horizontal motion. Caculate also the time period of motion.
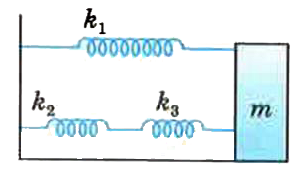
View SolutionThe figure shows an arrangement of mass m with three springs of different springs constant. When m is slightly displaced and then released, find the frequency of its motion.
View SolutionA uniform rod is placed on two spinning wheels as shown in figure. The axes of the wheels are separated by a distance l=20cm, the coefficient of friction between that in this case the rod performs harmonic oscillations. Find the period of these oscillations.

View SolutionComprehension A solid cylinder of mass mis rotating brought in contact with a plank of ma cylinder and plank because of which there is no slipping between cylinder and plank m is rotating with angular velocity foxy about its fixed axis of symmetry. It is at with a plank of mass m placed on a smooth om a smooth surface. There exist friction between se of which plank starts moving. After some time plank moves.such that in friction does the work m Rough surface 7772 ZEITEN Smooth surface The final velocity of the plank when there is no slipping between cylinder and plank is: (0) Ros Roo 3RW (A) 2Roo Roo (B) 4
View Solution