A particle of mass 'm' is moving in the x−y plane such that its x and y coordinate very according to the law x=asinωt and y=acosωt where 'a' and 'ω' are positive constants and 't' is time. Find
(a) equation of the path. Name the trajectory (path)
(b) whether the particle moves in clockwise or anticlockwise direction
magnitude of the force on the particle at any time t.
The correct Answer is:(a) x2+y2=a2, circle
(b) The particle moves in clock wise sense.
(C) The magnitude of force =m√a2x+a2y=mω2∂
(a) x2+y2=a2(sin2ωt+cos2(ωt))=a2
Hence the particle moves in a circle of radius 'a' with centre at origin.
(b) At t=0 sec, x=0 and y=+a. Hence the particle is at P as shown in figure.
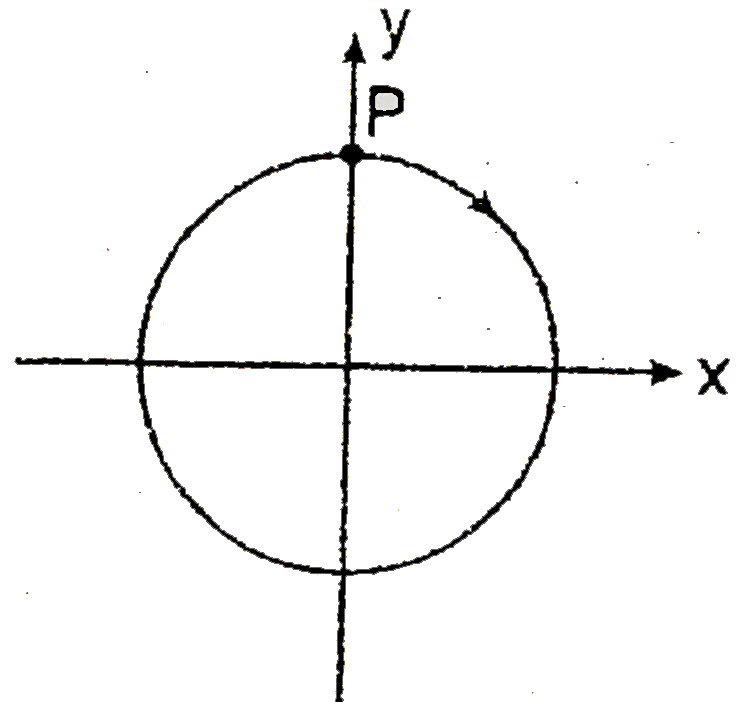
As 't' increases 'x' increases and 'y' decreases
∴ the particle moves in clock wise sense.
(c) The 'x' and 'y' compoenents of acceleration are
ax=d2xdt2=−ω2x,ay=d2ydt2=−ω2y
The magnitude of force =m√a2x+a2y=mω2a
Related Playlists
Similar Questions
A particle of mass 'm' is moving in the x-y plane such that its x and y coordinate vary according to the law x= a sin ωt and y= a cos ωt where 'a' and 'omega' are positive constants and 't' is time. Find
(a) equation of the path. Name the trajectory (path).
(b) whether the particle moves in clockwise or anticlockwise direction
(c) magnitude of the force on the particle at any time t.View SolutionA particle moves in xy plane according to the law x = a sin ωtand y = a(1 – cosωt) where a and ω are constants. The particle traces
View SolutionA point moves in the plane xy according to the law x=αsinωt,y=α(1−cosωt), where α and ω are positive constant and t is time. Find the distance traversed by point in time t0.
View SolutionA particle moves in Xy plane according to the law X=asinωtandy=a(1−cosωt) where a and ω are constants. The particle traces-
View SolutionA particle moves in the xy-plane according to the law x=asin(ωt)andy=a(1−cosωt) where 'a' and 'omega' are constants. Then the particle. follows :
View SolutionA point moves in the plane y according to the law x=Asinωt,y=Bcosωt, whereA,B&ω are positive constant.
The velocity of particle is given byView SolutionA point moves in the plane y according to the law x=Asinωt,y=Bcosωt, whereA,B&ω are positive constant.
The equation for trajectroy for path taken by particle isView SolutionFor a particle moving in the x-y plane, the x and y coordinates are changing as x=a sin ωt and y=a(1−cosωt), where 'a' and ω constants. Then, what can be inferred for the trajectory of the particle ?
View SolutionA particle moves in the XY-plane according to the law x=kt,y=kt(1−αt), where k and α are positive constants and t is time. The trajectory of the particle is
View SolutionA point moves in the plane y according to the law x=Asinωt,y=Bcosωt, whereA,B&ω are positive constant.
The acceleration of particle is given by [∵¯r is position vector]View Solution


