Draw a rough sketch of the given curve y=1+|x+1|,x=−3,x≡3,y=0 and find the area of the region bounded by them, using integration.
Video Solution
Text Solution
The correct Answer is:N/a
We have y=1+|x+1|,,x=-3,x =3 ,x=3 and y =0
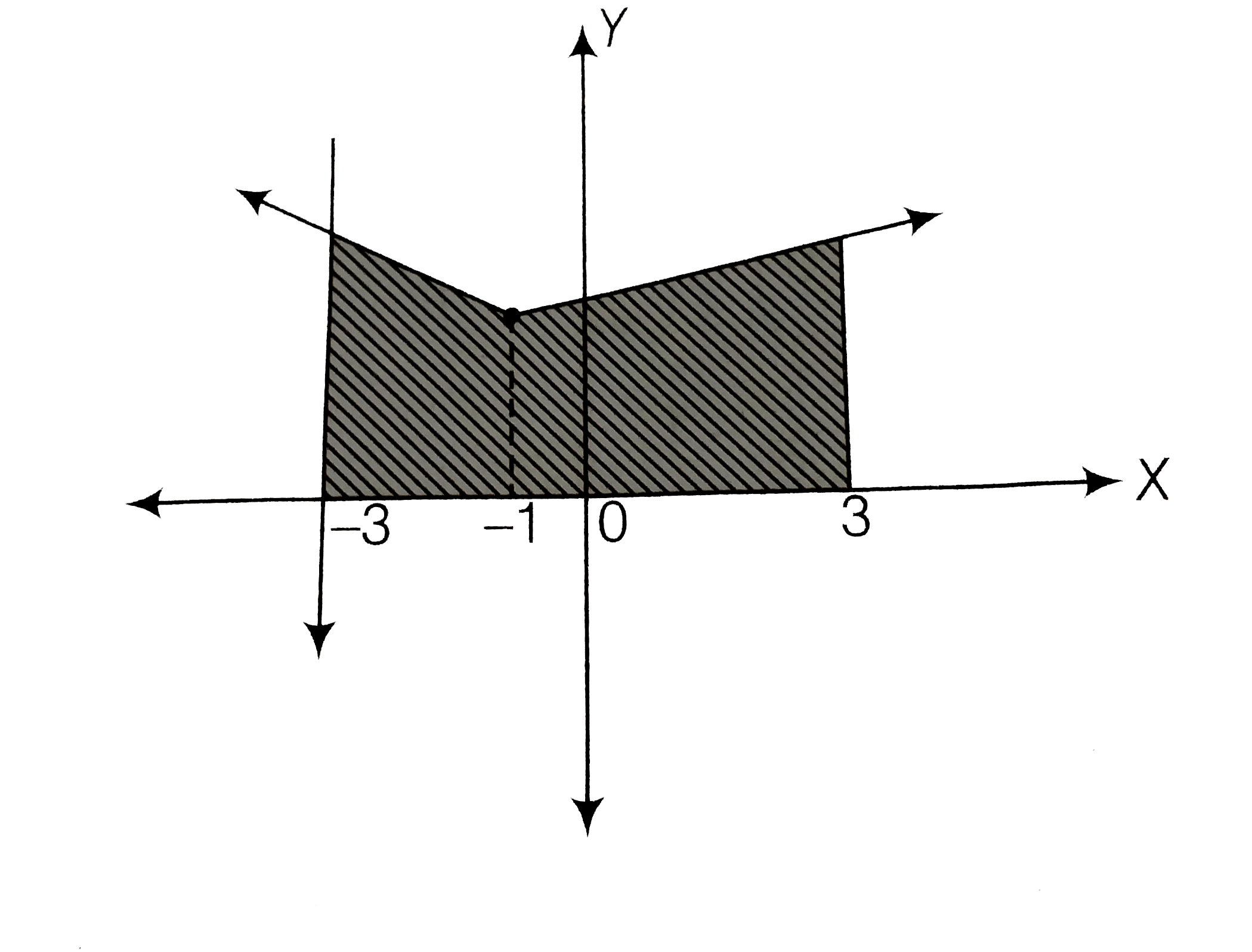
∴y={−xifx<−1x+2ifx≥−1
∴Area of shaded refion,A=∫−1−3−xdx+∫3−1(x+2)dx
=−[x22]−1+[x22+2x]3−1
≡[12−92]+[92+6−12+]
=−[−4]+[8+4]
=12+4 = 16 sq units
|
Topper's Solved these Questions
Similar Questions
Draw the rough sketch of the curve y=(x−1)2(x−3)3
View SolutionDraw a rough sketch of the curves y=sinxand y=cosx varies from 0 to π2 and find the area of the region enclosed by them and x-axis
View SolutionFind the area of the region bounded by the curves y=x2+2, y=x,x=0andx=3.
View SolutionDraw a rough sketch of the curves y2=x+1andy2=−x+1 and find the area enclosed between them,
View SolutionFind the area of the region bounded by the curves y=x2+2;y=x;x=0 and x=3
View SolutionFind the area of the region bounded by the curve y=x3,y=x+6 and x=0
View SolutionDraw the rough sketch of the curve y=(x−1)2(x−2)(x−3)3.
View SolutionFind the area of the region bounded by the curves y=x2+2y=x,x=0,andx=3.
View SolutionFind the area of the region bounded by y=−1,y=2,x=y3andx=0.
View SolutionFind the area of the region bounded by the curve |x|+|y|=1 and |x-1|+|y|=1
View Solution
