Bisectors of the angles B and C of an isosceles $Δ A B C$ with AB=AC intersect each other at 0.Show that external angle adejcent to $∠ A B C is equato ∠ B O C .$

Video Solution

Text Solution

Verified by Experts

Given $Δ A B C$ is an isoseceles triangle in which AB=AC,BO and CO are the bisectors of $∠ A B C and ∠ A C B$ respectively intersect at O.
To show $∠ D B A = ∠ B O C$
Construction Line CB Produced to D

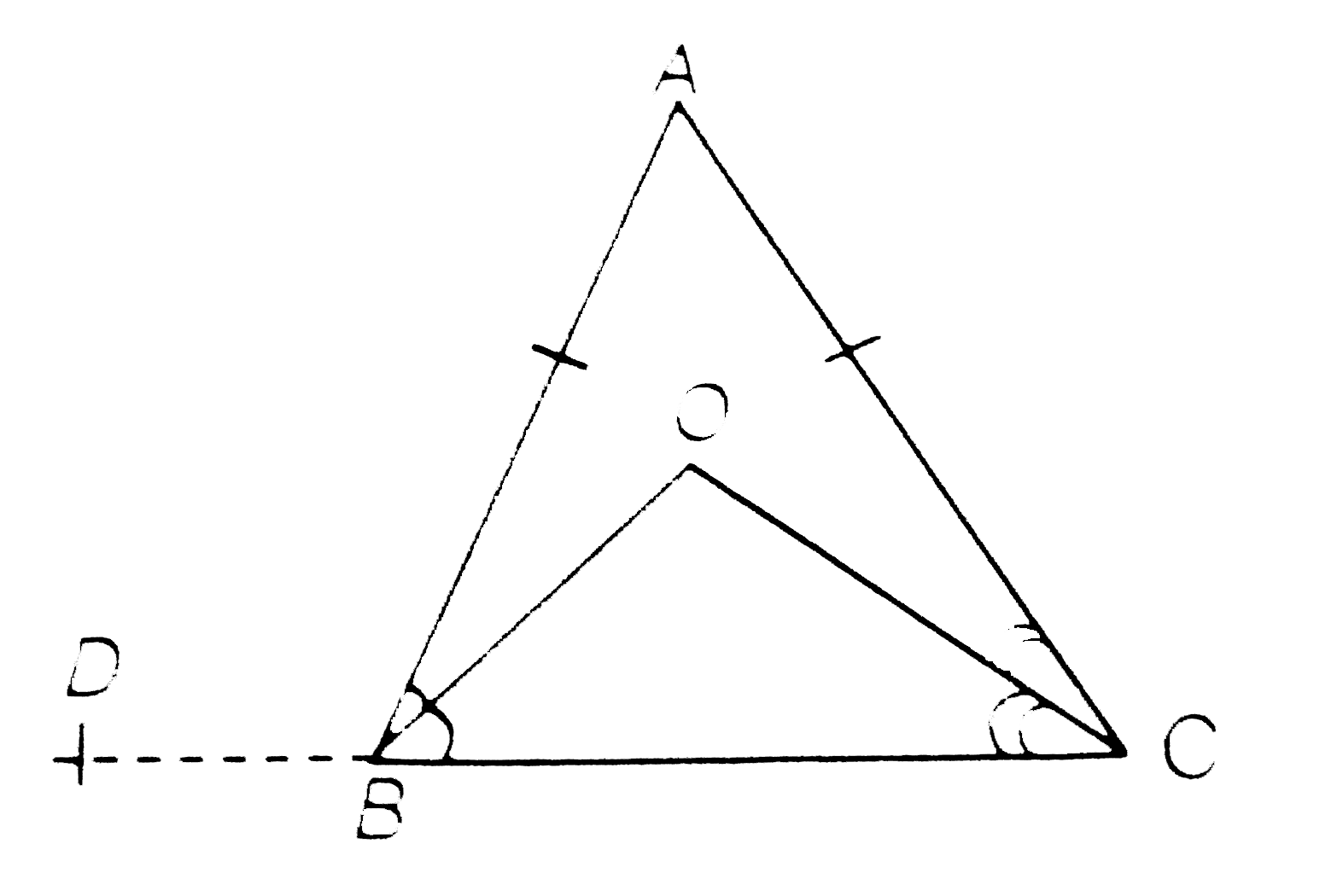
Proof in $Δ A B C A B = A C [given]$
$∠ A C B = ∠ A B C$
[angle opposite to equal sides are equal ]
$\Rightarrow \frac{1}{2} ∠ A C B = \frac{1}{2} ∠ A B C [on dividing both sides by 2]$
$\Rightarrow ∠ O C B = ∠ O B C ... . (i)$
[ $∵$ BO and CO are the bisectors of $∠ A B C and ∠ A C B$ ]
In $Δ B O C, ∠ O B C + ∠ O C B + ∠ B O C = 180^{\circ} [by angle sum propert of a triangle]$
$\Rightarrow ∠ O B C + ∠ O B C + ∠ B O C = 180^{\circ} [from Eq.(i)]$
$\Rightarrow 2 ∠ O B C + ∠ B O C = 180^{\circ}$
$\Rightarrow ∠ A B C + ∠ B O C = 180^{\circ} [∵ BO is the bisector of ∠ A B C]$
$\Rightarrow 180^{\circ} - ∠ D B A + ∠ B O C = 0$
$\Rightarrow ∠ D B A = ∠ B O C$

Updated on:21/07/2023

|

Class 9 MATHS TRIANGLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

In an isosceles $Δ A B C$ , if $A C = B C$ and $A B^{2} = 2 A C^{2}$ then $∠ C = ?$
A $30^{\circ}$
B $45^{\circ}$
C $60^{\circ}$
D $90^{\circ}$
If $△ A B C$ is an isosceles triangle with $∠ c = 90^{\circ}$ and AC = 5 cm then AB is:
A5 cm
B2.5 cm
C10 cm
D $5^{\sqrt{2}} c m$

NCERT EXEMPLAR-TRIANGLES-Triangles

Home
Ask Doubt
Profile