In a right triangle,Prove that the line-segment joining the mid-point of the hypotenuse to the opposite vertex is half the hypotenuse
Given In ΔABC,∠B=90∘ and D is the mid-point of AC
Construction produce BD to E such that BD =DE and join EC
To Prove
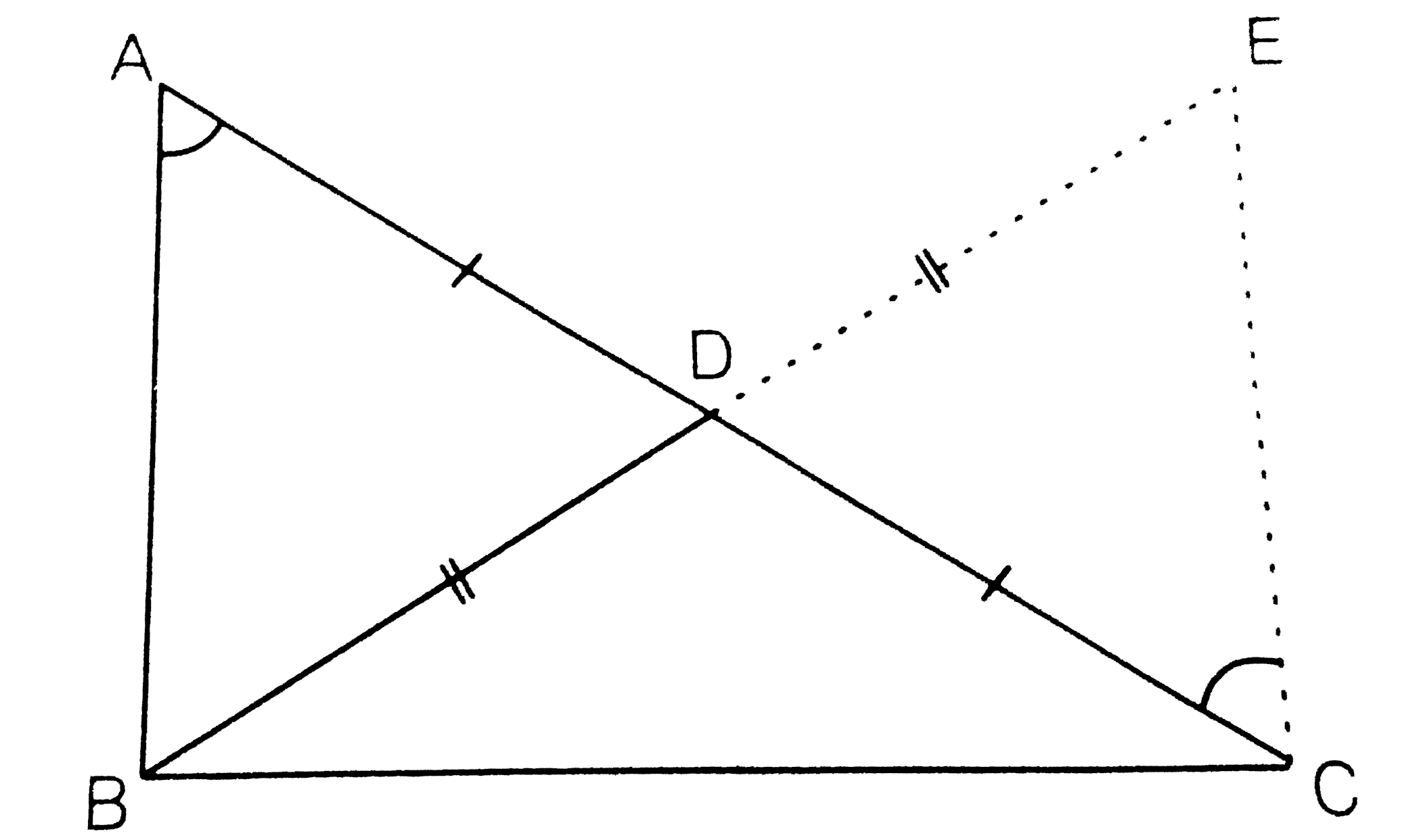
Proof In ΔADBandΔCDE AD=DC [∵D is mid-point ofAC]
BD =DE [by construction ]
and ∠ADB=∠CDE [vertically opposite angles ]
∴ΔADB≅ΔCDE [by SAS Congruence rule]
⇒AB=EC [by CPCT]
But ∠BADand∠DCE are alternate angles
So EC|| AB and BC is a transversal
∴∠ABC+∠BCE=180∘ [cointerior angles ]
⇒90∘+∠BCE=180∘ [∵∠ABC=90∘ given ]
⇒∠BCE=180∘−90∘
⇒∠BCE=90∘
In ΔABCandΔECB AB=EC [proved above]
BC=CB [common side]
and ∠ABC=∠ECB [each 90∘]
∴ΔABC≅ΔECB [by SAS congruence rule ]
⇒ AC=EB [by CPCT ]
⇒12EB=12AC [dividing both sides by 2]
⇒BD=12AC
Topper's Solved these Questions
Similar Questions
Knowledge Check
One side other than the hypotenuse of right angle isosceles triangle is 6 cm. The length of the perpendicular on the hypotenuse from the opposite vertex is :
A6 cmB6√2cmC4 cmD3√2cmOne side other than the hypotenuse of right angle isoceles triangle is 6 cm. The length of the perpendicular on the hypotenuse from the opposite vertex is :
A6 cmB6√2 cmC4 cmD3√2 cm
Prove that the line segment joining the middle points of two sides of a triangle is half the third side.
View SolutionProve that the line segment joining the mid points of two sides of a triangle is parallel to its third side.
View SolutionProve that the line segment joining the mid points of two side of a triangle is parallel to the third side and equal to half of it.
View SolutionIn order to prove, ''In a right angled triangle, the perpendicular segment to the hypotenuse from the opposite vertex, is the geometric mean of the segments into which the hypotenuse is divided."
(i) Draw a neat labelled figure.
(ii) Write 'Given' and 'To prove' from the figure drawn by you.
View SolutionProve that the line segment joining the mid-points of the diagonals of a trapezium is parallel to each of the parallel sides and is equal to half the difference of these sides.
View SolutionIn Fig. 14.40, a right triangle BOA is given. C is the mid-point of the hypotenuse AB . Show that it is equidistant from the vertices O, A and B . (FIGURE)
View Solution