Question

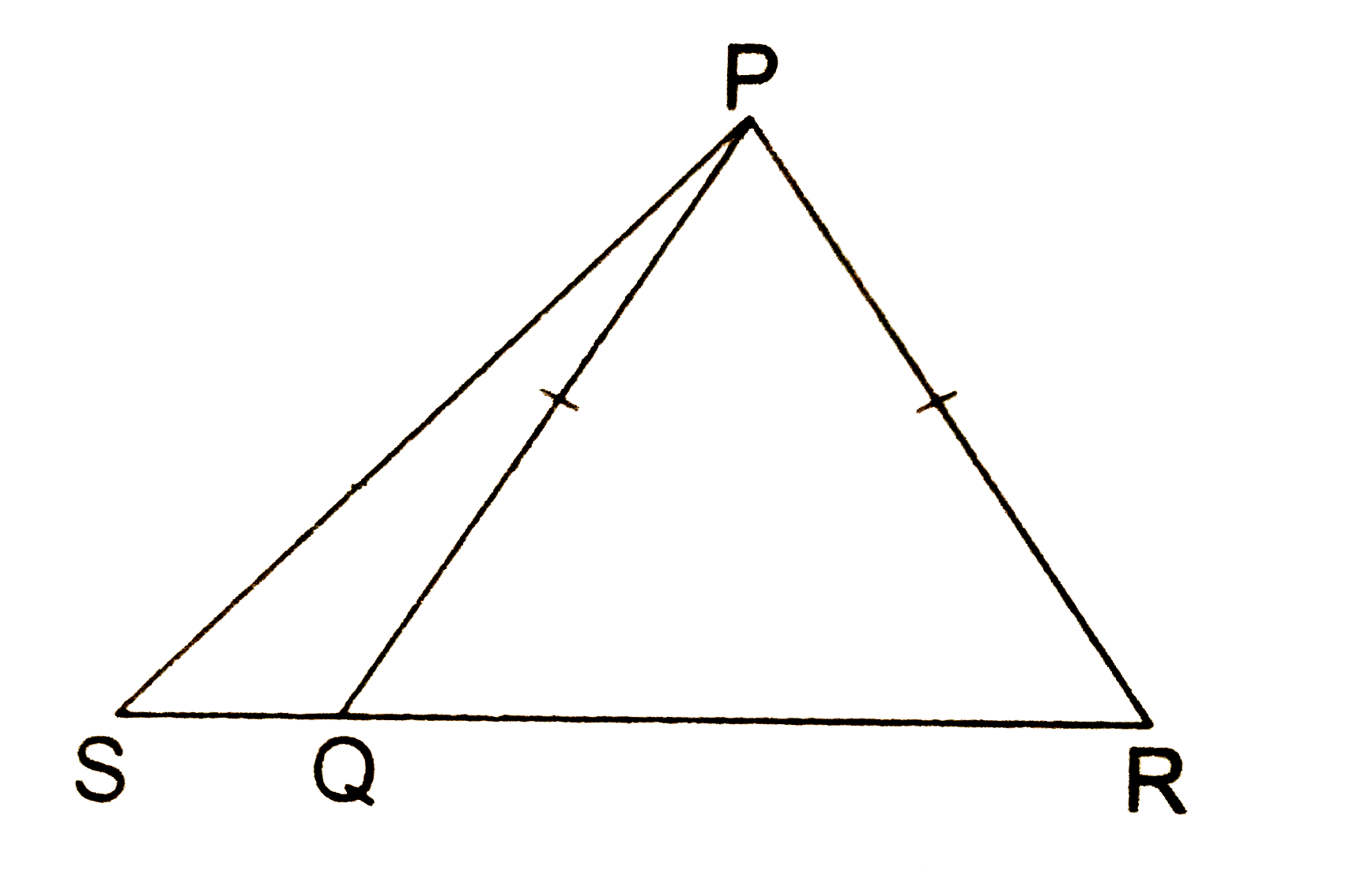
If S is a point on side PQ of a $\Delta PQR$ such that PS=QS=RS, then

Options

A. `PRcdotQR=RS^(2)`
B. `QS^(2)+RS^(2)=QR^(2)`
C. `PR^(2)+QR^(2)=PQ^(2)`
D. `PS^(2)+RS^(2)=PR^(2)`

Answer

C

Explanation

To solve the problem, we need to analyze the given triangle $$\Delta PQR$$ with point $$S$$ on side $$PQ$$ such that $$PS=QS=RS$$.

1. Draw the Triangle:
- Start by sketching triangle \( PQR \) with point \( S \) on side \( PQ \).

2. Label the Segments:
- Mark the lengths such that \( PS = QS = RS = x \) (where \( x \) is some length).

3. Identify Isosceles Triangles:
- Notice that triangle \( PSR \) is isosceles because \( PS = RS \).
- Similarly, triangle \( QSR \) is also isosceles because \( QS = RS \).

4. Apply the Isosceles Triangle Property:
- In triangle \( PSR \), since \( PS = RS \), the angles opposite these sides are equal:
- Let \( \angle 1 = \angle P \).
- In triangle \( QSR \), since \( QS = RS \), the angles opposite these sides are equal:
- Let \( \angle 2 = \angle Q \).

5. Use the Angle Sum Property of Triangle:
- For triangle \( PQR \), the sum of the angles is:
\( \angle P + \angle Q + \angle R = 180^\circ \)
- Substitute \( \angle P \) and \( \angle Q \) with \( \angle 1 \) and \( \angle 2 \):
\( \angle 1 + \angle 2 + \angle R = 180^\circ \)

6. Express \( \angle R \):
- From the previous step, we can express \( \angle R \) as:
\( \angle R = 180^\circ - (\angle 1 + \angle 2) \)

7. Substituting Values:
- Since \( \angle 1 = \angle P \) and \( \angle 2 = \angle Q \), we can write:
\( \angle R = 180^\circ - (\angle P + \angle Q) \)
- This implies that \( \angle R = 90^\circ \).

8. Conclusion:
- Since \( \angle R = 90^\circ \), triangle \( PQR \) is a right triangle.
- By the Pythagorean theorem, we have:
\( PQ^2 = PR^2 + QR^2 \)

Final Result:
The relationship established is that \( PQ^2 = PR^2 + QR^2 \).