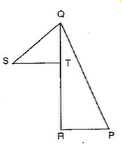
In the given figure, Q is a point on the side SR of $Δ P S R$ such that PQ = PR. Prove that $P S > P Q .$

Video Solution

Text Solution

Verified by Experts

We know that the angle opposite to equal sides are equal.
$∴ P Q = P R \Rightarrow ∠ P Q R = ∠ P R Q .$
In $Δ P S Q,$ the side SQ has been produced to R.
$∴ ∠ P Q R > ∠ P S Q [exterior angle is greater than int. opp. ∠ s]$
$\Rightarrow ∠ P R Q > ∠ P S Q [using (i)]$
$\Rightarrow ∠ P R S > ∠ P S R [∵ ∠ P R Q = ∠ P R S and ∠ P S Q = ∠ P S R]$
$\Rightarrow P S > P R$
$\Rightarrow P S > P Q [∵ P Q = P R] .$
Hence, $P S > P Q .$

Updated on:21/07/2023

|

Class 9 MATHS CONGRUENCE OF TRIANGLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

If S is a point on side PQ of a $Δ P Q R$ such that PS=QS=RS, then
A $P R \cdot Q R = R S^{2}$
B $Q S^{2} + R S^{2} = Q R^{2}$
C $P R^{2} + Q R^{2} = P Q^{2}$
D $P S^{2} + R S^{2} = P R^{2}$

RS AGGARWAL-CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLE-Multiple Choice Questions (Mcq)

Home
Ask Doubt
Profile