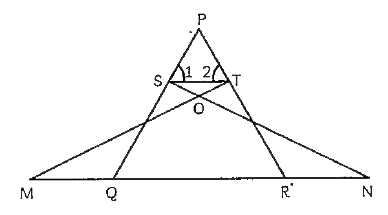
In figure, if ∠1=∠2 and ΔNSQ=ΔMTR, then prove that ΔPTS~ΔPRQ.
Video Solution
Text Solution
Given ΔNSQ≅ΔMTRand∠1=∠2
to prove ΔPTS~ΔPRQ
Proof Since, ΔNSQ≅ΔMTR
So, SQ=TR…..(i)
Also, ∠1=∠2⇒PT=PS..(ii)
[since, sides opposite to equal angles are also equal]
From Eqs. (i) and (ii) PSSQ=PTTR
⇒ST||QR [by convense of basic proportionality theorem]
∴∠1=∠PQR
and ∠2=∠PRQ
in ΔPTSandΔPRQ [common angles]
∠P=∠P
∠1=∠PQR
∠2=∠PRQ
∴ΔPTS~ΔPRQ [by AAA similarity criterion]
Hence proved
|
Topper's Solved these Questions
Similar Questions
In the given figure, figure, if ∠1=∠2 and △NSQ≅△MTR, then prove that △PTS~△PRQ.
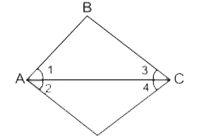
View SolutionIn the given figure, ∠1=∠2andACBD=CBCE prove that ΔACB~ΔDCE.
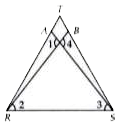
View SolutionIn figure, it is given that RT=TS,∠1=∠4and∠3=∠2. Prove that ΔRBT≅ΔSAT.
View SolutionIn figure it is given that BC=CEand∠1=∠2. Prove that ΔGCB≅ΔDCE.

View SolutionIn the given figure ∠1=∠2 and ∠2=∠3
then show that ∠1=∠3
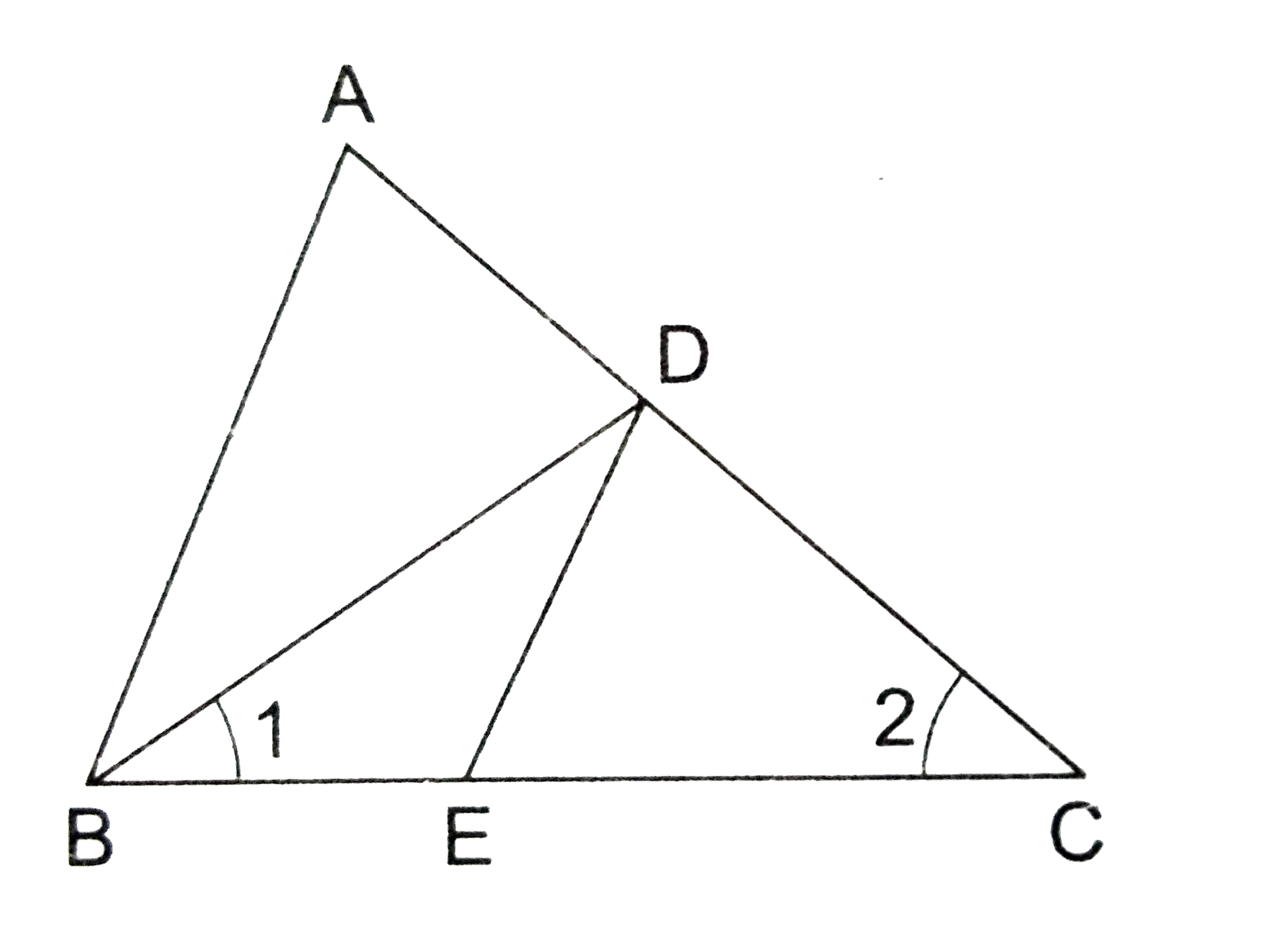
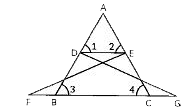
View SolutionIn the given figure, ΔFEC is congruent to ΔGDB and ∠1=∠2. Prove that ΔADE~ΔABC
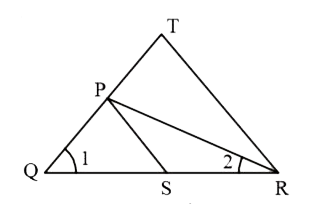
View SolutionIn the given figure, QRQS=QTPRand∠1=∠2 then prove that △PQS~△TQR.
View SolutionIn the adjoining figure, we have ∠1=∠2 and ∠2=∠3. Show that ∠1=∠3.

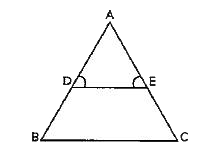
View SolutionIn the figure, ∠D=∠EandADDB=AEEC, prove that ΔBAC is an isosceles triangle
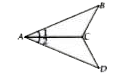
View SolutionIn the given figure, AB = AD and ∠1=∠2. Prove that : ΔABC≅ΔADC
View Solution
