Diagonals AC and BD of a quadrilateral ABCD intersect at O in such a way that ar(AOD)=ar(BOC) . Prove that ABCD is a trapezium.
Given: ABCD is a quadrilateral where diagonals AC and BD intersect at O.
⇒ar(△AOD)=ar(△BOC)
Adding ar(△ODC) on both sides,
⇒ar(△AOD)+ar(△ODC)=ar(△BOC)+ar(△ODC)
⇒ar(△ADC)=ar(△BDC)
Now, △ADC and △BDC lie on the same base DC and are equal in area and they lie between the lines AB and DC
⇒AB parallel DC (Two triangles having the same base and equal areas lie between the same parallels)
In ABCD,
⇒AB parallel to DC
So, one pair of opposite sides is parallel,
∴ABCD is trapezium.
Hence Proved.
Topper's Solved these Questions
Similar Questions
Diagonals AC and BD of a quadrilateral ABCD intersect at O in such a way that ar (AOD)=ar ( BOC). Prove that ABCD is a trapezium.
View SolutionDiagonals AC and BD of a quadrilateral ABCD intersect each at P. Show That: ar(APB)×ar(CPD)=ar(APD)×ar(PBC)
View SolutionIn Figure, ABCD is a trapezium in which AB∣∣CD . Prove that: ar(AOD)=ar(BOC).
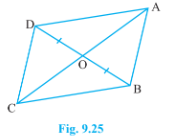
View SolutionIn Fig. 9.25, diagonals AC and BD of quadrilateral ABCD intersect at O such that OB = OD. If AB = CD, then show that:(i) ar (DOC) = ar (AOB)(ii) ar (DCB) = ar (ACB)(iii) D A\ ||\ C
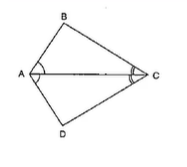
View SolutionIn Figure, diagonal AC of a quadrilateral ABCD bisects the angles A and C . Prove that AB=AD and CB=CD
View SolutionDiagonals AC and BD of a quadrilateral ABCD intersect each other at P. Show thatar (APB) × ar (CPD) = ar (APD) × ar (BPC).
View SolutionIf the diagonals AC,BD of a quadrilateral ABCD, intersect at O, and seqarate the quadrilateral into four triangles of equal area, show that quadrilateral ABCD is a parallelogram. GIVEN : A quadrilateral ABCD such that its diagonals AC and BD intersect at O and separate it into four parts such that ar(AOB)=ar(BOC)=ar(COD)=ar(AOD) TO PROVE : Quadrilateral ABCD is a parallelogram.
View SolutionThe diagonals of a parallelogram ABCD intersect at OA line through O intersects AB at XandDC at Y Prove that OX=OY
View SolutionIf the bisectors of two adjacent angles A and B of a quadrilateral ABCD intersect at a point O such that ∠C+∠D=k∠AOB, then find the value of k
View SolutionThe diagonals of quadrilateral ABCD,ACandBD intersect in O. Prove that if BO=OD, the triangles ABCandADC are equal in area.
View Solution