Triangles having equal areas and having one side of one of the triangles, equal to one side of the other, have their corresponding altitudes equal. GIVEN : Two triangles ABC and PQR such that: ar(ABC)=AR(PQR) (ii)AB=PQ
Given : Two triangles ABC and PQR such that (i) ar(ΔABC)=ar(ΔPQR) and (ii) AB=PQ.
CN and RT and the altitude corresponding to AB and PQ respectively of the two triangles.
To prove: CR=RT
Proof: In ΔABC,
CN is the altitude corresponding to the side AB.
ar(ΔABC)=12AB×CN ....(i)
Similarly, ar(ΔPQR)=12PQ×RT ....(ii)
From equation (i) and (ii), we get:
ar(ΔABC)=ar(ΔPQR) [Given]
∴12AB×CN=12PQ×RT
Also, AB=PQ [Given]
CN=RT
Hence proved.
Topper's Solved these Questions
Similar Questions
Triangles having equal areas and having one side of one of the triangle; equal to one side of the other; have their corresponding altitudes equal.
View SolutionIn two right triangles one side an acute angle of one are equal to the corresponding side and angle of the other. Prove that the triangles are congruent.
Let ABC and DEF be two right triangles such that
∠A=∠D,BC=EFand∠B=∠E=900
Thus, in △s ABC and DEF<br>/_A=/_D<br>/_B=/_E=90^0<br>BC=EFSo,by∀S≅ruencecriterion,weobta∈<br>/_\ABC~=/_\DEF
View Solution
Knowledge Check
If in two right triangles, the hypotenuse and one side of one triangle are equal to the hypotenuse and one side of the other triangle, then the two triangles are congruent. 1.
PL is an altitude from P of ΔPQR on QR such that QL = LR. Then,A∠Q<∠RB∠Q=∠RC∠Q>∠RD∠P=∠R{(p∧(p→q))∧(q→r)}→r is equal to
Aq→~rBp→~rCfallacyDtautology
Consider the following statements : Two triangles are said to be congruent, if
1. Three angles of one triangle are equal to the corresponding three angles of the other triangle.
2. Three sides of one triangle are equal to the corresponding three sides of the other triangle,
3. Two sides and the included angle of one triangle are equal to the corresponding two sides and the included angle of the other triangle.
4. Two angles and the included side of one triangle are equal to the corresponding two angles and the included side of the other triangle.
Which of the above statements are correct ?
View SolutionConsider a triangle PQR having sides of lengths p,q,r opposite to the angles P,Q,R respectively. Then which of the following statements is (are) true?
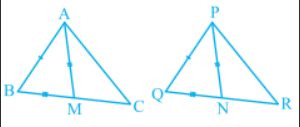
View SolutionIn Figure, two sides AB and BC and the median AD of ABC are equal respectively to the two sides PQ and QR and the median PM of the other triangle PQR. Prove that ABD≅PQM (ii) ABC ≅ PQR
View SolutionThe sides AB,BC and AC of a triangle ABC touch a circle with centre O and radius r at P, Q, and R respectively.
View SolutionTriangles on the same base and between the same parallels are equal in area. GIVEN : Two triangles ABC and PBC on the same base BC and between the same parallel lines BC and AP. TO PROVE : ar(ABC)=ar(PBC) CONSTRUCTION : Through B, draw BDCA intersecting PA produced in D and through C, draw CQBP, intersecting line AP in Q.
View SolutionIf in a triangle of base 'a', the ratio of the other two sides is r ( <1).Show that the altitude of the triangle is less than or equal to ar1−r2
View Solution
