A diagonal of parallelogram divides it into two congruent triangles.

Suppose ABCD is parallelogram and BD is the diagonal, then there are 2 triangles;
△ABDand△CBD
AB=CDandAD=BC(opposite sides of a parallelogram are equal)
BD is the Common Side
∴△ABD≅△CBD( By SSS Congruency)
hence the given statement is true
Topper's Solved these Questions
Similar Questions
Knowledge Check
Prove that each of the following diagonals of a parallelogram divides it into two congurent triangles. The following lowing are the steps involved in proving the above results. Arrange them in sequential order.
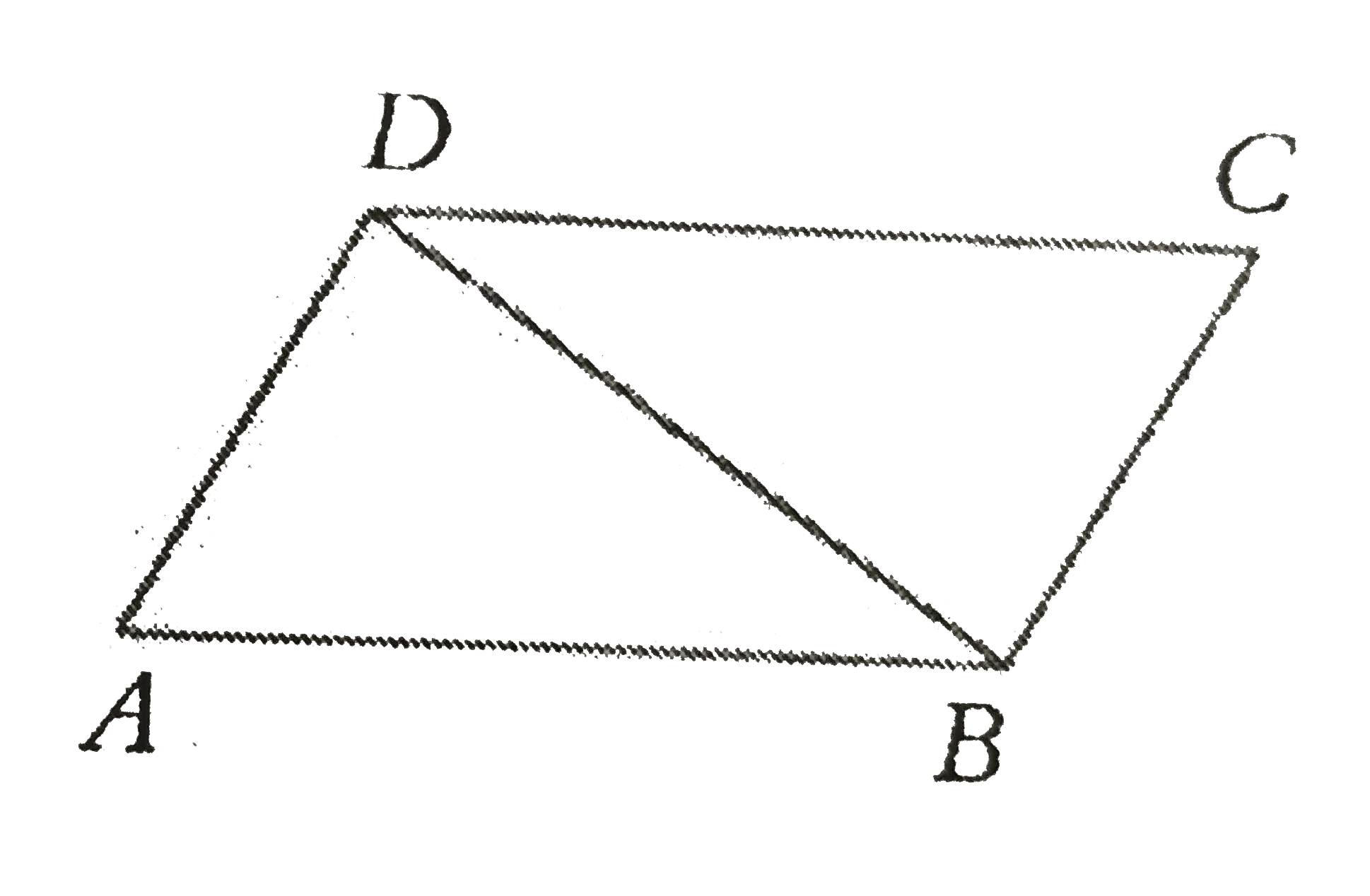
(A) By SSS conguruence property , ΔDAB≅ΔBCD.
(B) Let ABCD be a parallelogram and join BD.
(C ) AB=CD,AD=BC(opposite sides of parallelogram) and BD=BD (common side).
(D ) Similarly , AC divides the parallelogram into two congruent triangles.AABCDBBCADCBACDDCBADShow that each diagonal of a parallelogram divide it into two congruent triangles.
The following are the steps involved in showing the above result. Arrange them in sequential order.
A) In △ABC and △CDA, AB=DC and BC=AD (∴ opposite angles of parallelogram) AC=AC (common side).
B) Let ABCD be a parallelogram. Join AC.
C) By SSS congruence property, △ABC≅△CDA.
D) Similarly, BD divides the triangle into two congruent triangles.ABACDBBDACCBADCDBDCA
Prove that each of the following diagonals of a parallelogram divides it into two congurent triangles. The following lowing are the steps involved in proving the above results. Arrange them in sequential order.

(A) By SSS conguruence property , ΔDAB≅ΔBCD.
(B) Let ABCD be a parallelogram and join BD.
(C ) AB=CD,AD=BC(opposite sides of parallelogram) and BD=BD (common side).
(D ) Similarly , AC divides the parallelogram into two congruent triangles.
View SolutionA diagonal of a parallelogram divides it into two triangles of equal area.
View SolutionA diagonal of a parallelogram divides it into two triangles of equal area. GIVEN : A parallelogram ABCD in which BD is one of the diagonals. TO PROVE : ar (ABD)=ar(CDB)
View SolutionShow that the diagonals of a parallelogram divide it into four triangles of equal area.
View SolutionShow that the diagonals of a parallelogram divide it into four triangles of equal area.
View SolutionShow that the diagonals of a parallelogram divide it into four triangles of equal area.
View Solution
