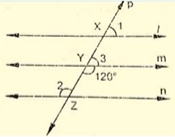
l, m and n are
three parallel lines intersected by transversals p and q such
that l, m
and n cut off equal
intercepts AB and BC on p (see Fig. 8.28). Show that l, m and
n cut off equal intercepts DE and EF on q also
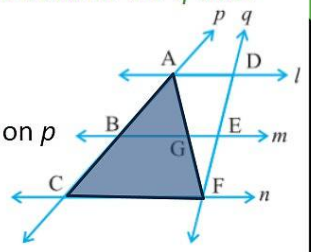
Given:AB=BC
To prove:DE=EF
Construction: Join AF intersecting m at G.
Now the trapezium ACFD is divided into two triangles: ΔACFandΔAFD.
In ΔACF,B is the mid point of AC and BG∣∣CF (as m || n )
∴ By mid point theorem, G is the mid point of AF.
In ΔAFD,G is the mid point of AFandGE∣∣AD (as l || m)
⇒E is the mid point of DF (by mid point theorem)
⇒ ∴DE=EF
Topper's Solved these Questions
Similar Questions
Knowledge Check
Two parallel line l and m are intersected by a transversal p. The quadrilateral formed by the bisectors of interior angles is a
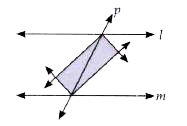 ATrapeziumBKiteCParallelogramDSquare
ATrapeziumBKiteCParallelogramDSquare
l and m are two parallel lines intersected by another pair of parallel lines p and q. Show that △ABC≅△CDA.
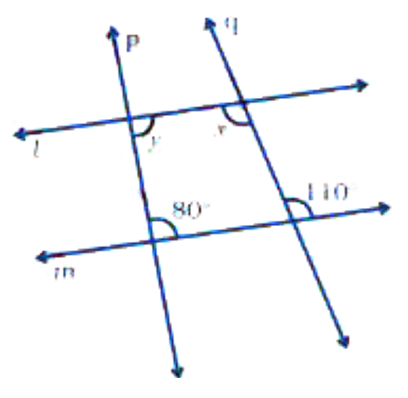
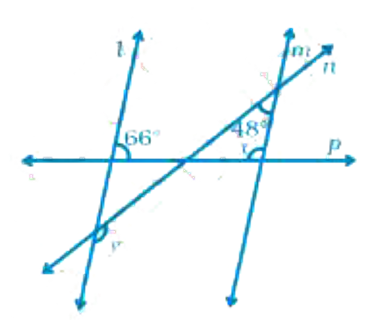
View SolutionIn Fig.5.66 , two parallel lines l and m are cut by two transversals p and q . Determine the values of x and y .
View SolutionIn Fig. 60, AB and CD are parallel lines intersected by a transversal PQ at L and M respectively. If ∠LMD=350 find ∠PLA.
View SolutionIn Fig. 5.60, two parallel lines l and m are cut by two transversals n and p. Find the values of x and y.
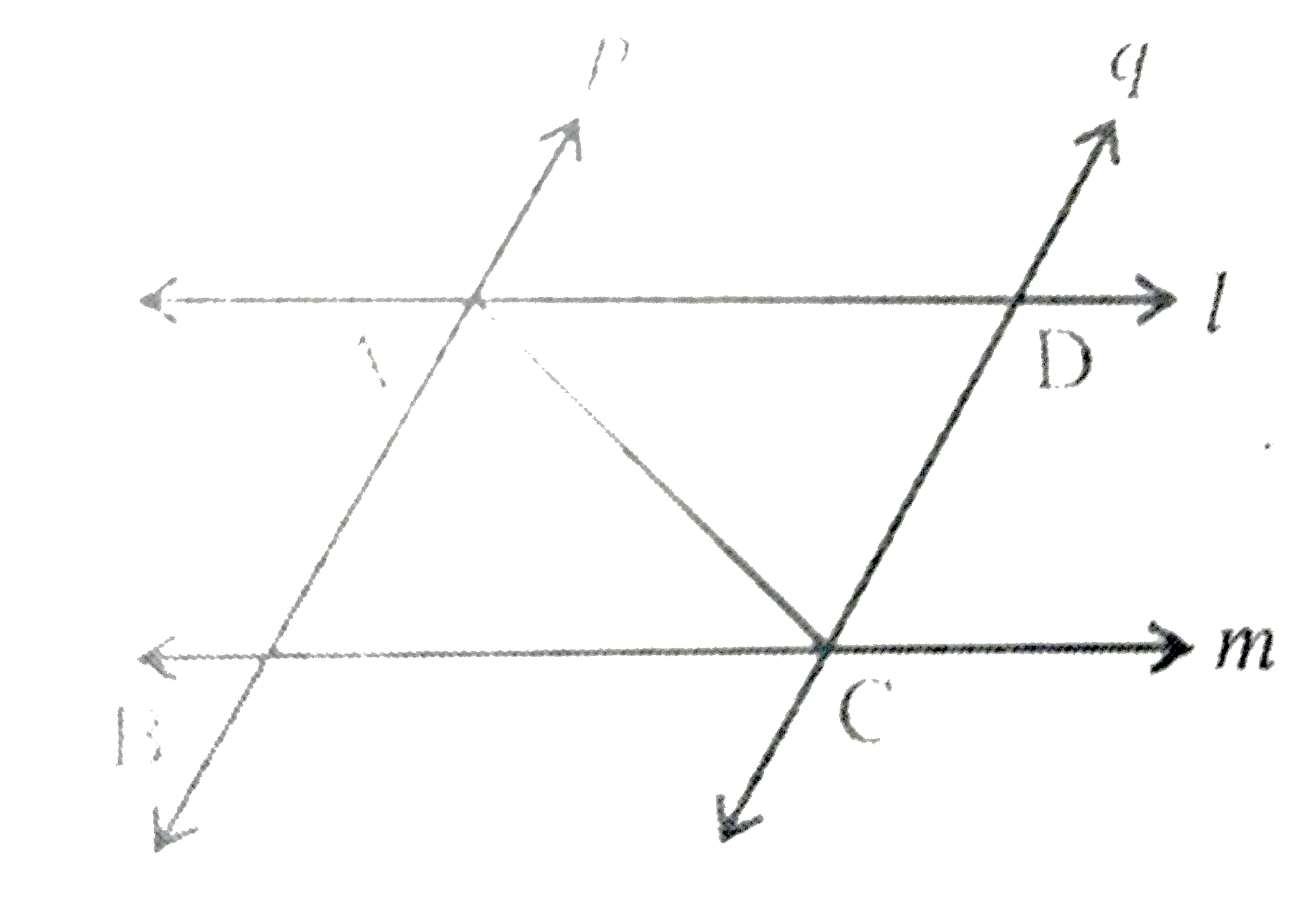
View Solutionl and m are two parallel lines intersected by another pair of parallel lines p and q (see Fig. 7.19). Show that ΔABC≅ΔCDA.
View Solutionl and m are two parallel lines intersected by another pair of parallel lines p and q as shown in figure. Show that ABC≅CDA.
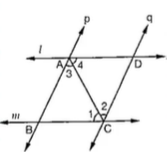
View SolutionIn thg given figure, two parallel lines l and m are intersected by two parallel lines p and q.
Show that ΔABC≅ΔCDA.
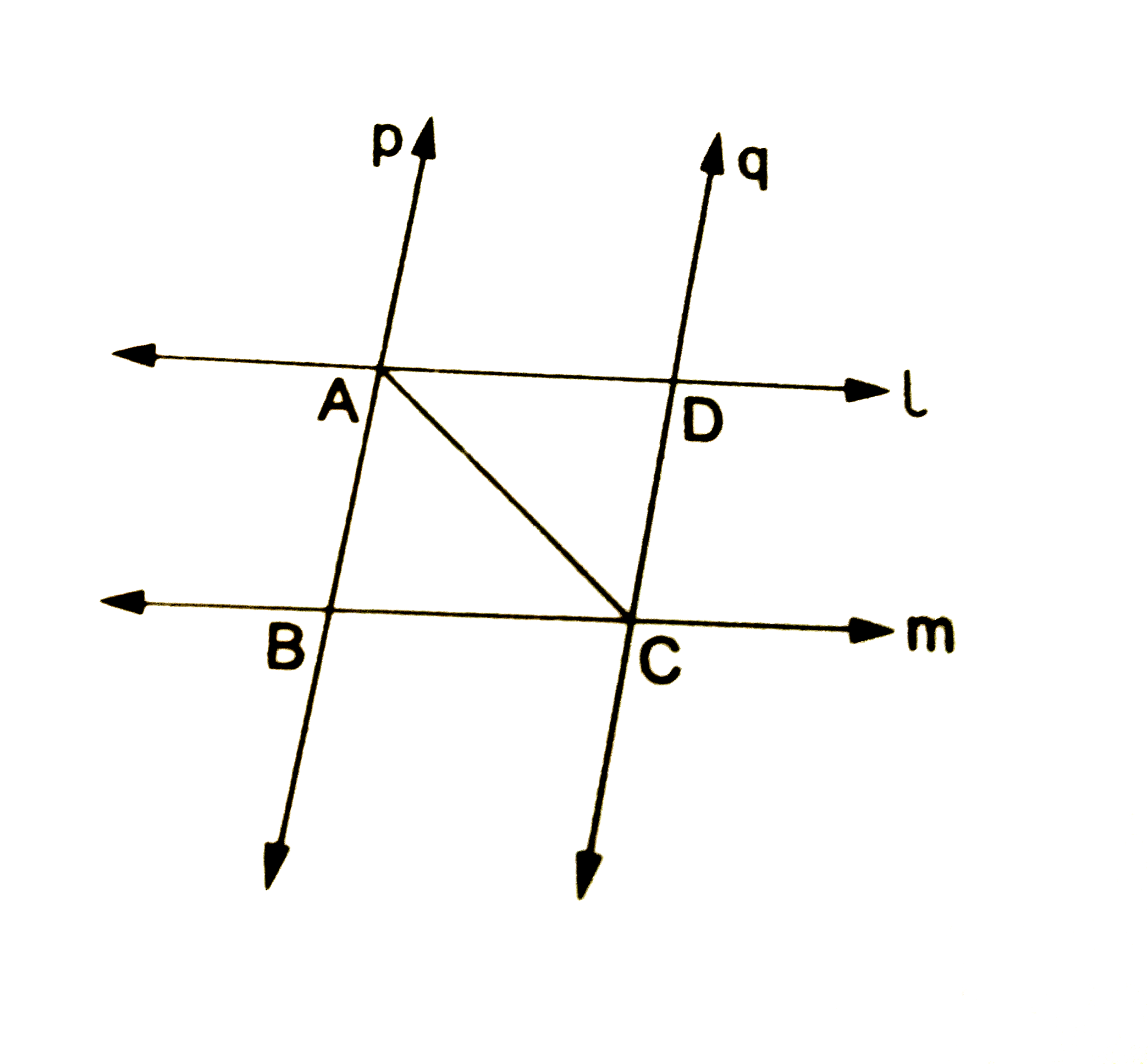
View Solution