A parallel plate capacitor has square plates of side length L kept at a separation d. The space between them is filled with a dielectric whose dielectric constant changes as K=eβx where x is distance measured from the left plate towards the right plate, and b is a positive constant. A poten- tial difference of V volt is applied with left plate positive.
(i) What happens to capacitance of the capacitor if d is increased ? What is the smallest possible capacitance that can be obtained by changing d ?
(ii) Write the expression of electric field between the plates as a function of x.
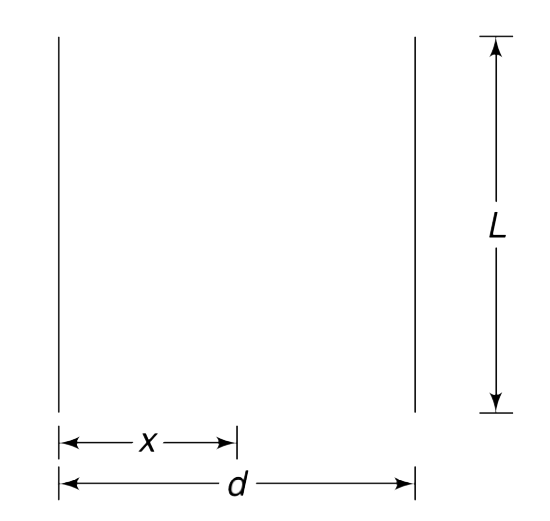
The correct Answer is:(i) Capacitance decreases, Cmin=∈0L2β
(iii) E=βVe−βx1−e=−βd
Step by step video, text & image solution for A parallel plate capacitor has square plates of side length L kept at a separation d. The space between them is filled with a dielectric whose dielectric constant changes as K = e^(betax) where x is distance measured from the left plate towards the right plate, and b is a positive constant. A poten- tial difference of V volt is applied with left plate positive. (i) What happens to capacitance of the capacitor if d is increased ? What is the smallest possible capacitance that can be obtained by changing d ? (ii) Write the expression of electric field between the plates as a function of x. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A parallel plate capacitor has square plates of area A separated by distance ‘d’ between them. It is filled with a dielectric which has a dielectric constant that varies as k(y)=K0(1+βy) where 'y' is the distance measured from bottom of capacitor. The total capacitance of the system is given by the expression:
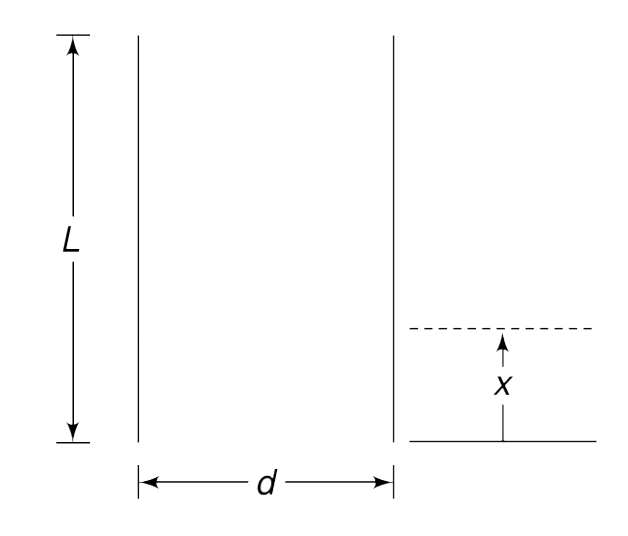
View SolutionA parallel plate capacitor has square plates of side length L. Plates are kept vertical at separation d between them. The space between the plates is filled with a dielectric whose dielectric constant (K) changes with height (x) from the lower edge of the plates as K=eβx where b is a positive constant. A potential difference of V is applied across the capacitor plates.
(i) Plot the variation of surface charge density (s) on the positive plate of the capacitor versus x.
(ii) Plot the variation of electric field between the plates as a function of x.
(iii) Calculate the capacitance of the capacitor.
View Solution
Knowledge Check
A parallel plate capacitor has plate area A and plate separation d. The space between the plates is filled up to a thickness x<d with a dielectric constant K. Calculate the capacitance of the system.
 A2KεAKd−x(K−1)BKεAK2d−x(K−1)CKεAK−x(K−1)DKεAKd−x(K−1)
A2KεAKd−x(K−1)BKεAK2d−x(K−1)CKεAK−x(K−1)DKεAKd−x(K−1)A parallel plate capacitor has plates of area A separated by distance ‘d’ between them. It is filled with a dielectric which has a dielectric constant that varies as k(x)=K(1+ax) where ‘x’ is shown in figure. If (al)<<1 , the total capacitance of the system is best given by the expression:
 AAK∈0d(1+αl)BAk∈0d(1+αl2)CA∈0Kd(1+(αl2)2)DA∈0Kd(1+α2l22)
AAK∈0d(1+αl)BAk∈0d(1+αl2)CA∈0Kd(1+(αl2)2)DA∈0Kd(1+α2l22)A parallel plate air capacitor has capacitance C. Half of space between the plates is filled with dielectric of dielectric constant K as shown in figure . The new capacitance is C . Then
 AC=C[KK+1]BC'=C[2KK+1]CC'=2CK+1DC'=C[1+K2]
AC=C[KK+1]BC'=C[2KK+1]CC'=2CK+1DC'=C[1+K2]
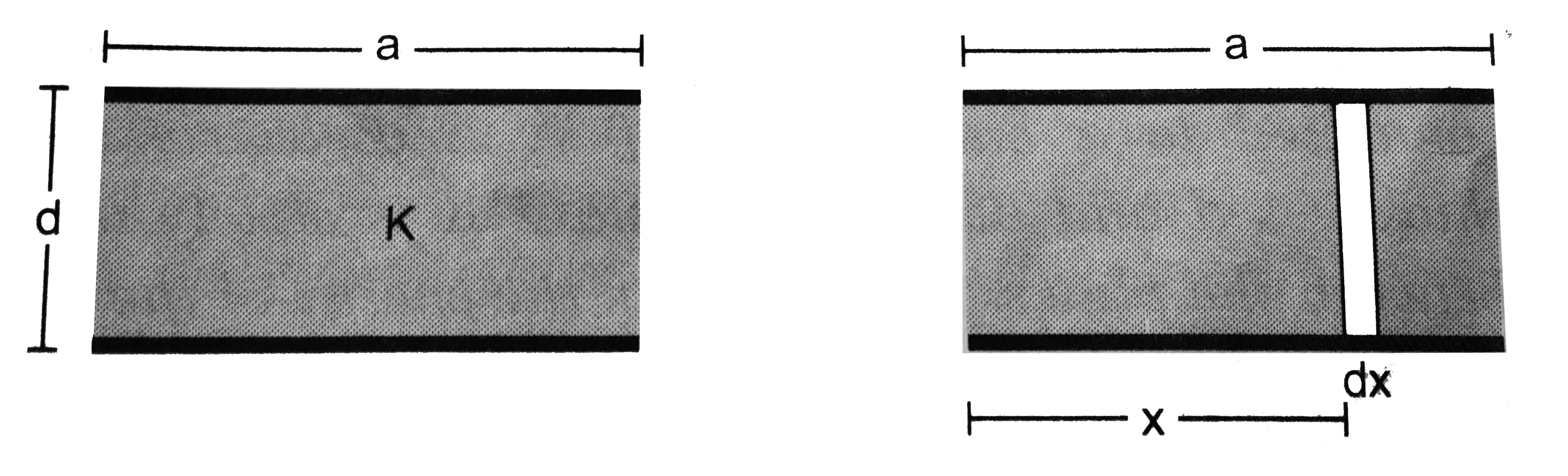
Figure shown a parallel-plate capacitor having square plates of edge a and plate-separation d. The gap between the plates is filled with a dielectric of dielectric constant K which varies parallel to an edge as K=K0+αx, where K and α are constants and x is the distance from the left end. Calculate the capacitance.
View SolutionA paralled plate capacitor is made of two square plates of side 'a' , separated by a distance d (dltlta). The lower triangular portion is filled with a dielecttic of dielectric constant K, as shown in the figure. Capacitance of this Capacitor is :
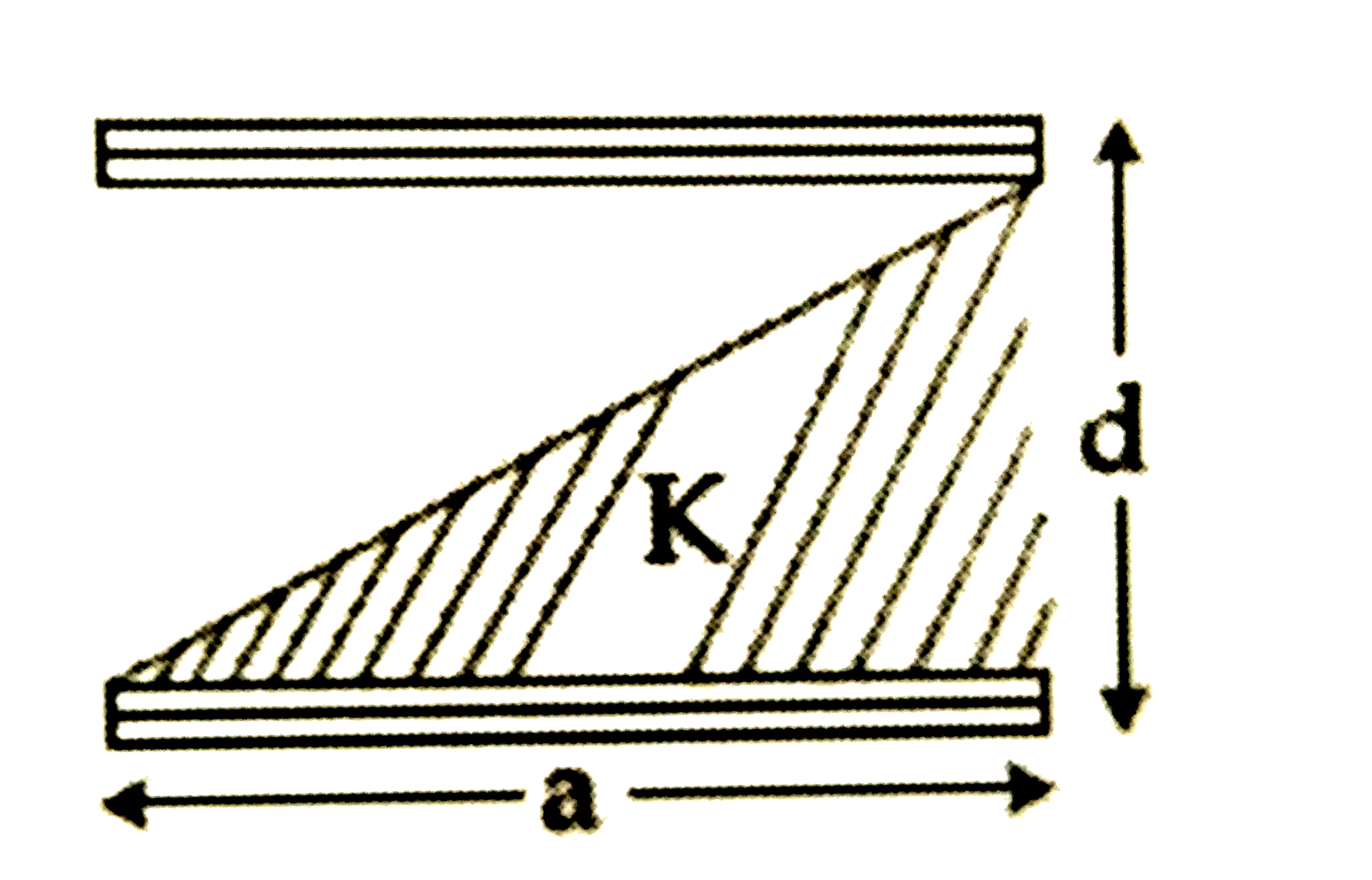
View SolutionWhen a parallel plate capacitor is filled with wax after separation between plates is doubled, its capacitance becomes twice. What is the dielectric constant of wax?
View SolutionA parallel plate capacitor with air between the plates has a capacitance C. If the distance between the plates is doubled and the space between the plates is filled with a dielectric of dielectric constant 6, then the capacitance will become.
View SolutionA capacitor with air as the dielectric is charged to a potential of 100 volts. If the space between the plates is now filled with a dielectric of dielectric constant 10, the potential difference between the plates will be
View Solution