When a parallel plate capacitor is filled with wax after separation between plates is doubled, its capacitance becomes twice. What is the dielectric constant of wax?
1
2
3
4
The correct Answer is:D
To solve the problem, we need to find the dielectric constant (k) of wax when a parallel plate capacitor is filled with wax after the separation between the plates is doubled, and its capacitance becomes twice.
1. Understand the Capacitance Formula:
The capacitance (C) of a parallel plate capacitor without a dielectric is given by the formula:
C=Aϵ0d
where:
- A = area of the plates
- ϵ0 = permittivity of free space
- d = separation between the plates
2. Capacitance with Dielectric:
When a dielectric material is inserted between the plates, the capacitance becomes:
C′=kAϵ0d
where k is the dielectric constant of the material.
3. Initial Conditions:
Let the initial capacitance be C:
C=Aϵ0d
4. New Conditions:
According to the problem, the separation between the plates is doubled, so the new separation d′=2d. The capacitance with the dielectric (wax) becomes:
C′=kAϵ02d
5. Capacitance Becomes Twice:
It is given that the new capacitance C′ is twice the original capacitance C:
C′=2C
6. Substituting Values:
Substitute the expressions for C and C′:
kAϵ02d=2(Aϵ0d)
7. Simplifying the Equation:
Cancel Aϵ0 from both sides:
k2=2
8. Solving for k:
Multiply both sides by 2 to isolate k:
k=4
Conclusion:
The dielectric constant of wax is k=4.
The capacitance (C) of a parallel plate capacitor without a dielectric is given by the formula:
C=Aϵ0d
where:
- A = area of the plates
- ϵ0 = permittivity of free space
- d = separation between the plates
When a dielectric material is inserted between the plates, the capacitance becomes:
C′=kAϵ0d
where k is the dielectric constant of the material.
Let the initial capacitance be C:
C=Aϵ0d
According to the problem, the separation between the plates is doubled, so the new separation d′=2d. The capacitance with the dielectric (wax) becomes:
C′=kAϵ02d
It is given that the new capacitance C′ is twice the original capacitance C:
C′=2C
Substitute the expressions for C and C′:
kAϵ02d=2(Aϵ0d)
Cancel Aϵ0 from both sides:
k2=2
Multiply both sides by 2 to isolate k:
k=4
The dielectric constant of wax is k=4.
Similar Questions
There is an air filled 1pF parallel plate capacitor. When the plate separation is doubled and the space is filled with wax, the capacitance increases to 2pF. The dielectric constant of wax is
View SolutionA parallel plate capacitor is filled with dielectrics as shown in Fig. What is its capacitance ?

View Solution
Knowledge Check
The capacitance of a parallel plate capacitor is 2.5μF when it is half filled with a dielectric as shown in the figure, Its capacitance becomes 5μF, the dielectric constant of the dielectric is
 A7.5B3C0.33D4
A7.5B3C0.33D4A parallel plate capacitor is filled with dielectrics. What is capacitance?
 A2K1K2K1+K2BK1K2K1+K2C2ε0Ad(K1K2K1+K2)D2ε0Ad(2K1+K22K1K2)
A2K1K2K1+K2BK1K2K1+K2C2ε0Ad(K1K2K1+K2)D2ε0Ad(2K1+K22K1K2)The capacitance of an air filled parallel plate capacitor is 10μF. The separation between the plates is doubled and the space between the plates is then filled with wax giving the capacitance a new value of 40×10−12 farads. The dielectirc consatnt of wax is
A12.0B10.0C8.0D4.2
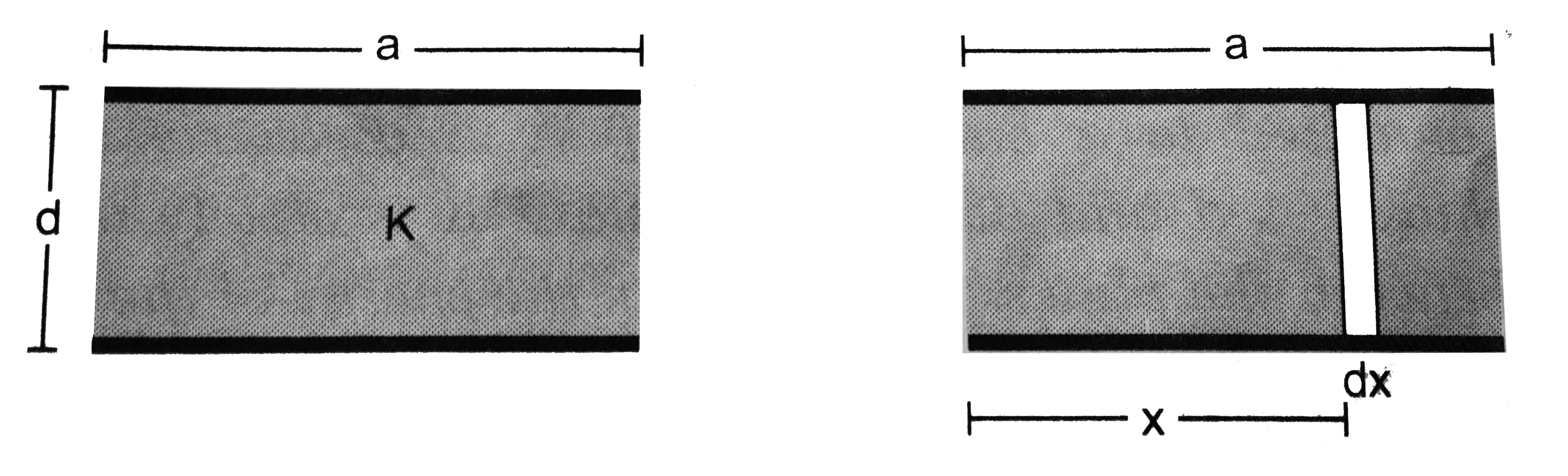
Figure shown a parallel-plate capacitor having square plates of edge a and plate-separation d. The gap between the plates is filled with a dielectric of dielectric constant K which varies parallel to an edge as K=K0+αx, where K and α are constants and x is the distance from the left end. Calculate the capacitance.
View SolutionThe capacitance of a parallel plate capacitor with plate area A and separation d is C. The space between the plates in filled with two wedges of dielectric constants K1 and K2 respectively. Find the capacitance of resulting capacitor.

View SolutionA parallel plate air capacitor has a capacity of 2 pF. If the separation between its plates is doubled and a mica sheet is introduced between its plates, its capacity becomes 6 pF. What is the dielectric constant of mica?
View SolutionA parallel plate capacitor has plate area A and plate separation d. The space between the plates is filled up to a thickness x<d with a dielectric constant K. Calculate the capacitance of the system.

View SolutionA dielectric of thickness 5cm and dielectric constant 10 is introduced between the plates of a parallel plate capacitor having plate area 500 sq. cm and separation between the plates 10cm. The capacitance of the capacitor with dielectric slab is
(ε0=8.8×10−12C2/N−m2)
View Solution