A dielectric of thickness 5cm and dielectric constant 10 is introduced between the plates of a parallel plate capacitor having plate area 500 sq. cm and separation between the plates 10cm. The capacitance of the capacitor with dielectric slab is
(ε0=8.8×10−12C2/N−m2)
4.4pF
6.2pF
8pF
10pF
The correct Answer is:C
To find the capacitance of a parallel plate capacitor with a dielectric slab inserted, we can follow these steps:
Step 1: Identify the Parameters
- Dielectric thickness td=5cm=0.05m
- Dielectric constant k=10
- Plate area A=500cm2=500×10−4m2=5×10−2m2
- Total separation between plates d=10cm=0.1m
Step 2: Calculate the Capacitance of the Dielectric Section (C1)
The capacitance of the section with the dielectric is given by:
C1=kϵ0Atd
Where ϵ0=8.85×10−12F/m.
Substituting the values:
C1=10×8.85×10−12×5×10−20.05
Calculating C1:
C1=10×8.85×10−12×5×10−20.05=8.85×10−12×10−1=8.85×10−12×10=88.5×10−12=88.5pF
Step 3: Calculate the Capacitance of the Air Section (C2)
The remaining distance between the plates that is filled with air is:
ta=d−td=0.1−0.05=0.05m
The capacitance of the air section is given by:
C2=ϵ0Ata
Substituting the values:
C2=8.85×10−12×5×10−20.05
Calculating C2:
C2=8.85×10−12×5×10−20.05=8.85×10−12×10−1=8.85×10−12×10=8.85×10−12×10=8.85pF
Step 4: Calculate the Equivalent Capacitance (Ceq)
Since C1 and C2 are in series, the equivalent capacitance Ceq can be calculated using:
1Ceq=1C1+1C2
Substituting the values:
1Ceq=188.5×10−12+18.85×10−12
Calculating:
1Ceq=188.5+18.85≈0.0113+0.113=0.1243
Thus,
Ceq=10.1243≈8.04pF
Final Answer
The equivalent capacitance of the capacitor with the dielectric slab is approximately:
Ceq≈8pF
- Dielectric thickness td=5cm=0.05m
- Dielectric constant k=10
- Plate area A=500cm2=500×10−4m2=5×10−2m2
- Total separation between plates d=10cm=0.1m
The capacitance of the section with the dielectric is given by:
C1=kϵ0Atd
Where ϵ0=8.85×10−12F/m.
C1=10×8.85×10−12×5×10−20.05
Calculating C1:
C1=10×8.85×10−12×5×10−20.05=8.85×10−12×10−1=8.85×10−12×10=88.5×10−12=88.5pF
The remaining distance between the plates that is filled with air is:
ta=d−td=0.1−0.05=0.05m
The capacitance of the air section is given by:
C2=ϵ0Ata
Substituting the values:
C2=8.85×10−12×5×10−20.05
Calculating C2:
C2=8.85×10−12×5×10−20.05=8.85×10−12×10−1=8.85×10−12×10=8.85×10−12×10=8.85pF
Since C1 and C2 are in series, the equivalent capacitance Ceq can be calculated using:
1Ceq=1C1+1C2
Substituting the values:
1Ceq=188.5×10−12+18.85×10−12
Calculating:
1Ceq=188.5+18.85≈0.0113+0.113=0.1243
Thus,
Ceq=10.1243≈8.04pF
The equivalent capacitance of the capacitor with the dielectric slab is approximately:
Ceq≈8pF
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCEBOOK - NARAYNACHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - Exercise-2(C.W)
53 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCEBOOK - NARAYNACHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - EXERCISE -2 (H.W)
50 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCEBOOK - NARAYNACHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - Exercise -1 (C.W)
44 Videos
- ELECTROMAGNETIC WAVES BOOK - NARAYNACHAPTER - ELECTROMAGNETIC WAVES EXERCISE - EXERCISE -4
15 Videos
- ELECTROSTATICS AND GAUSS LAWBOOK - NARAYNACHAPTER - ELECTROSTATICS AND GAUSS LAWEXERCISE - Intergers type question
11 Videos
Similar Questions
An ebomiote rod (K = 3), 6 mm thick is introduced between the plates of a parallel plate capacitor of plate area 4×10−2m2 and plate separation 0.01m. Find the capacitance.
View SolutionA dielectric slab of thickness 1.0 cm and dielectric constant 5 is placed between the plates of a parallel plate capacitor of plate area 0.01m2 and separation 2.0 cm. Calculate the change in capacity on introduction of dielectric. What would be on the change, if the dielectric slab were conducting?
View Solution
Knowledge Check
If a dielectric slab of thickness 5 mm and dielectric constant K=6 is introduced between the plates of a parallel plate air capacitor, with plate separation of 8 mm, then its capacitance is
A1. decreasedB2. unaffectedC3. almost halvedD4. almost doubledA dielectric slab is placed between the plates of a parallel plate capacitor. Its capacitance
Abecomes zeroBremains the sameCdecreasesDincreasesArea of a parallel plate capacitor of capacitance 2F and separation between the plates 0.5cm will be
A1.13×109m2B1.13×106m2C108m2D1.13m2
What is the area of the plates of a 2 farad parallel plate air capacitor, given that the separation between the plates is 0.5 cm?
View SolutionA dielectric slab of thickness 't' is kept between the plates of a parallel plate capacitor separated by a distance 'd' (t<d) . Derive the expression for the capacity of the capacitor .
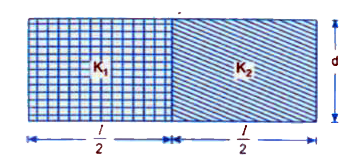
View SolutionTwo dielectric slabs of dielectric constants K1andK2 are filled in between the two plates, each of area A, of the parallel plate capacitor as shown in the figure. Find the net capacitance of the capacitor.
View SolutionA metel plate of thickness 2 cm is introduced between the plates of a parallel plate air capacitor having a plate separation of 6 cm. what is the ratio of the capacities of the capacitor before and after introducing the metal plate?
View SolutionWhen a dielectric slab of thickness 4cm is introduced between the plates of parallel plate condenser, it is found the distance between the plates has to be increased by 3cm to restore to capacity to original value. The dielectric constant of the slab is.
View Solution
