A parallel plate capacitor is to be constructed which can store q=10μC charge at V=1000 volt. The minimum plate area of the capacitor is required to be A1 when space between the plates has air. If a dielectric of constant K=3 is used between the plates, the minimum plate area required to make such a capacitor is A2. The breakdown field for the dielectric is 8 times that of air. Find A1A2
The correct Answer is:24
To solve the problem, we need to find the ratio of the minimum plate areas A1 and A2 for a parallel plate capacitor with and without a dielectric.
1. Understanding the Problem:
- We have a capacitor that stores a charge q=10μC=10×10−6C at a voltage V=1000V.
- We need to find the minimum plate area A1 when the space between the plates has air and A2 when a dielectric with constant K=3 is used.
2. Capacitance Formula:
- The capacitance C of a parallel plate capacitor is given by:
C=εAd
where ε is the permittivity of the material between the plates, A is the area of the plates, and d is the separation between the plates.
3. Charge and Capacitance Relationship:
- The relationship between charge, capacitance, and voltage is given by:
q=C⋅V
- Rearranging gives:
C=qV
4. Finding A1 (Air):
- For air, the permittivity ε0 is used:
C1=ε0A1d
- Thus:
A1=C1⋅dε0
- Substituting for C1:
A1=q⋅dε0V
5. Finding A2 (With Dielectric):
- When a dielectric is present, the capacitance becomes:
C2=Kε0A2d
- Thus:
A2=C2⋅dKε0
- Substituting for C2:
A2=q⋅dKε0V
6. Finding the Ratio A1A2:
- Now we can find the ratio:
A1A2=q⋅dε0Vq⋅dKε0V=11K=K
- Given K=3:
A1A2=3
7. Considering Breakdown Fields:
- The problem states that the breakdown field for the dielectric is 8 times that of air. This means:
EdielectricEair=8
- The electric field E is related to voltage and distance:
E=Vd
- Therefore, we can incorporate this into our calculations, but since we are only interested in the ratio of areas, we can conclude:
A1A2=K⋅EdielectricEair=3⋅8=24
Final Answer:
A1A2=24
- We have a capacitor that stores a charge q=10μC=10×10−6C at a voltage V=1000V.
- We need to find the minimum plate area A1 when the space between the plates has air and A2 when a dielectric with constant K=3 is used.
- The capacitance C of a parallel plate capacitor is given by:
C=εAd
where ε is the permittivity of the material between the plates, A is the area of the plates, and d is the separation between the plates.
- The relationship between charge, capacitance, and voltage is given by:
q=C⋅V
- Rearranging gives:
C=qV
- For air, the permittivity ε0 is used:
C1=ε0A1d
- Thus:
A1=C1⋅dε0
- Substituting for C1:
A1=q⋅dε0V
- When a dielectric is present, the capacitance becomes:
C2=Kε0A2d
- Thus:
A2=C2⋅dKε0
- Substituting for C2:
A2=q⋅dKε0V
- Now we can find the ratio:
A1A2=q⋅dε0Vq⋅dKε0V=11K=K
- Given K=3:
A1A2=3
- The problem states that the breakdown field for the dielectric is 8 times that of air. This means:
EdielectricEair=8
- The electric field E is related to voltage and distance:
E=Vd
- Therefore, we can incorporate this into our calculations, but since we are only interested in the ratio of areas, we can conclude:
A1A2=K⋅EdielectricEair=3⋅8=24
A1A2=24
Topper's Solved these Questions
Similar Questions
A resistance R and a capacitor having capaci- tance C are connected to an alternating source having emf v=V0sin(ωt).It is given that ω=1√3RC
(a) Plot the variation of power supplied by the source as a function of time. Mark the maximum and minimum values of power in the graph.
(b) How does the plot change if capacitor is removed and only R remains connected to the source?
(c) Plot the graph when only C remains connected to source and R is removed.
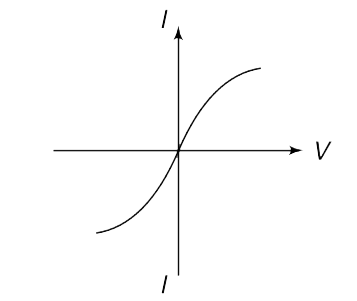
View SolutionThe current-voltage graph for a resistor is as shown in the figure. Is it right to say that resistance decreases as the current through it increases ?
View SolutionAn air -filled parallel-plate capacitor is to be constructed which can store12μC of charge when operated at 1200V.What can be the minimum plate area of the capacitor?The dielectric strength air is 3×106Vm−1.
View SolutionA parallel plate capacitor has an electric field of 10^(5)V//m between the plates. If the charge on the capacitor plate is 1muC , then force on each capacitor plate is-
View Solutionएक समांतर प्लेट तथा वायु संधारित्र की प्लेटों का क्षेत्रफल 100सेमी2 व इनके बीच की दुरी 5 मिमी है। प्लेटों के बीच बैटरी द्वारा 300 वोल्ट विभवांतर स्थापित किया गया है-
(A) प्लेटों के बीच वैघृत क्षेत्र, प्लेटों पर आवेश घनत्व तथा संधारित्र में संचित ऊर्जा का मान ज्ञात कीजिये।
(B) यदि बैटरी संधारित्र की प्लेटों के बीच जुडी रखते हुए, प्लेटों के बीच का स्थान एबोनाइट से भर दिया जाये तो नयी स्थिति में प्लेटों के बीच वैघृत क्षेत्र, प्लेटों पर आवेश घनत्व तथा संधारित्र में संचित ऊर्जा का मान क्या होगा?
View SolutionA parallel plate capacitor has an electric field of 10^(5)V//m between the plates. If the charge on the capacitor plate is 1muC , the force on each capacitor plate is
View SolutionA.P.D. of V volts is applied across the plates of a parallel plate capacitor having plate area A. if Q is the charge on its plates and K is the dielectric constant of the medium, between the plates, then the plate separation is given by
View Solutionएक समांतर पट्टिका वायु-संधारित्र बनाना है जिसे 1200 V के विभवांतर के बीच जोड़ने पर 12μC का आवेश संचित किया जा सके। संधारित्र की प्लेटो का न्यूनतम क्षेत्रफल ज्ञात करे, यदि वायु का परावैघुत सामर्थ्य 3×106Vm−1 हो।
View SolutionA parallel plate capacitor having plate area A and separation between the plates d is charged to potential V .The energy stored in capacitor is ....???
View SolutionFind an expression for the capacitance of a parallel plate capacitor when a dielectric slab of dielectric constant K and thickness t=(d)/(2) but the same area on the plates is inserted between the the capacitors plate. (d=separation between the plates)
View Solutionएक समान्तर प्लेट संधारित्र की प्लेटों के बीच दूरी d तथा प्लेटों के क्षेत्रफल A के बीच क्षेत्र में एकसमान विद्युत क्षेत्र E है। इस आवेश संधारित्र की ऊर्जा है -
View Solutionएक समानांतर पट्टिकये संधारित्र की प्लेटो के बीच की दूरी d और प्लेटो का अनुप्रस्थ परिच्छेदित क्षेत्रफल A है। इसे आवेशित कर प्लेटो के बीच अचर विधुत क्षेत्र E बनाना है। इसे आवेशित करने के लिए आवश्यक ऊर्जा होगी
View Solutionचित्र में प्रदर्शित संधारित्र की प्लेटो का क्षेत्रफल A1 तथा A2(A1<A2) है तथा प्लेटो के बीच की दूरी d है। संधारित्र की धारित्र ज्ञात कीजिए।
View SolutionAn air -filled parallel-plate capacitor is to be constructed which can store12μC of charge when operated at 1200V.What can be the minimum plate area of the capacitor?The dielectric strength air is 3×106Vm−1.
View SolutionA parallel plate capacitor has an electric field of 10^(5)V//m between the plates. If the charge on the capacitor plate is 1muC , then force on each capacitor plate is-
View Solution
