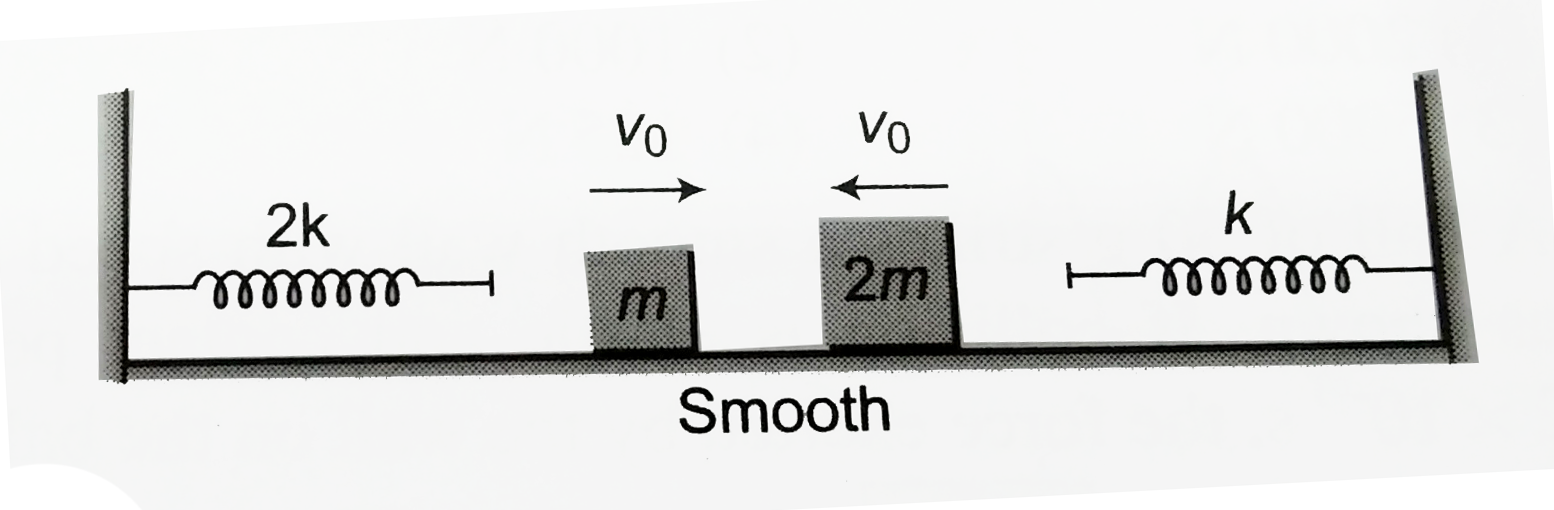
If blocks collide elastically head - on , the ratio of maximum compression of the left spring and the right spring will be
3:5
2:3
5:4
5:2
The correct Answer is:D
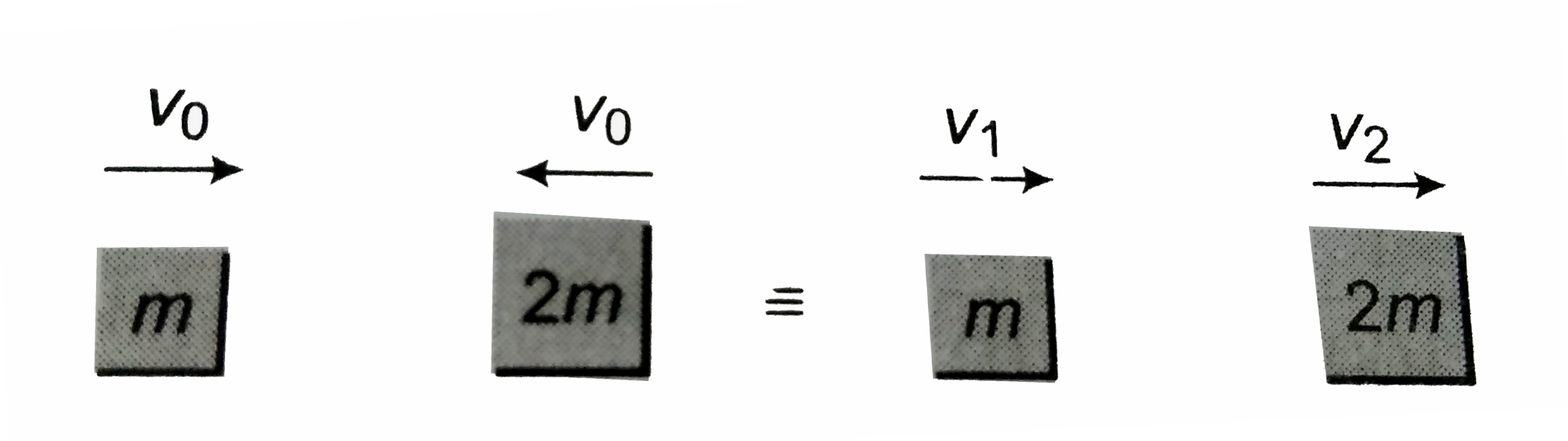
mv0−2mv0=mv1+2mv2 (i)
v1+v0=v2+(−v0) (ii)
Solving v1=−5v03,v2=v03
Let the maximum compression be x1
12m(5v03)2=122kx21⇒x21=25mv2018k (i)
Let the maximum compression be x2
12×2m(v03)2=12kx22⇒x22=2mv209k (ii)
x21x22=254⇒x1x2=52
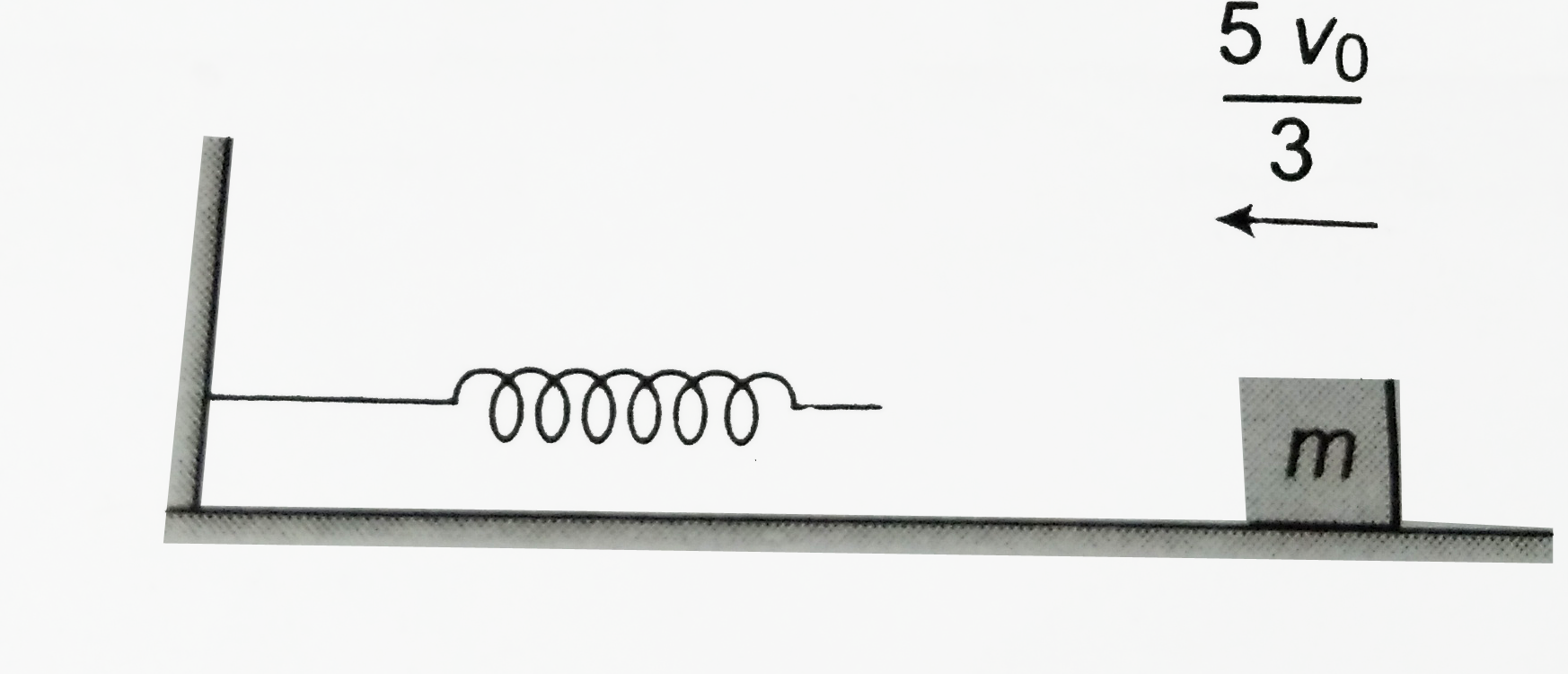
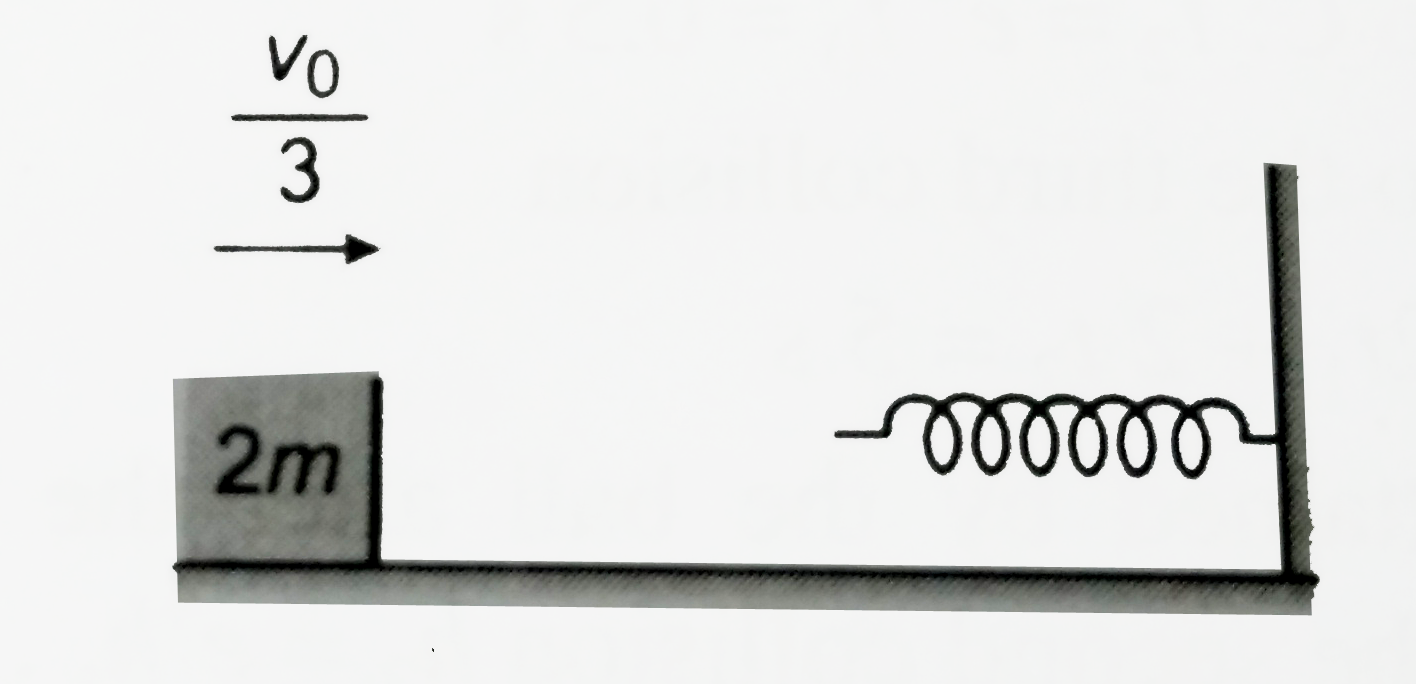
Topper's Solved these Questions
Similar Questions
Knowledge Check
Find maximum compression is the spring from the given figure.
 A√25mB16√25mC3√25mD6√25m
A√25mB16√25mC3√25mD6√25mThe ball sticks to block C, then block Ccollides elastically , head - on with A. If the maximum compression is x0, the spring constant k is
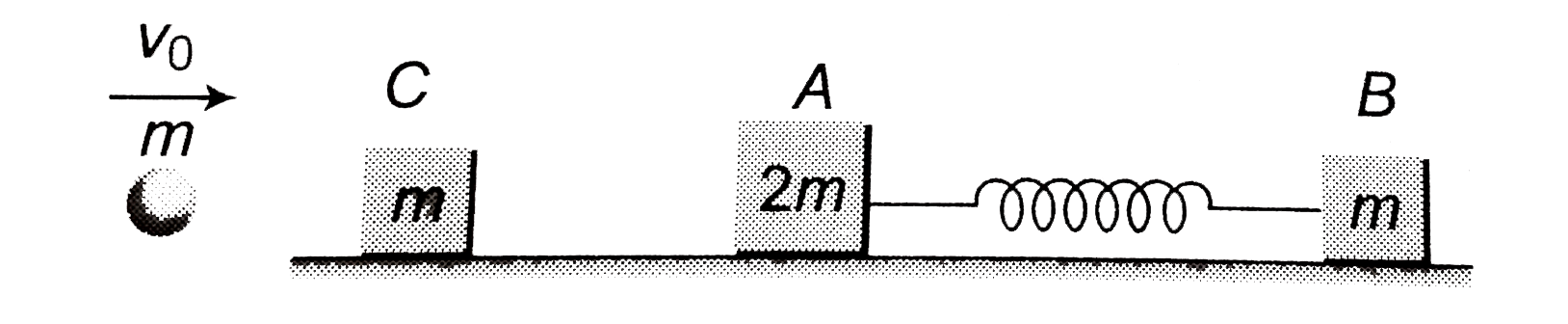 A23mv20x20Bmv202x20Cmv203x20Dmv206x20
A23mv20x20Bmv202x20Cmv203x20Dmv206x20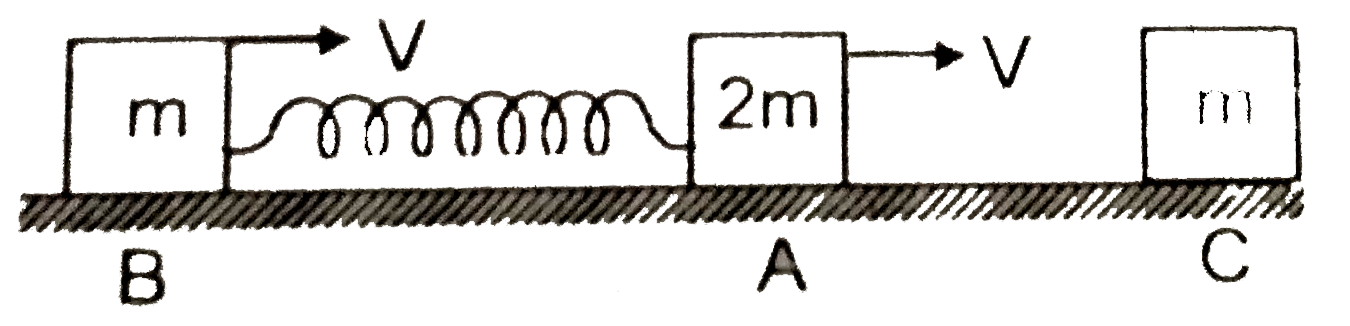
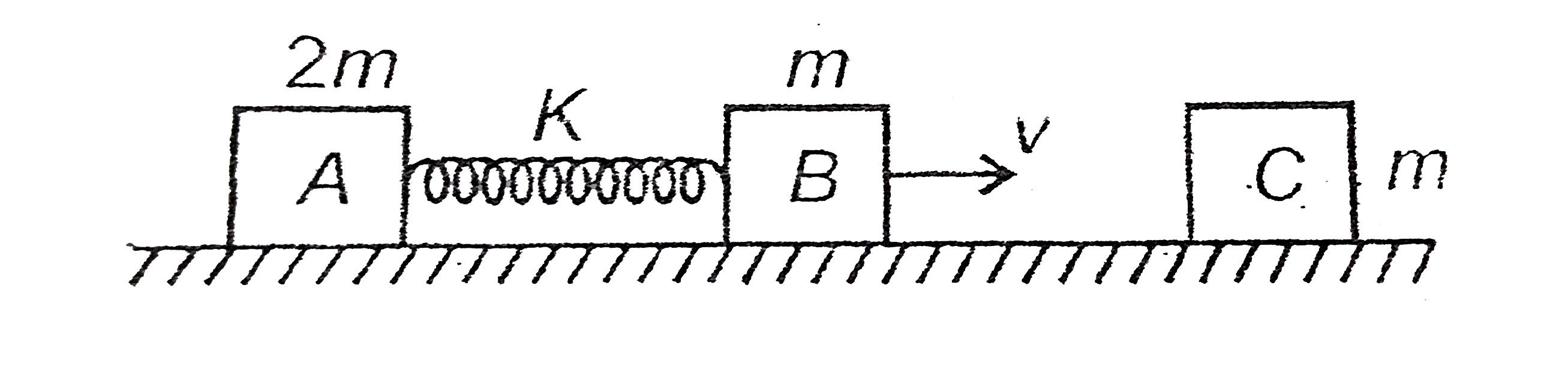
Two blocks A and B of mass 2 m and m respectively are connected to a massless spring of spring constant K. if A and B moving on the horizontal frictionless surface with velocity v to right. If A collides with C of mass m elastically and head on, then the maximum compressions of the spring will beA√32mkVB√27m8KVC√9m8KVD√8m27KV
A block of mass 180g is placed on a spring (spring constant k=120N/m) fixed from below. A ball of mass 20g is dropped from height 20m and the collision is completely inelastic. Find the maximum compression of the spring. Neglect the initial compression of the spring due to the block.
View Solution
In the figure, the spring is initially compressed by 3 cm and released. The block collides withth wall and rebounds to compress the spring again. The maximum compression in the srping after collision (coefficient of restitution is 0.7) is ........cm (Take √5=2.4,k=104Nm−1)
View SolutionTwo blocks A and B of mass 2m and m respectively are connected to a massless spring of force constant K as shown in figure A and B are moving on the horizontal frcitionless surface with velocity v to right with underformed spring. If B collides with C elastically, then maximum compression of the spring will be
View SolutionA block of mass m attached with a spring and held by a person such that the spring is in natural length l0. Now the man releases the block, the ratio of maximum compression in the spring in the given situation , to that of the compression at equilibrium position.

View SolutionIn figure, a block A of mass 2kg is moving to the right with a speed 5m/s on a horizontal frictionless surface. Another block B of mass 3kg a massless spring of spring constant 222N/m attached to it, is moving to the left on the same surface and with a speed 2m/s . Let us take the direction to the right as the positive X− direction. At some instant, block A collides with the spring attached to block B. At some other instant , the spring has maximum compression and then, finally, blocks move with their final velocities. Assuming that (i) the spring force is conservative and so there is no conversion of kinetic energy to internal energy and (ii), no sound is made when block A hits the spring, answer the following questions.
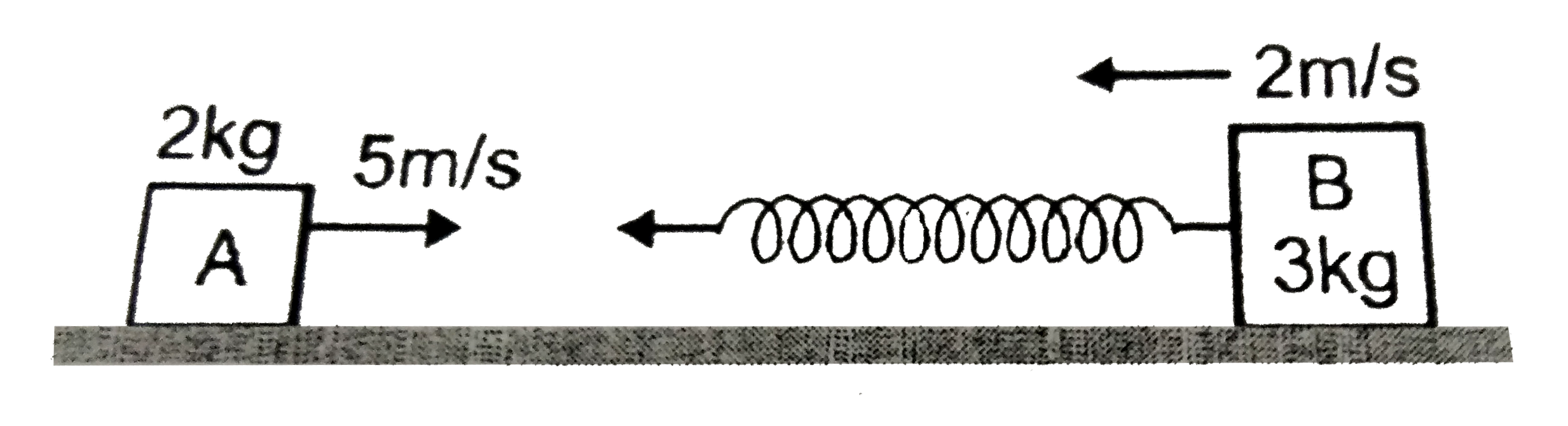
Maximum compression of the spring in the collision will be nearly
View Solution