In the previous problem , if the collision is inelastic and the coefficient of restitution is e=1/2 , the momentum imparted to the way by the wall is
A
0.25kgm/s
B
0.50kgm/s
C
0.75kgm/s
D
1kgm/s
Video Solution
Text Solution
The correct Answer is:C
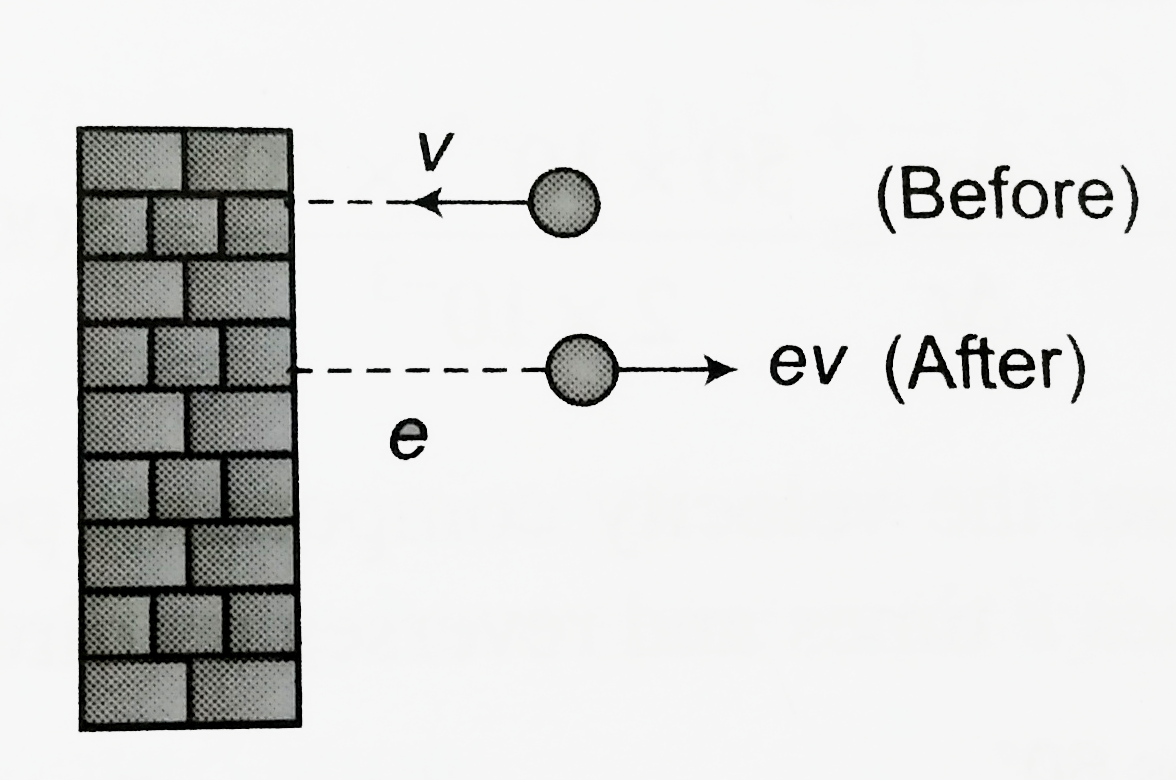
The speed of the ball becomes ev after the collision as shown.
Change in momentum of ball =mev−(−mv)=mv(1+e)
Since in collision , the momentum is conserved ,
Momentum imparted to wall =mv(1+e) in opposite direction.
mv(1+e)=50×10−3×10(1+12)=0.75kgm/s
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
In the previous problem , if the collision is inelastic and e=1/2
AAfter collision , the ball moves with 5√13m/s at angle tan−1(2√3) with horizontalBForce by wall on the ball is 375N to rightCChange in momentum of ball is 0.75kgm/sDAll options are correctIn the previous problem , the maximum loss in K.E. will be
A12mv20B13mv20C23mv20D34mv20A ball falls vertically onto a floor with momentum p and then bounces repeatedly. If coefficient of restitution is e, then the total momentum imparted by the ball to the floor is
Ap(1+e)Bp1−eCp(1+1e)Dp(1+e1−e)
For a superelastic collision, the coefficient of restitution is---------- unity.
View SolutionIn the previous problem , time taken by the ball up to the third collision
View SolutionA block of mass m moving with speed v0 strikes another particle of mass 2m at rest. If collision is head - on and the coefficient of restitution e=1/2 , then the loss in kinetic energy will be
View SolutionA ball falls vertically onto a floor with momentum p and then bounces repeatedly. If coefficient of restitution is e, then the total momentum imparted by the ball to the floor is
View SolutionThe coefficient of restitution e for a perfectly elastic collision is
View Solution