Find the potential energy of the gravitational interaction
(a) of two mass points of masses $m_{1}$ and $m_{2}$ located at a distance r from each other,
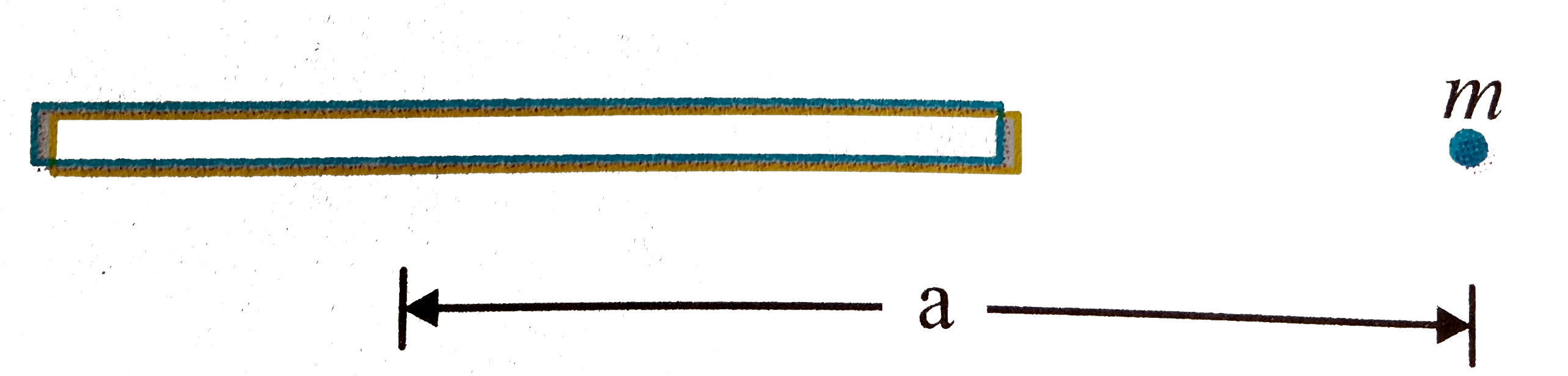
(b) of a mass point of mass m and a thin uniform rod of mass M and length l, if they are located along a straight line at a distance a from each other, also find the force of their interaction.

Video Solution

Text Solution

Verified by Experts

(a) The gravitational potential due to $m_{1}$ at the point of location of $m_{2}$ :
$V_{2} = \infty \int r \to G \cdot d \to r = \infty \int r - \frac{γ m_{1}}{x^{2}} d x = - \frac{γ m_{1}}{r}$
So, $U_{21} = m_{2} V_{2} = - \frac{γ m_{1} m_{2}}{r}$
Similarly $U_{12} = - \frac{γ m_{1} m_{2}}{r}$
Hence $U_{12} = U_{21} = U = - \frac{γ m_{1} m_{2}}{r}$

(b) Choose the location of the point mass as the origin. Then the potential energy $d U$ of an element of mass $d M = \frac{M}{l} d x$ of the rod in the field of the point mass is
$d U = - γ m \frac{M}{l} d x \frac{1}{x}$
where x is the distance between the element and the point. (Note that the rod and the point mass are on a straight line.) If then a is the distance of the nearer end of the rod from the point mass.

$U = - γ \frac{m M}{l} a + l \int a \frac{d x}{x} = - γ m \frac{M}{l} 1 n (1 + \frac{l}{a})$
The force of interaction is
$F = - \frac{δ U}{δ a}$
$= γ \frac{m M}{l} \times \frac{1}{1 + \frac{l}{a}} (- \frac{l}{a^{2}}) = - \frac{γ m M}{a (a + l)}$
Minus sign means attraction.

Updated on:21/07/2023

Choose the most appropriate option.
The potential energy of gravitational interaction of a point mass m and a thin uniform rod of mass M and length L, if they are located along a straight line at a distance a from each other, is
A $U = \frac{G M m}{a} ln (\frac{a + L}{α})$
B $U = G M m (\frac{1}{a} - \frac{1}{a + L})$
C $U = - \frac{G M m}{a}$
D $U = - \frac{G M m}{L} ln (\frac{a + L}{a})$
Find the potential energy of the gravitational interaction of a point mass $m$ and a rod of mass $m$ and length $l$ , if they are along a straight line. Point mass is at a distance of a from the end of the rod.

Find the potential energy of the gravitational
A $U = \frac{- G m^{2}}{l} ln (\frac{2 a + l}{2 a - l})$
B $U = \frac{- G m^{2}}{l^{2}} ln (\frac{2 a + l}{2 a - l})$
C $U = \frac{- G m^{2}}{l^{2}} ln (\frac{2 a - l}{2 a + l})$
D $U = \frac{- G m^{2}}{l^{2}} {ln (\frac{2 a - l}{2 a + l})}^{2}$
Two bodies of masses m and 2m are kept at distance r apart from each other. Then the value of G varies as
A $r^{2}$
B $r^{4}$
C $r^{- 2}$
D $r^{t h}$