A non-conducting disc of radius a and uniform positive surface charge density σ is placed on the ground, with its axis vertical. A particle of mass m and positive charge q is dropped, along the axis of the disc, from a height H with zero initial velocity. The particle has q/m=4∈0g/σ
(a) Find the value of H if the particle just reaches the disc.
(b) Sketch the potential energy of the particle as a function of its height and find its equilibrium position.
The correct Answer is:(a) H=4a3, (b) U=mg[2√h2+a2−h]
equilibrium at h=a√3.
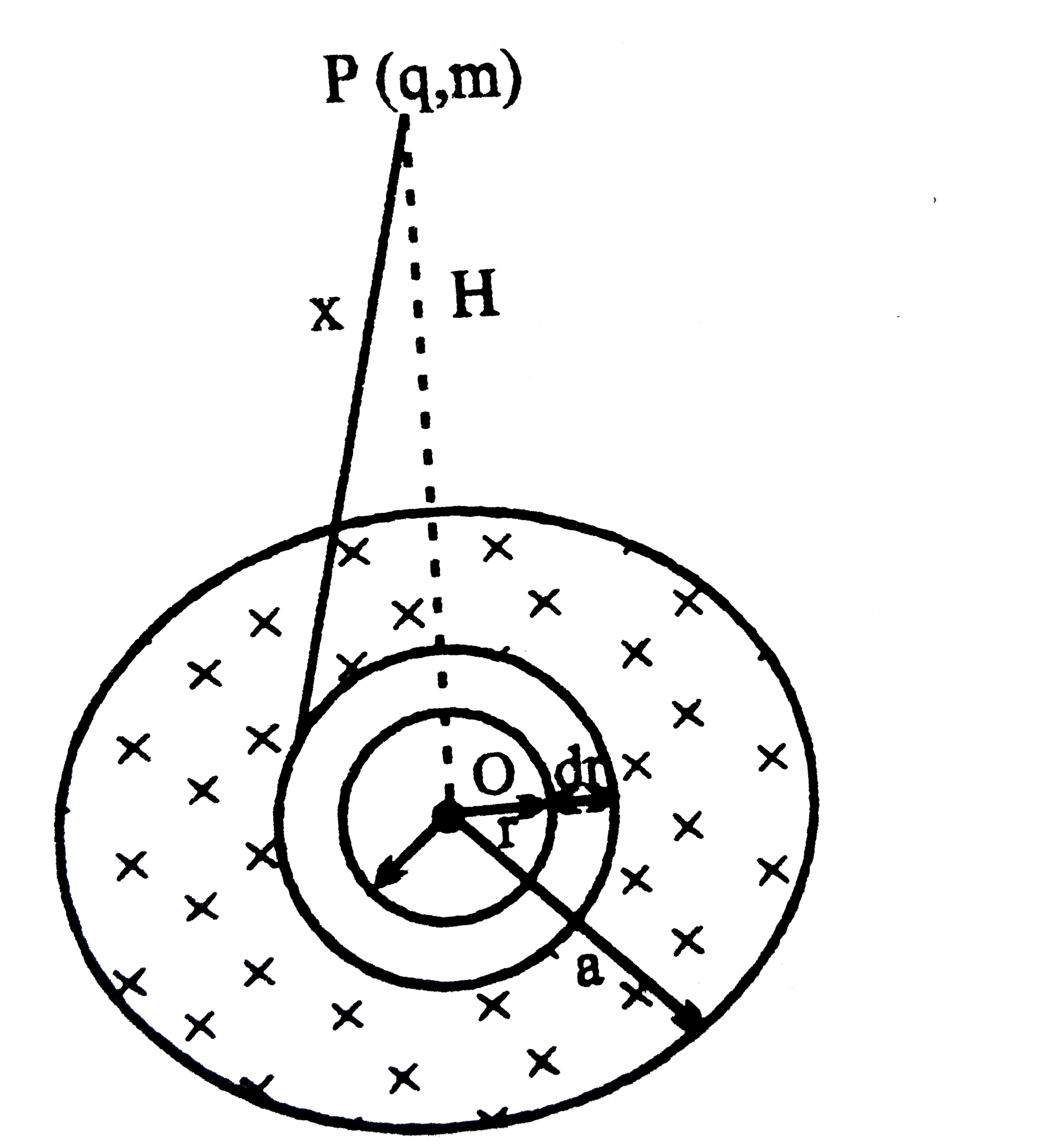
Potential at a height H on the axis of the disce V the charge dp conttained in the ring shown figure
dp=σ(2πrdr)
Potentiall at P due to this ring
dV=14πɛ0dq√H2+r2
⇒dV=14π∈0(2πrdr)σ√H2+r2=σ2π0rde√H2+r2
∴ Potential due to the complete disc Vp=∫r=ar=0dV
Vp=σ2∈0imtr=ar=0rdr√H2=r2
 .
.
Vp=σ2∈0[√H2+a2)−H]
Poyenyial at center will be V0=σa2ɛ0asH=0
(a) Particel is relased from P and it just reaches point O. therefore , form conservation of mechanical energy
Decrease in gravitational potential energy = Increase in eleratostatic potential energy
putH=q[V0−Va]
gH=(qm)[σ2∈0a−σ2∈0√a2+H2−H]....(1)
putqm=4∈0gsigam in equation (1)
H=(43)a
b Potential energy of the particel at height H= Electrostyatic potential energy + gravitational potential energy .
U=qV+mgH
Here V= Potential at height H
U=σ2∈0[√a2+H2−H]mgH ..(2)
At equilibrium potition : F=−dUdh=0
differntiating 2 w.r.t H
or mg+σq2∈0[(12)(2H)1√a2+H20−1=0
[∴σq2∈=2mg]
by sovloing H=a√3
From equation 2 we can write
U−H equation as
U=2mg(√a2+H2−H)
U=2mgaatH=0 and
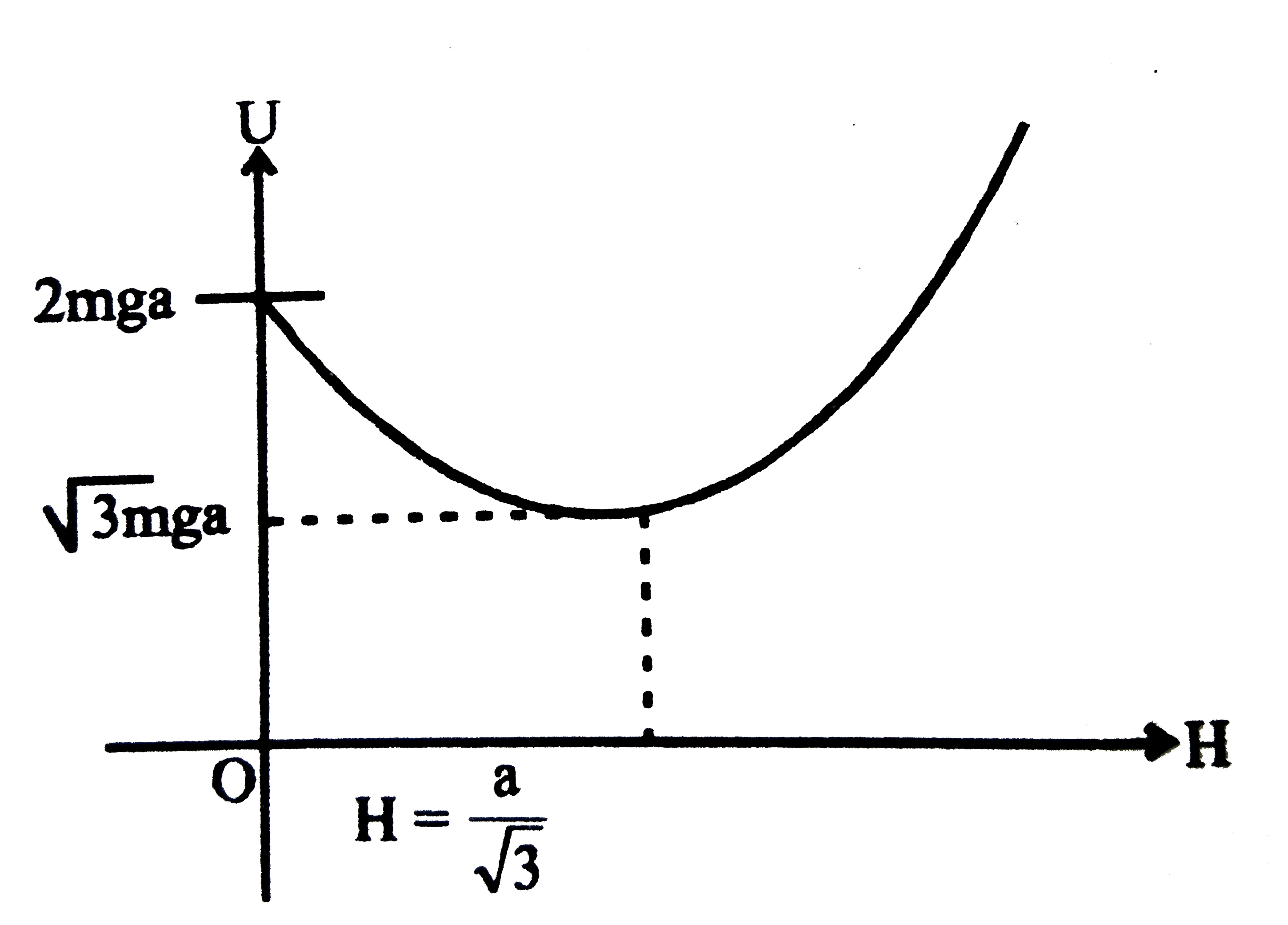
U=Umin=√3 mga at H=a√3
Therefor U−H graph will be as shown
Note that at H=a√3 is stable equilibrium postion .
Similar Questions
A nonconducting disk of radius a and uniform positive surface charge density σ is placed on the ground, with its axis vertical. A particle of mass m and positive charge q is dropped, along the axis of the disk, from a height H with zero initial velocity. The particle has q/m=4ε0g/σ.
(i) Find the value of H if the particle just reaches the disk.
(ii) Sketch the potential energy of the particle as a function of its height and find its equilibrium position.
View SolutionA dielectric disc of radius R and uniform positive surface charge density σ is placed on the grup with its axis vertical. A particle of mass m and positive charge q is dropped, along the axis of the disc from a height H with dropped, along the axis of the disc from a height H with zero intial velocity. The charge -mass ratio of the particle is qm=4εon0gσ.
(i). Find the value of H if the particle just reaches the disc.
(ii). Sketch the potential energy of the particle as a function of its height and find its height in equilibrium position.
View Solution
Knowledge Check
A non-conducting semi circular disc (as shown in figure) has a uniform surface charge density σ. The ratio of electric field to electric potential at the centre of the disc will be
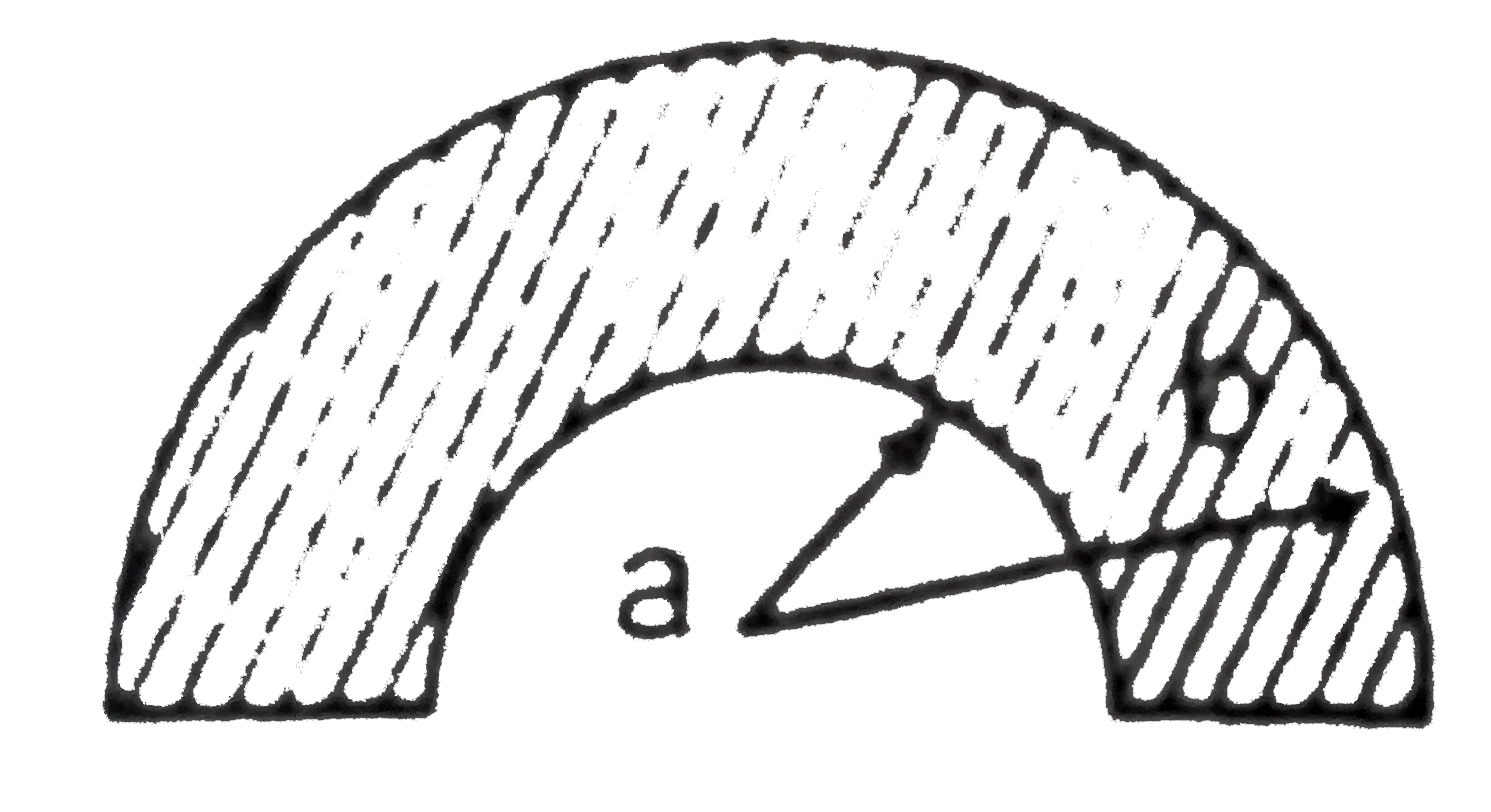 A1πlnb/a(b−a)B2πC1πln(b/a)2(b−a)Dπ(b−a)2ln(b/a)
A1πlnb/a(b−a)B2πC1πln(b/a)2(b−a)Dπ(b−a)2ln(b/a)
A uniformly charged disc of radius R having surface charge density σ is placed in the xy plane with its center at the origin. Find the electric field intensity along the z-axis at a distance Z from origin :
View SolutionA non-conducting thin disc of radius R charged uniformly over one side with surface density s rotates about its axis with an angular velocity ω. Find
(a) the magnetic induction at the centre of the disc,
(b) the magnetic moment of the disc.
View SolutionA non-conducting thin disc of radius R charged uniformly over one side with surface density σ, rotates about its axis with an angular velocity ω. Find (a) the magnetic field induction at the centre of the disc (b) the magnetic moment of the disc.
View SolutionA circular ring of radius R with uniform positive charge density λ per unit length is located in the y-z plane with its centre at the origin O. A particle of mass m and positive charge q is projected from the point P (R√3,0,0) on the positive x-axis directly towards O, with an initial speed v. Find the smallest (non-zero) value of the speed v such that the particle does not return to P.
View SolutionA flat disc of radius R charged uniformly on its surface at a surface charge density σ. About its central axis of rotation it rotates at an angular speed ω. Find the magnetic moment of disc due to rotation of charges.
View SolutionPositive charge Q is distributed uniformly over a circular ring of radius R. A particle having a mass m and a negative charge q, is placed on its axis at a distance x from the centre. Find the force on the particle. Assuming x ltltR, find the time period of oscillation of the particle if it is released from there.
View SolutionA smooth chute is made in a dielectric sphere of radius R and uniform volume charge density. ρ. A charge particle of mass m and charge -q is placed at the centre of the sphere. Find the time period of motion of the particle?

View Solution
