A nonconducting disk of radius a and uniform positive surface charge density σ is placed on the ground, with its axis vertical. A particle of mass m and positive charge q is dropped, along the axis of the disk, from a height H with zero initial velocity. The particle has q/m=4ε0g/σ.
(i) Find the value of H if the particle just reaches the disk.
(ii) Sketch the potential energy of the particle as a function of its height and find its equilibrium position.
(i) Given that a is the radius of disk, σ is the surface charge density and q/m=4ε0g/σ. The kinetic energy of the particle, when it reaches the disk, can be taken as zero. Potential due to a charged disk at any axial point situated at a distance x from O is
(##BMS_V03_C03_S01_047_S01##).
V(x)=σ2ε0[√a2+x2−x]
Hence,
V(H)=σ2ε0[√a2+H2−H]
and V(O)=σa2ε0
According to the law of conservation of energy, we have the loss of graviatation potential energy is equal to the gain in electric potential energy.
mgH=qΔV=q[V(0)−V(H)]
=q[a−{√(a2+H2)−H}]σ2ε0 ....(i)
we are given
σ2ε0=2mg
Putting this in Eq. (i), we get
mgH=2mg[a−{√(a2+H2)−H}]
or H=2[a+H−√(a2+H2)]
=2a+2H−2√(a2+H2)
or 2√(a2+H2)=H+2a
or 4a2+4H2=H2+4a2+4aH
or 3H2=4aH
or H=4a3 (Since H = 0 is not valid)
(ii) The total potential energy of the particle at height x is
U(x)=mgx+qV(x)=mgx+qσ2ε0(√a2+x2−x)
=mgx+2mg+[√(a2+x2)−x]
=mg[2√(a2+x2)−x] ...(ii)
For equilibrium
dUdx=0
This gives x=a/√3 From Eq. (ii) graph between U(x) and x can be plotted as shown in (Fig. 3.99).
 .
.
Topper's Solved these Questions
- ELECTRIC POTENTIALBook:CENGAGE PHYSICSChapter:ELECTRIC POTENTIALExercise:Exercise 3.1
Explore 23 Videos
- ELECTRIC POTENTIALBook:CENGAGE PHYSICSChapter:ELECTRIC POTENTIALExercise:Exercise 3.2
Explore 9 Videos
- ELECTRIC POTENTIALBook:CENGAGE PHYSICSChapter:ELECTRIC POTENTIALExercise:DPP 3.5
Explore 14 Videos
- ELECTRIC FLUX AND GAUSS LAWBook:CENGAGE PHYSICSChapter:ELECTRIC FLUX AND GAUSS LAWExercise:MCQ s
Explore 38 Videos
- ELECTRICAL MEASURING INSTRUMENTSBook:CENGAGE PHYSICSChapter:ELECTRICAL MEASURING INSTRUMENTSExercise:M.C.Q
Explore 2 Videos
Similar Questions
A dielectric disc of radius R and uniform positive surface charge density σ is placed on the grup with its axis vertical. A particle of mass m and positive charge q is dropped, along the axis of the disc from a height H with dropped, along the axis of the disc from a height H with zero intial velocity. The charge -mass ratio of the particle is qm=4εon0gσ.
(i). Find the value of H if the particle just reaches the disc.
(ii). Sketch the potential energy of the particle as a function of its height and find its height in equilibrium position.
View SolutionA uniformly charged disc of radius R having surface charge density σ is placed in the xy plane with its center at the origin. Find the electric field intensity along the z-axis at a distance Z from origin :
View Solution
Knowledge Check
A uniform cube of mass m and edge a moves on a horizontal surface along the positive x-axis, with initial velocity v0. Then
ADuring motion , NgtmgBDuring motion, normal reactions acts on the center of massCduring motion, the normal reaction shifts towards positive x-axis from the center of massDduring motion, normal reaction shifts in the direction of the force of friction.A long solenoid carrying a current I is placed with its axis vertical as shown in the figure. A particle of mass m and charge q is released from the top of the solenoid. Its acceleration is (g being acceleration due to gravity)
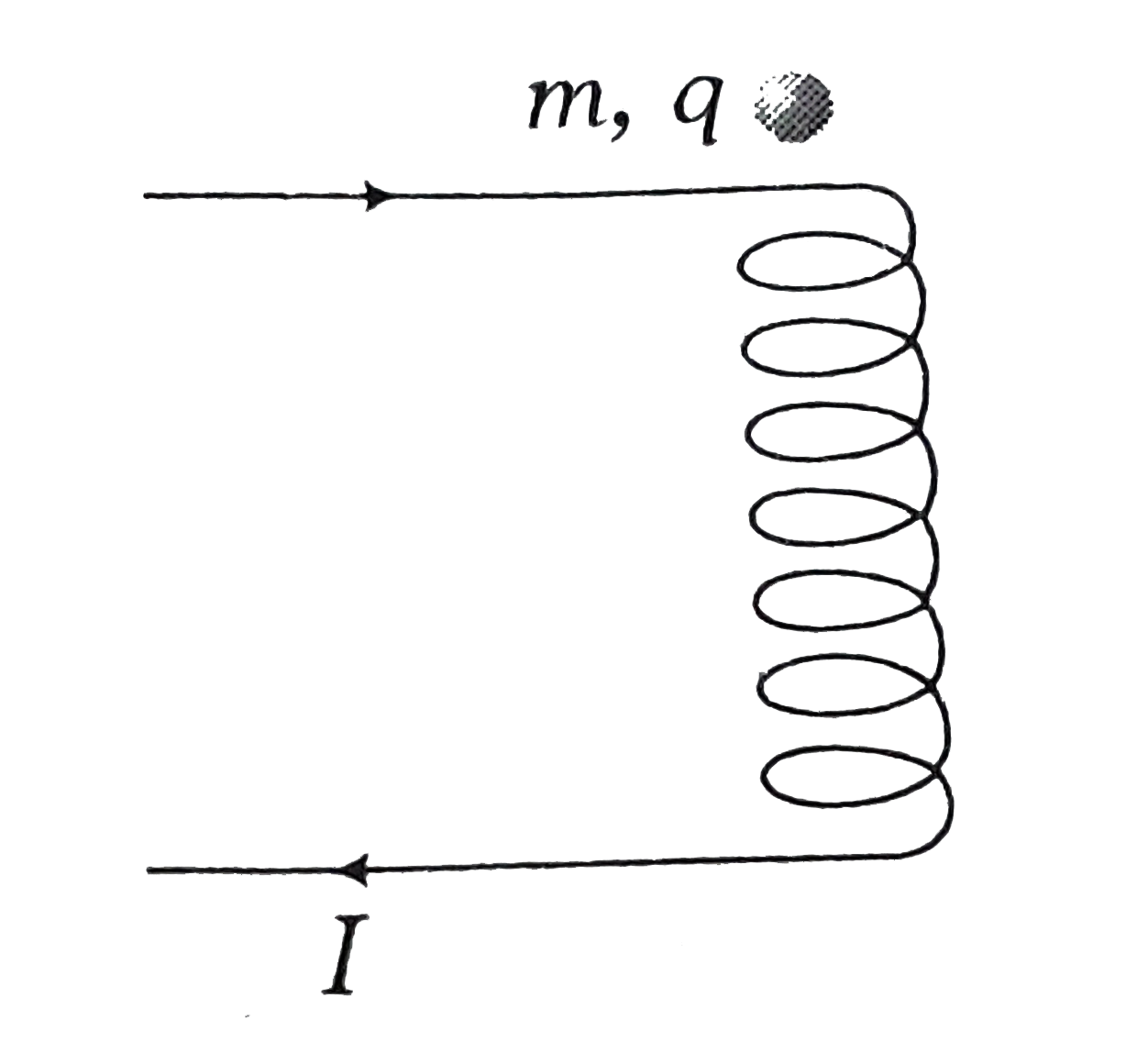 Agreater than gBless than gCequal to gDNone of these
Agreater than gBless than gCequal to gDNone of theseA thin circular disk of radius R is uniformly charged with density σ>0 per unit area.The disk rotates about its axis with a uniform angular speed ω.The magnetic moment of the disk is :
AπR4σωBπR42σωCπR44σωD2πR4σω
Charged Disk
View SolutionA circular ring of radius R with uniform positive charge density λ per unit length is located in the y-z plane with its centre at the origin O. A particle of mass m and positive charge q is projected from the point P (R√3,0,0) on the positive x-axis directly towards O, with an initial speed v. Find the smallest (non-zero) value of the speed v such that the particle does not return to P.
View SolutionA smooth chute is made in a dielectric sphere of radius R and uniform volume charge density. ρ. A charge particle of mass m and charge -q is placed at the centre of the sphere. Find the time period of motion of the particle?

View SolutionAn infinte wire having linear charge density λ is arranged as shown in fig. A charge particle of mass m and charge q is released charged q is released from point P. Find the initial acceleration of the particle just after the particle is released.
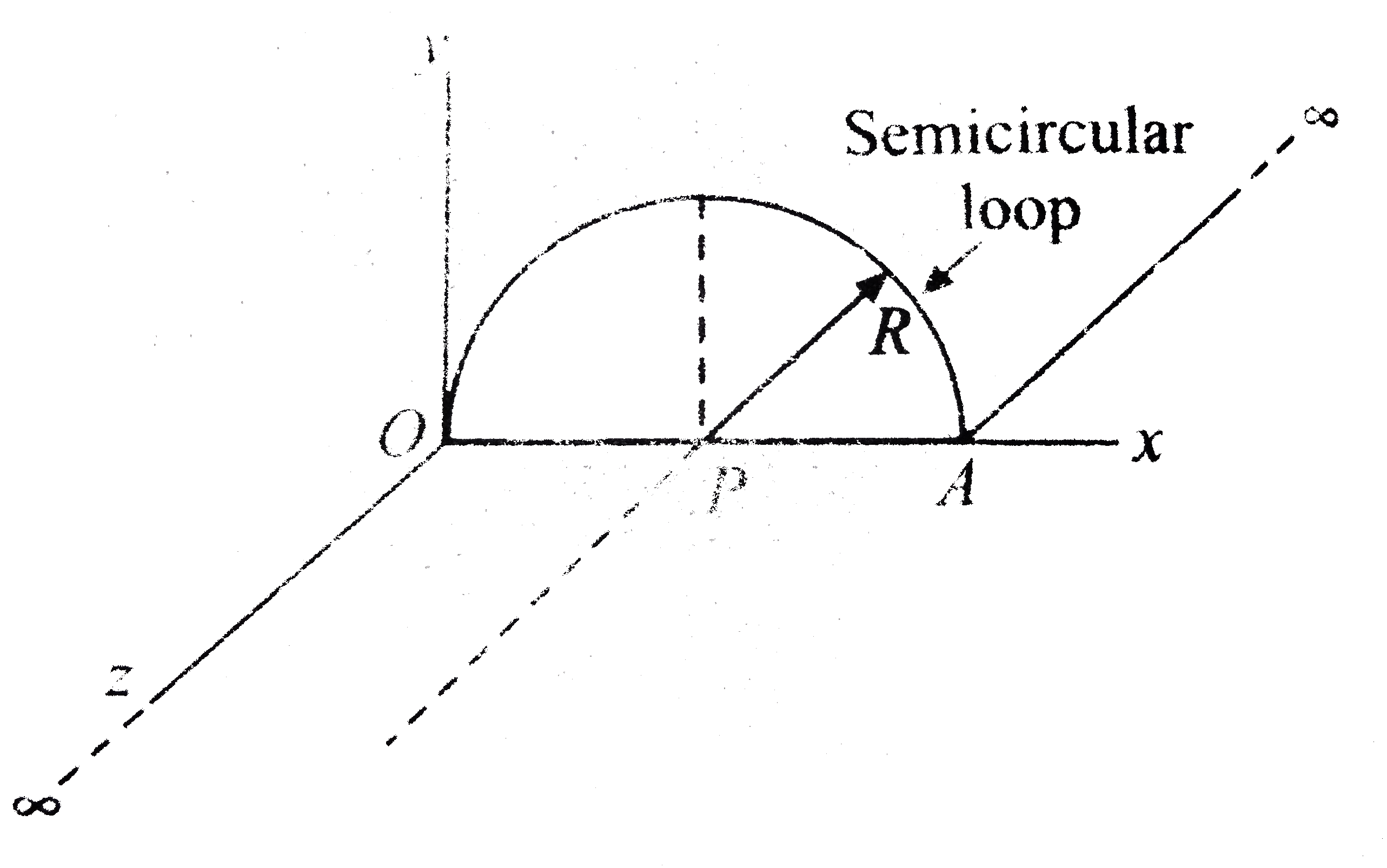
View SolutionA circular ring of radius R with uniform positive charge density λ per unit length is located in the y z plane with its center at the origin O. A particle of mass m and positive charge q is projected from that point p(−√3R,0,0) on the negative x - axis directly toward O, with initial speed V. Find the smallest (nonzero) value of the speed such that the particle does not return to P ?
View Solution