Question

A non-conducting disc of radius a with uniform positive surface charge density $\sigma$ is placed on the ground with its axis vertical. A particle of mass m and positive charge q is dropped along the axis of the disc from a height H with zero initial velocity. Charge per unit mass of the particle is $\frac{q}{m}=\frac{4{\epsilon }_{0}g}{\sigma }$.
(i) Find the value of H if the particle just reaches the disc.

Explanation

Let us assume that the amount of charge in the ring of thickness dr is dq.
Potential at P due to this ring,
`dV = 1/(4 pi epsilon_0) (dq)/(x)`, where `x = sqrt(H^(2)+r^(2))`
`:. dV = 1/(4 pi epsilon_0) ((2pi r dr)sigma)/(sqrt(H^(2)+r^(2))) [ :' dq = (2 pi r dr)sigma]`
`=(sigma)/(2epsilon_0) (rdr)/(sqrt(H^(2)+r^(2)))`

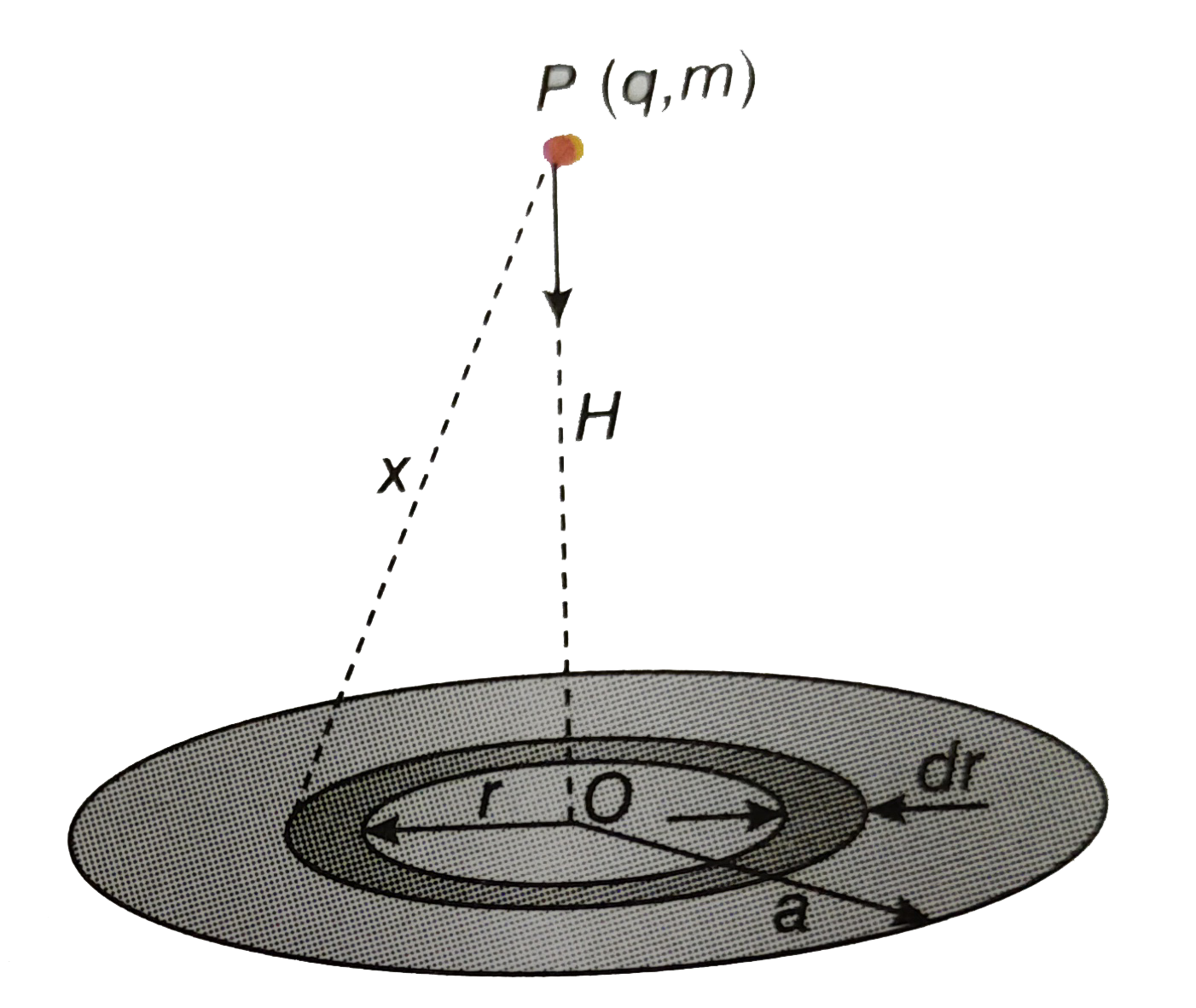
Now, potential at P due to the complete disc,
`V_(p) = int_(r=0)^(r=a)dV=(sigma)/(2epsilon_0)int_(r=0)^(r=a) (rdr)/(sqrt(H^(2)+r^(2)))`
`:. V_(P) = (sigma)/(2epsilon_0)[sqrt(a^(2)+H^(2))-H]`
So potential at the centre `(O) , V_(O) = (sigma a)/(2 epsilon_0) [ :' H = 0 ]`
(i) Particle is released from P and it just reaches O.
Since initial kinetic energy = final kinetic energy = 0
Hence increase in kinetic energy = 0
From conservation of mechanical energy we may write, decrease in gravitational potential energy = increase in electrostatic potential energy .
`:. mgH = q[V_(O) - V_(P)]`
or, `mgH = (q) ((sigma)/(2 epsilon_0))[a -sqrt(a^(2)+H^(2))+H]`
`gH = (q)/(m) ((sigma)/(2 epsilon_0))[a -sqrt(a^(2)+H^(2))+H]` ...........(1)
Given , `q/m = (4 epsilon_(0)g)/(simga) or, (qsigma)/(2 epsilon_(0)m) = 2g`
Substituting this value in equation (1), we get
`gH = 2g[a + H - sqrt(a^(2)+H^(2))]`
or, `H/2 = (a+H) - sqrt(a^(2)+H^(2))`
or, `a^(2) + H^(2) = a^(2) + (H^2)/4 + aH or, 3/4 H^(2) = aH`
`:. H = 4/3 a [ :' H != 0]`