Find the centre of mass of a uniform semicircular ring of radius R and mass M.
To find the center of mass of a uniform semicircular ring of radius R and mass M, we can follow these steps:
Step 1: Define the Geometry
Consider a semicircular ring with radius R. The center of the semicircle is at the origin (0, 0) in a Cartesian coordinate system. The semicircular ring extends from −R to R along the x-axis and is symmetric about the y-axis.
Step 2: Set Up the Differential Mass Element
Since the ring is uniform, we can define the mass per unit length λ:
λ=ML
where L is the length of the semicircular ring. The length L of a semicircle is given by:
L=πR
Thus, we have:
λ=MπR
Now, we can express a differential mass element dm in terms of an angle θ:
dm=λdL
The differential length dL for a small angle dθ is:
dL=Rdθ
So, substituting for dm:
dm=λRdθ=MπRRdθ=Mπdθ
Step 3: Determine the Coordinates of the Center of Mass
The coordinates of the center of mass (xcm,ycm) can be found using the following formulas:
xcm=1M∫xdm
ycm=1M∫ydm
For the semicircular ring, the coordinates (x,y) in terms of θ are:
x=Rcosθ
y=Rsinθ
Step 4: Calculate xcm
Since the semicircular ring is symmetric about the y-axis, the x-coordinate of the center of mass will be zero:
xcm=1M∫π0(Rcosθ)dm=0
Step 5: Calculate ycm
Now, we calculate ycm:
ycm=1M∫π0(Rsinθ)dm
Substituting dm:
ycm=1M∫π0(Rsinθ)(Mπdθ)
=Rπ∫π0sinθdθ
Step 6: Evaluate the Integral
The integral of sinθ from 0 to π is:
∫π0sinθdθ=(−cosθ)π0=−cos(π)−(−cos(0))=1+1=2
Thus:
ycm=Rπ⋅2=2Rπ
Step 7: Final Result
The center of mass of the uniform semicircular ring is located at:
(0,2Rπ)
Consider a semicircular ring with radius R. The center of the semicircle is at the origin (0, 0) in a Cartesian coordinate system. The semicircular ring extends from −R to R along the x-axis and is symmetric about the y-axis.
Since the ring is uniform, we can define the mass per unit length λ:
λ=ML
where L is the length of the semicircular ring. The length L of a semicircle is given by:
L=πR
Thus, we have:
λ=MπR
dm=λdL
The differential length dL for a small angle dθ is:
dL=Rdθ
So, substituting for dm:
dm=λRdθ=MπRRdθ=Mπdθ
The coordinates of the center of mass (xcm,ycm) can be found using the following formulas:
xcm=1M∫xdm
ycm=1M∫ydm
x=Rcosθ
y=Rsinθ
Since the semicircular ring is symmetric about the y-axis, the x-coordinate of the center of mass will be zero:
xcm=1M∫π0(Rcosθ)dm=0
Now, we calculate ycm:
ycm=1M∫π0(Rsinθ)dm
Substituting dm:
ycm=1M∫π0(Rsinθ)(Mπdθ)
=Rπ∫π0sinθdθ
The integral of sinθ from 0 to π is:
∫π0sinθdθ=(−cosθ)π0=−cos(π)−(−cos(0))=1+1=2
Thus:
ycm=Rπ⋅2=2Rπ
The center of mass of the uniform semicircular ring is located at:
(0,2Rπ)
Topper's Solved these Questions
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - NCERT
21 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - ADDITIONAL EXERCISES
11 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - SOLVED EXAMPLES TYPE E
1 Video
- RAY OPTICSBOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - Problem For Practice(a)
25 Videos
- THERMODYNAMICSBOOK - PRADEEPCHAPTER - THERMODYNAMICSEXERCISE - Assertion- Reason Type Questions
19 Videos
Similar Questions
Knowledge Check
A particle of mass m is placed on centre of curvature of a fixed, uniform semi-circular ring of radius R and mass M as shown in figure. Calculate:
(a) interaction force between the ring and the particle and
(b) work required to displace the particle from centre of curvature to infinity.
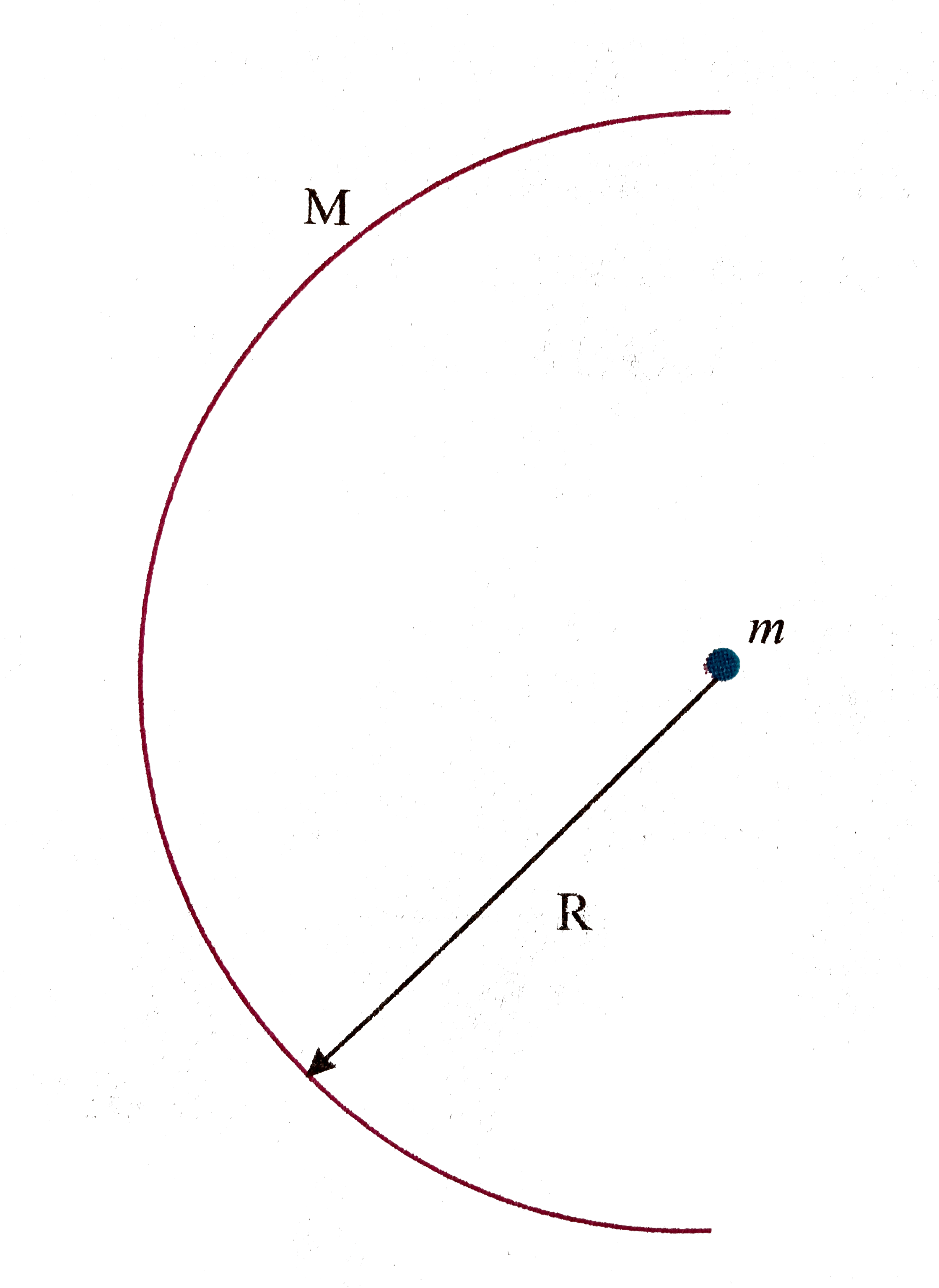 A(a)F=2GMπR2,(b)GMRB(a)F=2GMmπ2R,(b)GMmR2C(a)F=−2GMmπR2(b)−GMmRD(a)F=2GMmπR2(b)GMmR
A(a)F=2GMπR2,(b)GMRB(a)F=2GMmπ2R,(b)GMmR2C(a)F=−2GMmπR2(b)−GMmRD(a)F=2GMmπR2(b)GMmRConsider following statements
(1) CM of a uniform semicircular disc of radius R is 2R/π from the centre
(2) CM of a uniform semicircular ring of radius R is 4R/3π from the centre
(3) CM of a solid hemisphere of radius R is 4R/3π from the centre
(4) CM of a hemisphere shell of radius R is R/2 from the centre
Which statements are correct?A1, 2, 4B1, 3, 4C4 onlyD1, 2 only
Centre Of Mass Of Uniform Semi Circular Ring
View SolutionFind the centre of mass of a homogeneous semicircular plate of radius a.
View SolutionDetermine the centre of mass of a uniform hemisphere of radius R.
View SolutionLocate c.m. of thin , uniform semicircular wire of radius R.
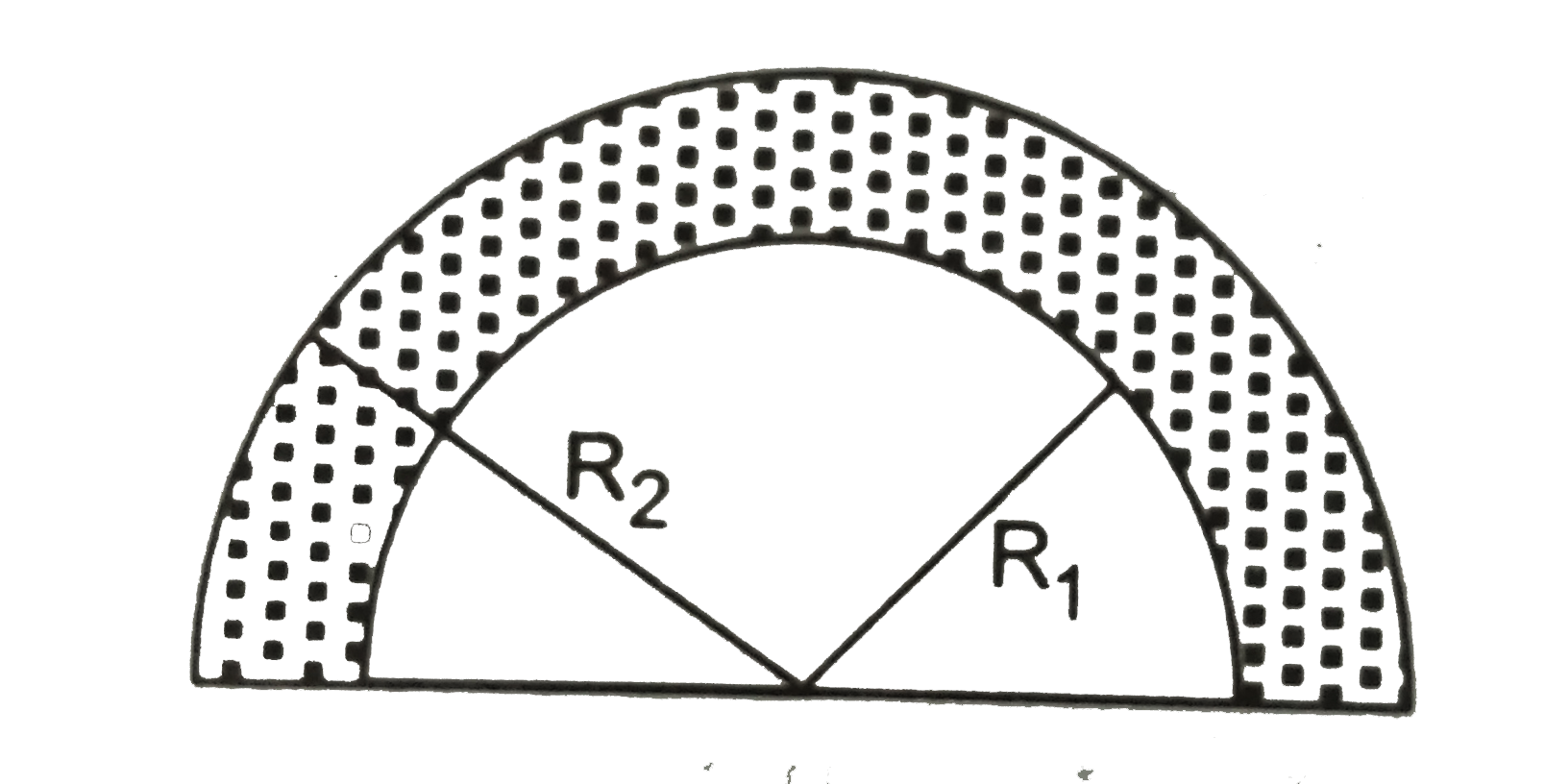
View SolutionFind the centre of mass of a uniform plate having semicircular inner and outer boundaries of radii R1andR2.
View SolutionFind the electric field at the centre of a uniformly charged semicircular ring of radius R. Linear charge density is λ
View Solution
