The density of a linear rod of length L varies as $ρ = A + B x$ where x is the distance from the left end. Locate the centre of mass.

Video Solution

Text Solution

Verified by Experts

Here, variation of density is given by
$ρ = A + B x$
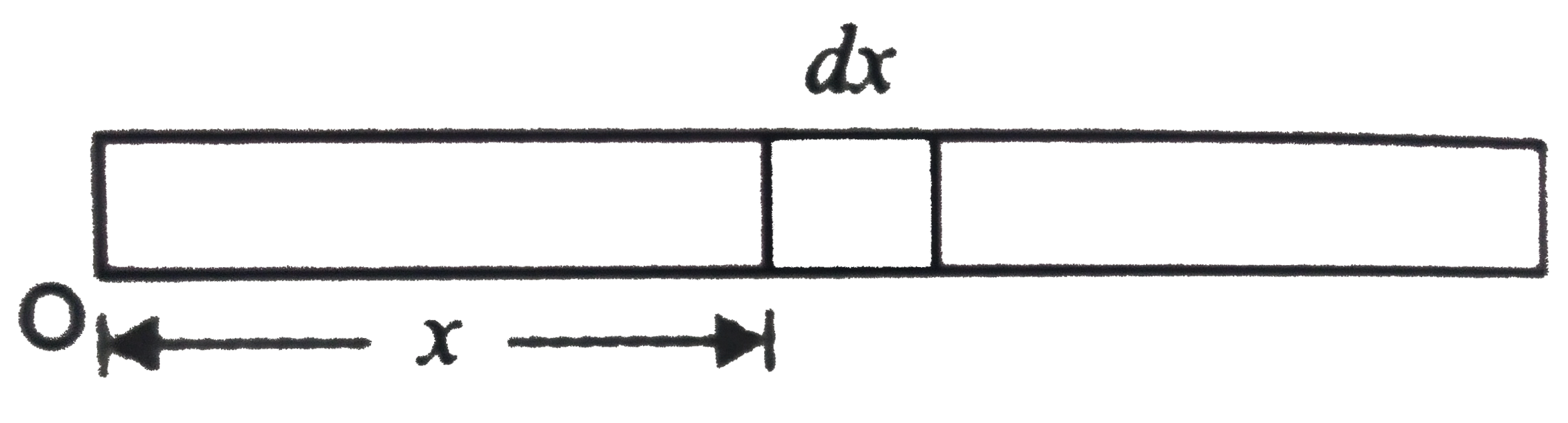
Let $a$ be the area of cross-section of the linear rod. Consider a small element of the rod, of length $d x$ , Fig at a distance $x$ from the left end.
Volume of the element $= a d x$
mass of element, $d m = (a d x) ρ = (A + B x) a d x$
The x-coordinate of the centre of mass is
$x_{c m} = \frac{\int_{0}^{L} x d m}{\int_{0}^{L} d m} = \frac{\int_{0}^{L} x (A + B x) a d x}{\int_{0}^{L} (A + B x) a d x}$
$= \frac{{[A \frac{x^{2}}{2} + B \frac{x^{3}}{3}]}_{0}^{L}}{{[A x + \frac{B x^{2}}{2}]}_{0}^{L}} = \frac{[\frac{A L^{2}}{2} + \frac{B L^{3}}{2}]}{[A L + \frac{B L^{2}}{2}]}$
$x_{c m} = \frac{3 A L + 2 B L^{2}}{3 (2 A + B L)}$

Updated on:21/07/2023

If the linear density (mass per unit length) of a rod of length $3 m$ is proportional to $x$ , where $x$ , where $x$ is the distance from one end of the rod, the distance of the centre of gravity of the rod from this end is.
A2.5 m
B1 m
C1.5 m
D2 m
A rod of length L is of non uniform cross-section. Its mass per unit length varies linearly with distance from left end. Then its centre of mass from left end is at a distance. (Take linear density at left end=0).

A $\frac{L}{3}$
B $\frac{L}{6}$
C $\frac{L}{2}$
D $\frac{2 L}{3}$