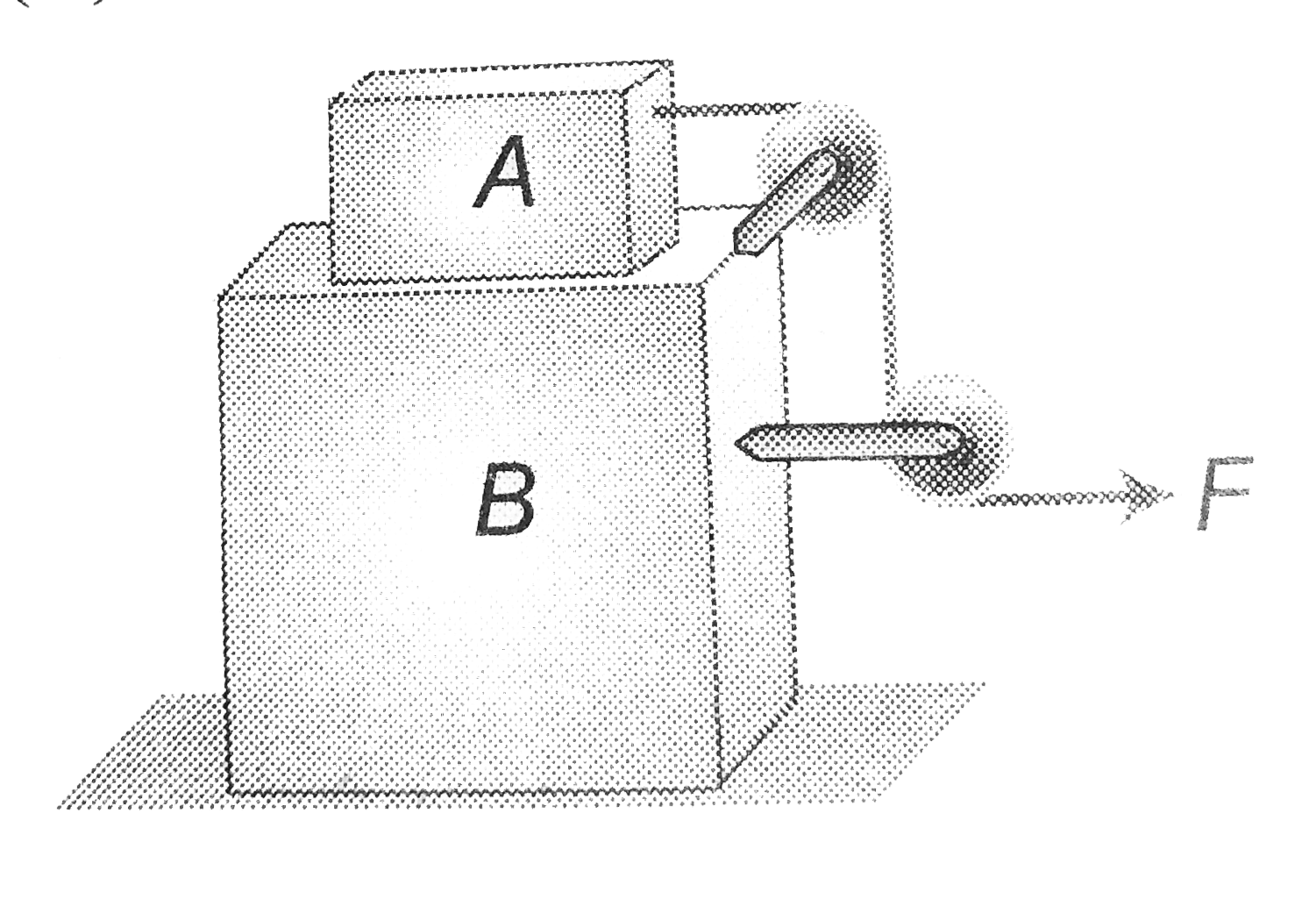
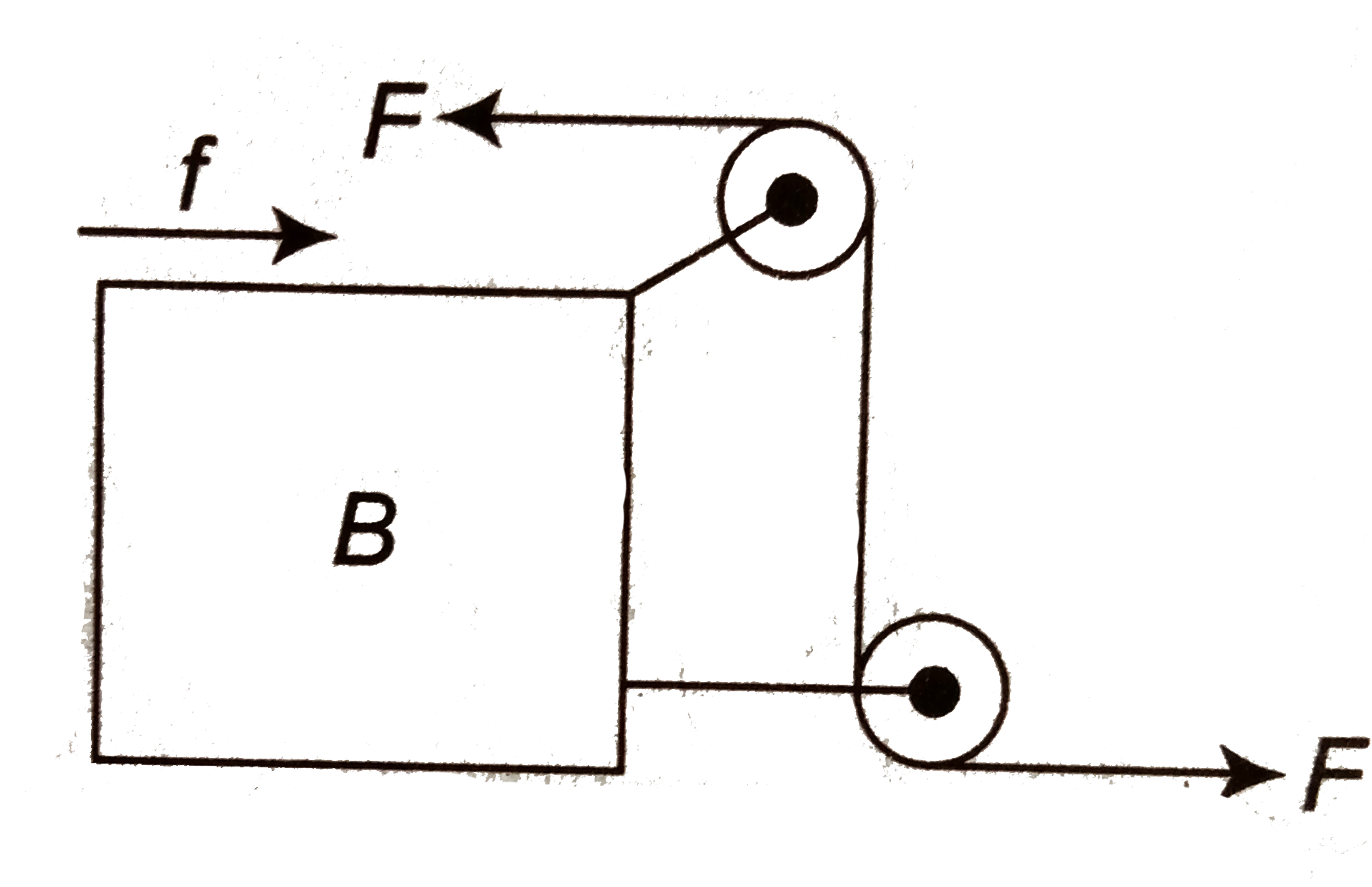
In the arrangement shown in figure, m_A = m_B = 2kg.
String is massless and pulley is frictionless. Block B is resting on a smooth horizontal surface, while friction coefficient beteen block A and B is . the Maximum horizontal force F that can be applied so that block A does not slip over block B is.
The correct Answer is:D
Maximum value of friction on ,
Hence, the block is subject to this frictional force in the forward direction. Since the block does not slip on , both of them move with a common acceleration. The acceleration of is the same as the acceleration of .
To determmine acceleration of , we find that
. 
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBook:A2ZChapter:NEWTONS LAWS OF MOTIONExercise:Assertion Reasoning
Explore 14 Videos
- NEWTONS LAWS OF MOTIONBook:A2ZChapter:NEWTONS LAWS OF MOTIONExercise:NEET Questions
Explore 38 Videos
- NEWTONS LAWS OF MOTIONBook:A2ZChapter:NEWTONS LAWS OF MOTIONExercise:Dynamics Of Circular Motion
Explore 31 Videos
- MOTION IN TWO DIMENSIONBook:A2ZChapter:MOTION IN TWO DIMENSIONExercise:Chapter Test
Explore 29 Videos
- OSCILLATION AND SIMPLE HARMONIC MOTIONBook:A2ZChapter:OSCILLATION AND SIMPLE HARMONIC MOTIONExercise:Chapter Test
Explore 29 Videos
Similar Questions
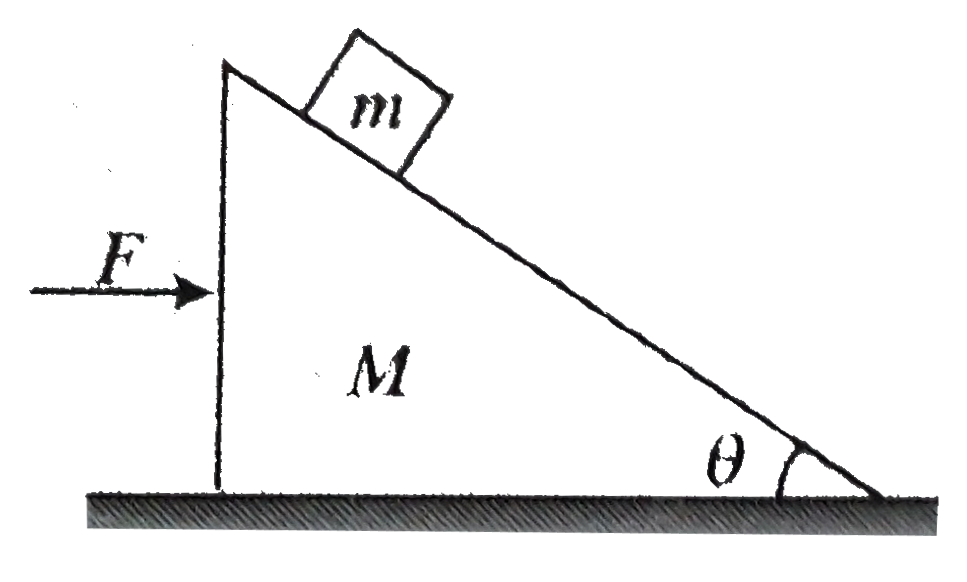
A bar of mass m is placed on a triangular block of mass M as shown in figure The friction coefficient between the two surface is μ and ground is smooth find the minimum and maximum horizontal force F required to be applied on block so that the bar will not slip on the inclined surface of block
View SolutionTwo block "A&B" of mass MA=1kg&MB=3kg are kept on a smooth table as shown in the figure.The coefficient of friction between "A&B" is "0.2" .The maximum force "F" that can be applied on "B" horizontally,so that the block "A" do not slide over the block "B" is Take g=10ms2andk=400Nm)
View Solution
Knowledge Check
In the arrangement shown in figure mA=mB=2kg. String is massless and pulley is frictionless. Block B is resting on a smooth horizontal surface, while friction coefficient between blocks A and B is μ=0.5. What maximum horizontal force F can be applied so that block A does not slip over the block B? (g=10m/s2)
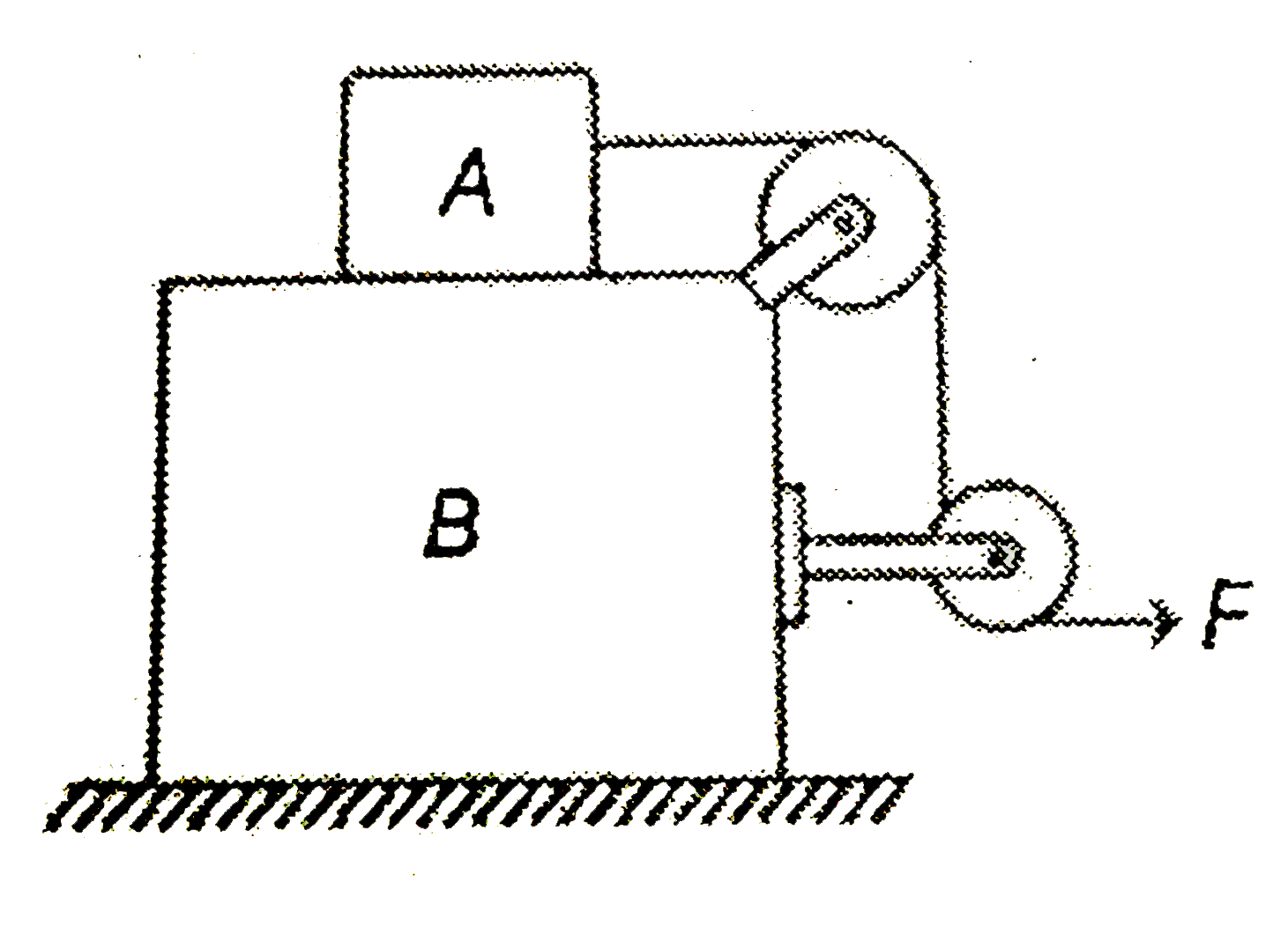 A25 NB40 NC30 ND20 N
A25 NB40 NC30 ND20 NTwo block A and B of masses 5 kg. and 3 kg. respectively rest on smooth horizontal surface with B over A. The coefficient of friction between A and B. 0.5 the maximum horizontal force that can be applied to A so that there will be motion of A and B without separation is :-
A15B25C40D50A block is kept on a rough horizontal surface as shown. Its mass is 2 kg and coefficient of friction between block and surface (μ)=0.5. A horizontal force F is acting on the block. When
 AF = 4 N, acceleration is zero.BF = 4 N, friction is 10 N and acceleration is 3m/s2.CF = 14 N, acceleration is 2m/s2.DF = 14 N, friction is 14 N.
AF = 4 N, acceleration is zero.BF = 4 N, friction is 10 N and acceleration is 3m/s2.CF = 14 N, acceleration is 2m/s2.DF = 14 N, friction is 14 N.
A block of mass m is placed on another block of mass M lying on a smooth horizontal surface as shown in the figure. The co-efficient of friction between the blocks is μ. Find the maximum horizontal force F (in Newton) that can be applied to the block M so that the blocks together with same acceleration? (M=3kg,m=2kg,μ=0.1,g=10m/s2)

View SolutionBlock B rests on a smooth surface .The coefficient of static friction between A and B is μ=0.4 where F=30N then
Acceleration of lower block is

View SolutionIn the arrangement shown in figure the wall is smooth and friction coefficient between the blocks is μ=0.1. A horizontal force F=1000N is applied on the 2kg block. The wrong statement is :

View Solution
Two blocks A and B are shown in figure. The minimum horizontal force F applied on Blocks B for which slipping begins at B and ground is
View SolutionBlock B rests on a smooth surface .The coefficient of static friction between A and B is μ=0.4 where F=30N then
Acceleration of upper block is
View Solution